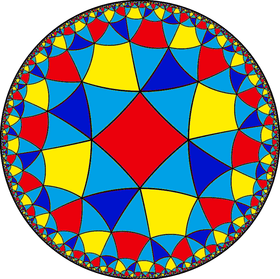
Cantic order-4 hexagonal tiling
In geometry, the cantic order-4 hexagonal tiling is a uniform tiling of the hyperbolic plane. It has Schläfli symbol of t0,1{(4,4,3)} or h2{6,4}.
| Cantic order-4 hexagonal tiling | |
|---|---|
 Poincaré disk model of the hyperbolic plane | |
| Type | Hyperbolic uniform tiling |
| Vertex configuration | 3.8.4.8 |
| Schläfli symbol | t0,1(4,4,3) |
| Wythoff symbol | 4 4 | 3 |
| Coxeter diagram |    |
| Symmetry group | [(4,4,3)], (*443) |
| Dual | Order-4-4-3 t01 dual tiling |
| Properties | Vertex-transitive |
Related polyhedra and tiling
| Uniform (4,4,3) tilings | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Symmetry: [(4,4,3)] (*443) | [(4,4,3)]+ (443) |
[(4,4,3+)] (3*22) |
[(4,1+,4,3)] (*3232) | |||||||
 |
|
 |
 |
|
 |
|
  |
|
|
|
  |
|
 |
|
|
|
|
|
|
|
 |
 |
|
 |
 |
 |
 |
 |
 |
 |
 |
 |
| h{6,4} t0(4,4,3) |
h2{6,4} t0,1(4,4,3) |
{4,6}1/2 t1(4,4,3) |
h2{6,4} t1,2(4,4,3) |
h{6,4} t2(4,4,3) |
r{6,4}1/2 t0,2(4,4,3) |
t{4,6}1/2 t0,1,2(4,4,3) |
s{4,6}1/2 s(4,4,3) |
hr{4,6}1/2 hr(4,3,4) |
h{4,6}1/2 h(4,3,4) |
q{4,6} h1(4,3,4) |
| Uniform duals | ||||||||||
 |
 |
 |
 |
|||||||
| V(3.4)4 | V3.8.4.8 | V(4.4)3 | V3.8.4.8 | V(3.4)4 | V4.6.4.6 | V6.8.8 | V3.3.3.4.3.4 | V(4.4.3)2 | V66 | V4.3.4.6.6 |
gollark: Hypothetically you could have a cryptocurrency where only the government can issue a coin - instead of mining it (proof of work), it would just be digitally signed by a government key.
gollark: They totally can.
gollark: Having one organization perform an increasingly large amount of important functions never ends well.
gollark: Payments are hard and Bitcoin and other cryptocurrencies, despite being generally kind of terrible, at least have a solution which is *technologically* secured instead of just relying on goodwill or something, and which doesn't force you into one central provider.
gollark: That's a good thing. Having your payment provider *also* keep your money is a problem.
References
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 19, The Hyperbolic Archimedean Tessellations)
- "Chapter 10: Regular honeycombs in hyperbolic space". The Beauty of Geometry: Twelve Essays. Dover Publications. 1999. ISBN 0-486-40919-8. LCCN 99035678.
See also
 |
Wikimedia Commons has media related to Uniform tiling 3-8-4-8. |
- Square tiling
- Uniform tilings in hyperbolic plane
- List of regular polytopes
External links
- Weisstein, Eric W. "Hyperbolic tiling". MathWorld.
- Weisstein, Eric W. "Poincaré hyperbolic disk". MathWorld.
- Hyperbolic and Spherical Tiling Gallery
- KaleidoTile 3: Educational software to create spherical, planar and hyperbolic tilings
- Hyperbolic Planar Tessellations, Don Hatch
  | ||||||
| ||||||
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.