Apeirogonal antiprism
In geometry, an apeirogonal antiprism or infinite antiprism[1] is the arithmetic limit of the family of antiprisms; it can be considered an infinite polyhedron or a tiling of the plane.
| Apeirogonal antiprism | |
|---|---|
 | |
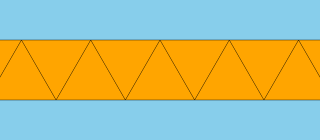| Type | Semiregular tiling |
| Vertex configuration | 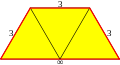 3.3.3.∞ |
| Schläfli symbol | sr{2,∞} or |
| Wythoff symbol | | 2 2 ∞ |
| Coxeter diagram |     |
| Symmetry | [∞,2+], (∞22) |
| Rotation symmetry | [∞,2]+, (∞22) |
| Bowers acronym | Azap |
| Dual | Apeirogonal deltohedron |
| Properties | Vertex-transitive |
If the sides are equilateral triangles, it is a uniform tiling. In general, it can have two sets of alternating congruent isosceles triangles, surrounded by two half-planes.
Related tilings and polyhedra
The apeirogonal antiprism is the arithmetic limit of the family of antiprisms sr{2, p} or p.3.3.3, as p tends to infinity, thereby turning the antiprism into a Euclidean tiling.
The apeirogonal antiprism can be constructed by applying an alternation operation to an apeirogonal prism.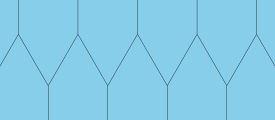
The dual tiling of an apeirogonal antiprism is an apeirogonal deltohedron.
Similarly to the uniform polyhedra and the uniform tilings, eight uniform tilings may be based from the regular apeirogonal tiling. The rectified and cantellated forms are duplicated, and as two times infinity is also infinity, the truncated and omnitruncated forms are also duplicated, therefore reducing the number of unique forms to four: the apeirogonal tiling, the apeirogonal hosohedron, the apeirogonal prism, and the apeirogonal antiprism.
| (∞ 2 2) | Parent | Truncated | Rectified | Bitruncated | Birectified (dual) |
Cantellated | Omnitruncated (Cantitruncated) |
Snub |
|---|---|---|---|---|---|---|---|---|
| Wythoff | 2 | ∞ 2 | 2 2 | ∞ | 2 | ∞ 2 | 2 ∞ | 2 | ∞ | 2 2 | ∞ 2 | 2 | ∞ 2 2 | | | ∞ 2 2 |
| Schläfli | {∞,2} | t{∞,2} | r{∞,2} | t{2,∞} | {2,∞} | rr{∞,2} | tr{∞,2} | sr{∞,2} |
| Coxeter |  |
|
|
|
|
|
|
|
| Image Vertex figure |
 {∞,2} |
∞.∞ |
∞.∞ |
4.4.∞ |
 {2,∞} |
4.4.∞ |
 4.4.∞ |
3.3.3.∞ |
Notes
- Conway (2008), p. 263
References
- The Symmetries of Things 2008, John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, ISBN 978-1-56881-220-5
- Grünbaum, Branko; Shephard, G. C. (1987). Tilings and Patterns. W. H. Freeman and Company. ISBN 0-7167-1193-1.
- T. Gosset: On the Regular and Semi-Regular Figures in Space of n Dimensions, Messenger of Mathematics, Macmillan, 1900
  | ||||||
| ||||||