5-cube
In five-dimensional geometry, a 5-cube is a name for a five-dimensional hypercube with 32 vertices, 80 edges, 80 square faces, 40 cubic cells, and 10 tesseract 4-faces.
| 5-cube penteract (pent) | ||
|---|---|---|
| Type | uniform 5-polytope | |
| Schläfli symbol | {4,3,3,3} {4,3,3}×{ } {4,3}×{4} {4,3}×{ }2 {4}×{4}×{ } {4}×{ }3 { }5 | |
| Coxeter diagram | ||
| 4-faces | 10 | tesseracts |
| Cells | 40 | cubes |
| Faces | 80 | squares |
| Edges | 80 | |
| Vertices | 32 | |
| Vertex figure | 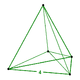 5-cell | |
| Coxeter group | B5, [4,33], order 3840 [4,3,3,2], order 768 [4,3,2,4], order 384 [4,3,2,2], order 192 [4,2,4,2], order 128 [4,2,2,2], order 64 [2,2,2,2], order 32 | |
| Dual | 5-orthoplex | |
| Base point | (1,1,1,1,1,1) | |
| Circumradius | sqrt(5)/2 = 1.118034 | |
| Properties | convex, isogonal regular | |
It is represented by Schläfli symbol {4,3,3,3} or {4,33}, constructed as 3 tesseracts, {4,3,3}, around each cubic ridge. It can be called a penteract, a portmanteau of tesseract (the 4-cube) and pente for five (dimensions) in Greek. It can also be called a regular deca-5-tope or decateron, being a 5-dimensional polytope constructed from 10 regular facets.
Related polytopes
It is a part of an infinite hypercube family. The dual of a 5-cube is the 5-orthoplex, of the infinite family of orthoplexes.
Applying an alternation operation, deleting alternating vertices of the 5-cube, creates another uniform 5-polytope, called a 5-demicube, which is also part of an infinite family called the demihypercubes.
The 5-cube can be seen as an order-3 tesseractic honeycomb on a 4-sphere. It is related to the Euclidean 4-space (order-4) tesseractic honeycomb and paracompact hyperbolic honeycomb order-5 tesseractic honeycomb.
As a configuration
This configuration matrix represents the 5-cube. The rows and columns correspond to vertices, edges, faces, cells, and 4-faces. The diagonal numbers say how many of each element occur in the whole 5-cube. The nondiagonal numbers say how many of the column's element occur in or at the row's element.[1][2]
Cartesian coordinates
The Cartesian coordinates of the vertices of a 5-cube centered at the origin and having edge length 2 are
- (±1,±1,±1,±1,±1),
while this 5-cube's interior consists of all points (x0, x1, x2, x3, x4) with -1 < xi < 1 for all i.
Images
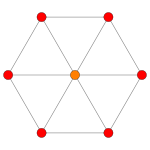
n-cube Coxeter plane projections in the Bk Coxeter groups project into k-cube graphs, with power of two vertices overlapping in the projective graphs.
| Coxeter plane | B5 | B4 / D5 | B3 / D4 / A2 |
|---|---|---|---|
| Graph |  |
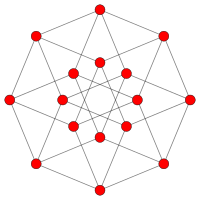 |
 |
| Dihedral symmetry | [10] | [8] | [6] |
| Coxeter plane | Other | B2 | A3 |
| Graph | 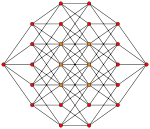 |
 |
 |
| Dihedral symmetry | [2] | [4] | [4] |
 Wireframe skew direction |
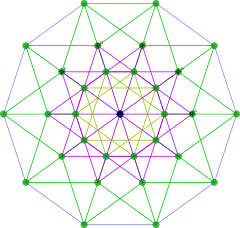 B5 Coxeter plane |
 Vertex-edge graph. |
 A perspective projection 3D to 2D of stereographic projection 4D to 3D of Schlegel diagram 5D to 4D. |
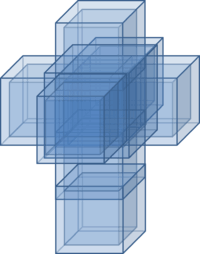 4D net of the 5-cube, perspective projected into 3D. |
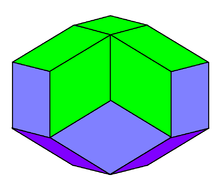
Projection
The 5-cube can be projected down to 3 dimensions with a rhombic icosahedron envelope. There are 22 exterior vertices, and 10 interior vertices. The 10 interior vertices have the convex hull of a pentagonal antiprism. The 80 edges project into 40 external edges and 40 internal ones. The 40 cubes project into golden rhombohedra which can be used to dissect the rhombic icosahedron. The projection vectors are u = {1, φ, 0, -1, φ}, v = {φ, 0, 1, φ, 0}, w = {0, 1, φ, 0, -1}, where φ is the golden ratio, .
| rhombic icosahedron | 5-cube | |
|---|---|---|
| Perspective | orthogonal | |
 |
 |
 |
Related polytopes
This polytope is one of 31 uniform 5-polytopes generated from the regular 5-cube or 5-orthoplex.
| B5 polytopes | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
 β5 |
 t1β5 |
 t2γ5 |
 t1γ5 |
 γ5 |
 t0,1β5 |
 t0,2β5 |
 t1,2β5 | ||||
 t0,3β5 |
 t1,3γ5 |
 t1,2γ5 |
 t0,4γ5 |
 t0,3γ5 |
 t0,2γ5 |
 t0,1γ5 |
 t0,1,2β5 | ||||
 t0,1,3β5 |
 t0,2,3β5 |
 t1,2,3γ5 |
 t0,1,4β5 |
 t0,2,4γ5 |
 t0,2,3γ5 |
 t0,1,4γ5 |
 t0,1,3γ5 | ||||
 t0,1,2γ5 |
 t0,1,2,3β5 |
 t0,1,2,4β5 |
 t0,1,3,4γ5 |
 t0,1,2,4γ5 |
 t0,1,2,3γ5 |
 t0,1,2,3,4γ5 | |||||
References
- Coxeter, Regular Polytopes, sec 1.8 Configurations
- Coxeter, Complex Regular Polytopes, p.117
- H.S.M. Coxeter:
- Coxeter, Regular Polytopes, (3rd edition, 1973), Dover edition, ISBN 0-486-61480-8, p. 296, Table I (iii): Regular Polytopes, three regular polytopes in n-dimensions (n≥5)
- Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6
- (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10]
- (Paper 23) H.S.M. Coxeter, Regular and Semi-Regular Polytopes II, [Math. Zeit. 188 (1985) 559-591]
- (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45]
- Norman Johnson Uniform Polytopes, Manuscript (1991)
- N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D. (1966)
- Klitzing, Richard. "5D uniform polytopes (polytera) o3o3o3o4x - pent".
External links
- Weisstein, Eric W. "Hypercube". MathWorld.
- Olshevsky, George. "Measure polytope". Glossary for Hyperspace. Archived from the original on 4 February 2007.
- Multi-dimensional Glossary: hypercube Garrett Jones
Fundamental convex regular and uniform polytopes in dimensions 2–10 | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Family | An | Bn | I2(p) / Dn | E6 / E7 / E8 / F4 / G2 | Hn | |||||||
| Regular polygon | Triangle | Square | p-gon | Hexagon | Pentagon | |||||||
| Uniform polyhedron | Tetrahedron | Octahedron • Cube | Demicube | Dodecahedron • Icosahedron | ||||||||
| Uniform 4-polytope | 5-cell | 16-cell • Tesseract | Demitesseract | 24-cell | 120-cell • 600-cell | |||||||
| Uniform 5-polytope | 5-simplex | 5-orthoplex • 5-cube | 5-demicube | |||||||||
| Uniform 6-polytope | 6-simplex | 6-orthoplex • 6-cube | 6-demicube | 122 • 221 | ||||||||
| Uniform 7-polytope | 7-simplex | 7-orthoplex • 7-cube | 7-demicube | 132 • 231 • 321 | ||||||||
| Uniform 8-polytope | 8-simplex | 8-orthoplex • 8-cube | 8-demicube | 142 • 241 • 421 | ||||||||
| Uniform 9-polytope | 9-simplex | 9-orthoplex • 9-cube | 9-demicube | |||||||||
| Uniform 10-polytope | 10-simplex | 10-orthoplex • 10-cube | 10-demicube | |||||||||
| Uniform n-polytope | n-simplex | n-orthoplex • n-cube | n-demicube | 1k2 • 2k1 • k21 | n-pentagonal polytope | |||||||
| Topics: Polytope families • Regular polytope • List of regular polytopes and compounds | ||||||||||||