n-sphere
In mathematics, an n-sphere is a topological space that is homeomorphic to a standard n-sphere, which is the set of points in (n + 1)-dimensional Euclidean space that are situated at a constant distance r from a fixed point, called the center. It is the generalization of an ordinary sphere in the ordinary three-dimensional space. The "radius" of a sphere is the constant distance of its points to the center. When the sphere has unit radius, it is usual to call it the unit n-sphere or simply the n-sphere for brevity. In terms of the standard norm, the n-sphere is defined as

and an n-sphere of radius r can be defined as
The 0-sphere is a pair of points on the line, the 1-sphere is a circle in the plane, and the 2-sphere is an ordinary sphere within 3-dimensional space.
The dimension of n-sphere is n, and must not be confused with the dimension (n + 1) of the Euclidean space in which it is naturally embedded. An n-sphere is the surface or boundary of an (n + 1)-dimensional ball.
In particular:
- the pair of points at the ends of a (one-dimensional) line segment is a 0-sphere,
- a circle, which is the one-dimensional circumference of a (two-dimensional) disk, is a 1-sphere,
- the two-dimensional surface of a (three-dimensional) ball in three-dimensional space is a 2-sphere, often simply called a sphere,
- the three-dimensional boundary of a (four-dimensional) 4-ball in four-dimensional Euclidean is a 3-sphere, also known as a glome.
- the n – 1 dimensional boundary of a (n-dimensional) n-ball is an (n – 1)-sphere.
For n ≥ 2, the n-spheres that are differential manifolds can be characterized (up to a diffeomorphism) as the simply connected n-dimensional manifolds of constant, positive curvature. The n-spheres admit several other topological descriptions: for example, they can be constructed by gluing two n-dimensional Euclidean spaces together, by identifying the boundary of an n-cube with a point, or (inductively) by forming the suspension of an (n − 1)-sphere. The 1-sphere is the 1-manifold that is a circle, which is not simply connected. The 0-sphere is the 0-manifold consisting of two points, which is not even connected.
Description
For any natural number n, an n-sphere of radius r is defined as the set of points in (n + 1)-dimensional Euclidean space that are at distance r from some fixed point c, where r may be any positive real number and where c may be any point in (n + 1)-dimensional space. In particular:
- a 0-sphere is a pair of points {c − r, c + r}, and is the boundary of a line segment (1-ball).
- a 1-sphere is a circle of radius r centered at c, and is the boundary of a disk (2-ball).
- a 2-sphere is an ordinary 2-dimensional sphere in 3-dimensional Euclidean space, and is the boundary of an ordinary ball (3-ball).
- a 3-sphere is a 3-dimensional sphere in 4-dimensional Euclidean space.
Euclidean coordinates in (n + 1)-space
The set of points in (n + 1)-space, (x1, x2, ..., xn+1), that define an n-sphere, , is represented by the equation:
where c = (c1, c2, ..., cn+1) is a center point, and r is the radius.
The above n-sphere exists in (n + 1)-dimensional Euclidean space and is an example of an n-manifold. The volume form ω of an n-sphere of radius r is given by
where ∗ is the Hodge star operator; see Flanders (1989, §6.1) for a discussion and proof of this formula in the case r = 1. As a result,
n-ball
The space enclosed by an n-sphere is called an (n + 1)-ball. An (n + 1)-ball is closed if it includes the n-sphere, and it is open if it does not include the n-sphere.
Specifically:
Topological description
Topologically, an n-sphere can be constructed as a one-point compactification of n-dimensional Euclidean space. Briefly, the n-sphere can be described as Sn = Rn ∪ {∞}, which is n-dimensional Euclidean space plus a single point representing infinity in all directions. In particular, if a single point is removed from an n-sphere, it becomes homeomorphic to Rn. This forms the basis for stereographic projection.[1]
Volume and surface area
Vn(R) and Sn(R) are the n-dimensional volume of the n-ball and the surface area of the n-sphere embedded in dimension n + 1, respectively, of radius R.
The constants Vn and Sn (for R = 1, the unit ball and sphere) are related by the recurrences:
The surfaces and volumes can also be given in closed form:
where Γ is the gamma function. Derivations of these equations are given in this section.
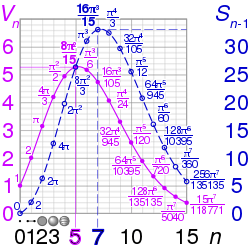
In theory, one could compare the values of Sn(R) and Sm(R) for n ≠ m. However, this is not well-defined. For example, if n = 2 and m = 3 then the comparison is like comparing a number of square meters to a different number of cubic meters. The same applies to a comparison of Vn(R) and Vm(R) for n ≠ m.
Examples
The 0-ball consists of a single point. The 0-dimensional Hausdorff measure is the number of points in a set. So,
The unit 1-ball is the interval [−1,1] of length 2. So,
The 0-sphere consists of its two end-points, {−1,1}. So,
The unit 1-sphere is the unit circle in the Euclidean plane, and this has circumference (1-dimensional measure)
The region enclosed by the unit 1-sphere is the 2-ball, or unit disc, and this has area (2-dimensional measure)
Analogously, in 3-dimensional Euclidean space, the surface area (2-dimensional measure) of the unit 2-sphere is given by
and the volume enclosed is the volume (3-dimensional measure) of the unit 3-ball, given by
Recurrences
The surface area, or properly the n-dimensional volume, of the n-sphere at the boundary of the (n + 1)-ball of radius R is related to the volume of the ball by the differential equation
or, equivalently, representing the unit n-ball as a union of concentric (n − 1)-sphere shells,
So,
We can also represent the unit (n + 2)-sphere as a union of tori, each the product of a circle (1-sphere) with an n-sphere. Let r = cos θ and r2 + R2 = 1, so that R = sin θ and dR = cos θ dθ. Then,
Since S1 = 2π V0, the equation
holds for all n.
This completes the derivation of the recurrences:
Closed forms
Combining the recurrences, we see that
So it is simple to show by induction on k that,
where !! denotes the double factorial, defined for odd natural numbers 2k + 1 by (2k + 1)!! = 1 × 3 × 5 × ... × (2k − 1) × (2k + 1) and similarly for even numbers (2k)!! = 2 × 4 × 6 × ... × (2k − 2) × (2k).
In general, the volume, in n-dimensional Euclidean space, of the unit n-ball, is given by
where Γ is the gamma function, which satisfies Γ(1/2) = √π, Γ(1) = 1, and Γ(x + 1) = xΓ(x), and so Γ(x + 1) = x!, and where we conversely define x! = Γ(x + 1) for any x.
By multiplying Vn by Rn, differentiating with respect to R, and then setting R = 1, we get the closed form
for the (n-1)-dimensional volume of the sphere Sn-1.
Other relations
The recurrences can be combined to give a "reverse-direction" recurrence relation for surface area, as depicted in the diagram:
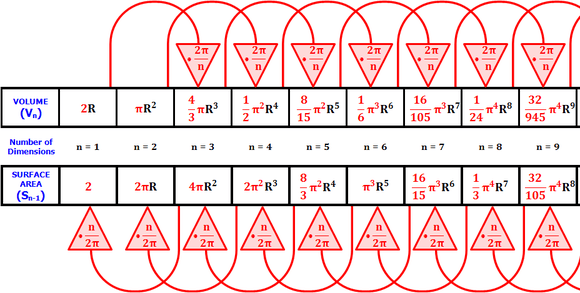
Index-shifting n to n − 2 then yields the recurrence relations:
where S0 = 2, V1 = 2, S1 = 2π and V2 = π.
The recurrence relation for Vn can also be proved via integration with 2-dimensional polar coordinates:
Spherical coordinates
We may define a coordinate system in an n-dimensional Euclidean space which is analogous to the spherical coordinate system defined for 3-dimensional Euclidean space, in which the coordinates consist of a radial coordinate r, and n − 1 angular coordinates φ1, φ2, ... φn−1, where the angles φ1, φ2, ... φn−2 range over [0,π] radians (or over [0,180] degrees) and φn−1 ranges over [0,2π) radians (or over [0,360) degrees). If xi are the Cartesian coordinates, then we may compute x1, ... xn from r, φ1, ... φn−1 with: [2]
Except in the special cases described below, the inverse transformation is unique:
where if xk ≠ 0 for some k but all of xk+1, ... xn are zero then φk = 0 when xk > 0, and φk = π (180 degrees) when xk < 0.
There are some special cases where the inverse transform is not unique; φk for any k will be ambiguous whenever all of xk, xk+1, ... xn are zero; in this case φk may be chosen to be zero.
Spherical volume and area elements
Expressing the angular measures in radians, the volume element in n-dimensional Euclidean space will be found from the Jacobian of the transformation:
and the above equation for the volume of the n-ball can be recovered by integrating:
Similarly the surface area element of the (n − 1)-sphere, which generalizes the area element of the 2-sphere, is given by
The natural choice of an orthogonal basis over the angular coordinates is a product of ultraspherical polynomials,
for j = 1, 2,... n − 2, and the eisφj for the angle j = n − 1 in concordance with the spherical harmonics.
Stereographic projection
Just as a two-dimensional sphere embedded in three dimensions can be mapped onto a two-dimensional plane by a stereographic projection, an n-sphere can be mapped onto an n-dimensional hyperplane by the n-dimensional version of the stereographic projection. For example, the point [x,y,z] on a two-dimensional sphere of radius 1 maps to the point [x/1 − z,y/1 − z] on the xy-plane. In other words,
Likewise, the stereographic projection of an n-sphere Sn−1 of radius 1 will map to the (n − 1)-dimensional hyperplane Rn−1 perpendicular to the xn-axis as
Generating random points
Uniformly at random on the (n − 1)-sphere
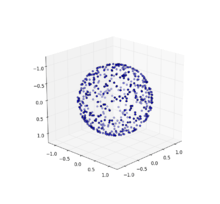
To generate uniformly distributed random points on the unit (n − 1)-sphere (that is, the surface of the unit n-ball), Marsaglia (1972) gives the following algorithm.
Generate an n-dimensional vector of normal deviates (it suffices to use N(0, 1), although in fact the choice of the variance is arbitrary), x = (x1, x2,... xn). Now calculate the "radius" of this point:
The vector 1/rx is uniformly distributed over the surface of the unit n-ball.
An alternative given by Marsaglia is to uniformly randomly select a point x = (x1, x2,... xn) in the unit n-cube by sampling each xi independently from the uniform distribution over (–1,1), computing r as above, and rejecting the point and resampling if r ≥ 1 (i.e., if the point is not in the n-ball), and when a point in the ball is obtained scaling it up to the spherical surface by the factor 1/r; then again 1/rx is uniformly distributed over the surface of the unit n-ball.
Uniformly at random within the n-ball
With a point selected uniformly at random from the surface of the unit (n - 1)-sphere (e.g., by using Marsaglia's algorithm), one needs only a radius to obtain a point uniformly at random from within the unit n-ball. If u is a number generated uniformly at random from the interval [0, 1] and x is a point selected uniformly at random from the unit (n - 1)-sphere, then u1⁄nx is uniformly distributed within the unit n-ball.
Alternatively, points may be sampled uniformly from within the unit n-ball by a reduction from the unit (n + 1)-sphere. In particular, if (x1,x2,...,xn+2) is a point selected uniformly from the unit (n + 1)-sphere, then (x1,x2,...,xn) is uniformly distributed within the unit n-ball (i.e., by simply discarding two coordinates).[3]
If n is sufficiently large, most of the volume of the n-ball will be contained in the region very close to its surface, so a point selected from that volume will also probably be close to the surface. This is one of the phenomena leading to the so-called curse of dimensionality that arises in some numerical and other applications.
Specific spheres
- 0-sphere
- The pair of points {±R} with the discrete topology for some R > 0. The only sphere that is not path-connected. Has a natural Lie group structure; isomorphic to O(1). Parallelizable.
- 1-sphere
- Also known as the circle. Has a nontrivial fundamental group. Abelian Lie group structure U(1); the circle group. Topologically equivalent to the real projective line, RP1. Parallelizable. SO(2) = U(1).
- 2-sphere
- Also known as the sphere. Complex structure; see Riemann sphere. Equivalent to the complex projective line, CP1. SO(3)/SO(2).
- 3-sphere
- Also known as the glome. Parallelizable, principal U(1)-bundle over the 2-sphere, Lie group structure Sp(1), where also
- .
- 4-sphere
- Equivalent to the quaternionic projective line, HP1. SO(5)/SO(4).
- 5-sphere
- Principal U(1)-bundle over CP2. SO(6)/SO(5) = SU(3)/SU(2).
- 6-sphere
- Possesses an almost complex structure coming from the set of pure unit octonions. SO(7)/SO(6) = G2/SU(3). The question of whether it has a complex structure is known as the Hopf problem, after Heinz Hopf.[4]
- 7-sphere
- Topological quasigroup structure as the set of unit octonions. Principal Sp(1)-bundle over S4. Parallelizable. SO(8)/SO(7) = SU(4)/SU(3) = Sp(2)/Sp(1) = Spin(7)/G2 = Spin(6)/SU(3). The 7-sphere is of particular interest since it was in this dimension that the first exotic spheres were discovered.
- 8-sphere
- Equivalent to the octonionic projective line OP1.
- 23-sphere
- A highly dense sphere-packing is possible in 24-dimensional space, which is related to the unique qualities of the Leech lattice.
Octahedral sphere
The octahedral n-sphere is defined similarly to the n-sphere but using the 1-norm:The octahedral 1-sphere is a square (without its interior). The octahedral 2-sphere is a regular octahedron; hence the name. The octahedral n-sphere is the topological join of n+1 pairs of isolated points.[5] Intuitively, the topological join of two pairs is generated by drawing a segment between each point in one pair and each point in the other pair; this yields a square. To join this with a third pair, draw a segment between each point on the square and each point in the third pair; this gives a octahedron.
See also
Notes
- James W. Vick (1994). Homology theory, p. 60. Springer
- Blumenson, L. E. (1960). "A Derivation of n-Dimensional Spherical Coordinates". The American Mathematical Monthly. 67 (1): 63–66. doi:10.2307/2308932. JSTOR 2308932.
- Voelker, Aaron R.; Gosmann, Jan; Stewart, Terrence C. (2017). Efficiently sampling vectors and coordinates from the n-sphere and n-ball (Report). Centre for Theoretical Neuroscience. doi:10.13140/RG.2.2.15829.01767/1.
- Agricola, Ilka; Bazzoni, Giovanni; Goertsches, Oliver; Konstantis, Panagiotis; Rollenske, Sönke (2018). "On the history of the Hopf problem". Differential Geometry and Its Applications. 57: 1–9. arXiv:1708.01068. doi:10.1016/j.difgeo.2017.10.014.
- Meshulam, Roy (2001-01-01). "The Clique Complex and Hypergraph Matching". Combinatorica. 21 (1): 89–94. doi:10.1007/s004930170006. ISSN 1439-6912.
References
- Flanders, Harley (1989). Differential forms with applications to the physical sciences. New York: Dover Publications. ISBN 978-0-486-66169-8.
- Moura, Eduarda; Henderson, David G. (1996). Experiencing geometry: on plane and sphere. Prentice Hall. ISBN 978-0-13-373770-7 (Chapter 20: 3-spheres and hyperbolic 3-spaces).
- Weeks, Jeffrey R. (1985). The Shape of Space: how to visualize surfaces and three-dimensional manifolds. Marcel Dekker. ISBN 978-0-8247-7437-0 (Chapter 14: The Hypersphere).
- Marsaglia, G. (1972). "Choosing a Point from the Surface of a Sphere". Annals of Mathematical Statistics. 43 (2): 645–646. doi:10.1214/aoms/1177692644.
- Huber, Greg (1982). "Gamma function derivation of n-sphere volumes". Amer. Math. Monthly. 89 (5): 301–302. doi:10.2307/2321716. JSTOR 2321716. MR 1539933.
- Barnea, Nir (1999). "Hyperspherical functions with arbitrary permutational symmetry: Reverse construction". Phys. Rev. A. 59 (2): 1135–1146. Bibcode:1999PhRvA..59.1135B. doi:10.1103/PhysRevA.59.1135.
