1,000,000
1,000,000 (one million), or one thousand thousand, is the natural number following 999,999 and preceding 1,000,001. The word is derived from the early Italian millione (milione in modern Italian), from mille, "thousand", plus the augmentative suffix -one.[1] It is commonly abbreviated as m[2][3][4] (not to be confused with the metric prefix for 1×10−3) or M[5][6] and MM ("thousand thousands", from Latin "Mille"; not to be confused with the Roman numeral MM = 2,000), mm, or mn in financial contexts.[7]
| ||||
|---|---|---|---|---|
| Cardinal | one million | |||
| Ordinal | 1000000th (one millionth) | |||
| Factorization | 26 × 56 | |||
| Greek numeral | ||||
| Roman numeral | M | |||
| Binary | 111101000010010000002 | |||
| Ternary | 12122102020013 | |||
| Octal | 36411008 | |||
| Duodecimal | 40285412 | |||
| Hexadecimal | F424016 | |||
| Look up million in Wiktionary, the free dictionary. |
In scientific notation, it is written as 1×106 or 106.[8] Physical quantities can also be expressed using the SI prefix mega (M), when dealing with SI units; for example, 1 megawatt (1 MW) equals 1,000,000 watts.
The meaning of the word "million" is common to the short scale and long scale numbering systems, unlike the larger numbers, which have different names in the two systems.
The million is sometimes used in the English language as a metaphor for a very large number, as in "Not in a million years" and "You're one in a million", or a hyperbole, as in "I've walked a million miles" and "You've asked a million-dollar question".
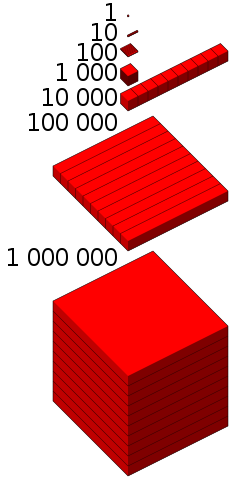
Visualizing one million
Even though it is often stressed that counting to precisely a million would be an exceedingly tedious task due to the time and concentration required, there are many ways to bring the number "down to size" in approximate quantities, ignoring irregularities or packing effects.
- Information: Not counting spaces, the text printed on 136 pages of an Encyclopædia Britannica, or 600 pages of pulp paperback fiction contains approximately one million characters.
- Length: There are one million millimetres in a kilometre, and roughly a million sixteenths of an inch in a mile. A typical car tire might rotate a million times in a 1,900-kilometre (1,200 mi) trip, while the engine would do several times that number of revolutions.
- Fingers: If the width of a human finger is 22 mm (7⁄8 in), then a million fingers lined up would cover a distance of 22 km (14 mi). If a person walks at a speed of 4 km/h (2.5 mph), it would take them approximately five and a half hours to reach the end of the fingers.
- Area: A square a thousand objects or units on a side contains a million such objects or square units, so a million holes might be found in less than three square yards of window screen, or similarly, in about one half square foot (400–500 cm2) of bed sheet cloth. A city lot 70 by 100 feet is about a million square inches.
- Volume: The cube root of one million is one hundred, so a million objects or cubic units is contained in a cube a hundred objects or linear units on a side. A million grains of table salt or granulated sugar occupies about 64 mL (2.3 imp fl oz; 2.2 US fl oz), the volume of a cube one hundred grains on a side. One million cubic inches would be the volume of a small room 8 1⁄3 feet long by 8 1⁄3 feet wide by 8 1⁄3 feet high.
- Mass: A million cubic millimetres (small droplets) of water would have a volume of one litre and a mass of one kilogram. A million millilitres or cubic centimetres (one cubic metre) of water has a mass of a million grams or one tonne.
- Weight: A million 80-milligram (1.2 gr) honey bees would weigh the same as an 80 kg (180 lb) person.
- Landscape: A pyramidal hill 600 feet (180 m) wide at the base and 100 feet (30 m) high would weigh about a million short tons.
- Computer: A display resolution of 1,280 by 800 pixels contains 1,024,000 pixels.
- Money: A USD bill of any denomination weighs 1 gram (0.035 oz). There are 454 grams in a pound. One million USD bills would weigh 1 megagram (1,000 kg; 2,200 lb) or 1 tonne (just over 1 short ton).
- Time: A million seconds, 1 megasecond, is 11.57 days.
In Indian English and Pakistani English, it is also expressed as 10 lakh. Lakh is derived from lakṣa for 100,000 in Sanskrit.

Selected 7-digit numbers (1,000,001–9,999,999)
1,000,001 to 1,999,999
- 1,000,003 – Smallest 7-digit prime number
- 1,000,405 – Smallest triangular number with 7 digits and the 1,414th triangular number
- 1,006,301 – First number of the first pair of prime quadruplets occurring thirty apart ({1006301, 1006303, 1006307, 1006309} and {1006331, 1006333, 1006337, 1006339})[9]
- 1,024,000 – Sometimes, the number of bytes in a megabyte[10]
- 1,046,527 – Carol number[11]
- 1,048,576 – 220 (power of two, the number of bytes in a mebibyte (or often, a megabyte)
- 1,048,976 – Leyland number
- 1,050,623 – Kynea number[12]
- 1,058,576 – Leyland number
- 1,084,051 – Keith number[13]
- 1,089,270 – harmonic divisor number[14]
- 1,111,111 – repunit
- 1,136,689 – Pell number,[15] Markov number
- 1,278,818 – Markov number
- 1,299,709 – 100,000th prime number
- 1,346,269 – Fibonacci number,[16] Markov number
- 1,413,721 – square triangular number[17]
- 1,419,857 – 175
- 1,421,280 – harmonic divisor number[14]
- 1,441,440 – colossally abundant number,[18] superior highly composite number[19]
- 1,441,889 – Markov number
- 1,539,720 – harmonic divisor number[14]
- 1,563,372 – Wedderburn-Etherington number[20]
- 1,594,323 – 313
- 1,596,520 – Leyland number
- 1,647,086 – Leyland number
- 1,671,800 – Initial number of first century xx00 to xx99 consisting entirely of composite numbers[21]
- 1,679,616 – 68
- 1,686,049 – Markov number
- 1,741,725 – equal to the sum of the seventh power of its digits
- 1,771,561 – 116, also, Commander Spock's estimate for the tribble population in the Star Trek episode "The Trouble with Tribbles"
- 1,889,568 – 185
- 1,941,760 – Leyland number
- 1,953,125 – 59
2,000,000 to 2,999,999
- 2,012,174 – Leyland number
- 2,012,674 – Markov number
- 2,097,152 – 221
- 2,097,593 – prime Leyland number[22]
- 2,124,679 – Wolstenholme prime[23]
- 2,178,309 – Fibonacci number[16]
- 2,222,222 – repdigit
- 2,356,779 – Motzkin number[24]
- 2,423,525 – Markov number
- 2,476,099 – 195
- 2,674,440 – Catalan number[25]
- 2,744,210 – Pell number[15]
- 2,796,203 – Wagstaff prime[26]
- 2,890,625 – 1-automorphic number[27]
- 2,922,509 – Markov number
- 2,985,984 – 126
3,000,000 to 3,999,999
- 3,200,000 – 205
- 3,263,442 – product of the first five terms of Sylvester's sequence
- 3,263,443 – sixth term of Sylvester's sequence[28]
- 3,276,509 – Markov number
- 3,301,819 – alternating factorial[29]
- 3,333,333 – repdigit
- 3,360,633 – palindromic in 3 consecutive bases: 62818269 = 336063310 = 199599111
- 3,524,578 – Fibonacci number,[16] Markov number
- 3,626,149 – Wedderburn-Etherington number[20]
- 3,628,800 – 10! (factorial of ten)
4,000,000 to 4,999,999
- 4,037,913 – sum of the first ten factorials
- 4,084,101 – 215
- 4,190,207 – Carol number[11]
- 4,194,304 – 222
- 4,194,788 – Leyland number
- 4,198,399 – Kynea number[12]
- 4,208,945 – Leyland number
- 4,210,818 – equal to the sum of the seventh powers of its digits
- 4,213,597 – Bell number[30]
- 4,324,320 – colossally abundant number,[18] superior highly composite number,[19] pronic number
- 4,400,489 – Markov number
- 4,444,444 – repdigit
- 4,782,969 – 314
- 4,785,713 – Leyland number
- 4,826,809 – 136
5,000,000 to 5,999,999
- 5,134,240 – the largest number that cannot be expressed as the sum of distinct fourth powers
- 5,153,632 – 225
- 5,496,925 – first cyclic number in base 6
- 5,555,555 – repdigit
- 5,702,887 – Fibonacci number[16]
- 5,764,801 – 78
- 5,882,353 – 5882 + 23532
6,000,000 to 6,999,999
7,000,000 to 7,999,999
- 7,109,376 – 1-automorphic number[27]
- 7,453,378 – Markov number
- 7,529,536 – 146
- 7,652,413 – Largest n-digit pandigital prime
- 7,777,777 – repdigit
- 7,779,311 – A hit song written by Prince and released in 1982 by The Time
- 7,861,953 – Leyland number
- 7,913,837 – Keith number[13]
- 7,962,624 – 245
8,000,000 to 8,999,999
- 8,000,000 – Used to represent infinity in Japanese mythology
- 8,108,731 – repunit prime in base 14
- 8,388,608 – 223
- 8,389,137 – Leyland number
- 8,399,329 – Markov number
- 8,436,379 – Wedderburn-Etherington number[20]
- 8,675,309 – A hit song for Tommy Tutone (also a twin prime)
- 8,675,311 – A twin prime
- 8,888,888 – repdigit
- 8,946,176 – self-descriptive number in base 8
9,000,000 to 9,999,999
- 9,227,465 – Fibonacci number,[16] Markov number
- 9,369,319 – Newman–Shanks–Williams prime[31]
- 9,647,009 – Markov number
- 9,694,845 – Catalan number[25]
- 9,765,625 – 510
- 9,800,817 – equal to the sum of the seventh powers of its digits
- 9,865,625 – Leyland number
- 9,926,315 – equal to the sum of the seventh powers of its digits
- 9,997,156 – largest triangular number with 7 digits and the 4,471st triangular number
- 9,999,991 – Largest 7-digit prime number
- 9,999,999 – repdigit
See also
- Huh (god), depictions of whom were also used in hieroglyphs to represent one million
- Megagon
- Names of large numbers
- Orders of magnitude (numbers) to help compare dimensionless numbers between 1,000,000 and 10,000,000 (106 and 107).
References
- "million". Dictionary.com Unabridged. Random House, Inc. Retrieved 4 October 2010.
- "m". Oxford Dictionaries. Oxford University Press. Retrieved 2015-06-30.
- "figures". The Economist Style Guide (11th ed.). The Economist. 2015.
- "6.7 Abbreviating 'million' and 'billion'". English Style Guide. A handbook for authors and translators in the European Commission (PDF) (2019 ed.). 26 February 2019. p. 37.
- "M". Merriam-Webster. Merriam-Webster Inc. Retrieved 2015-06-30.
- "Definition of "m"". Collins English Dictionary. HarperCollins Publishers. Retrieved 2015-06-30.
- Averkamp, Harold. "Q&A: What Does M and MM Stand For?". AccountingCoach.com. AccountingCoach, LLC. Retrieved 25 June 2015.
- David Wells (1987). The Penguin Dictionary of Curious and Interesting Numbers. London: Penguin Group. p. 185.
1,000,000 = 106
- Sloane, N. J. A. (ed.). "Sequence A059925 (Initial members of two prime quadruples (A007530) with the smallest possible difference of 30.)". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2019-01-27.
- Tracing the History of the Computer - History of the Floppy Disk
- "Sloane's A093112 : a(n) = (2^n-1)^2 - 2". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-17.
- "Sloane's A093069 : a(n) = (2^n + 1)^2 - 2". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-17.
- "Sloane's A007629 : Repfigit (REPetitive FIbonacci-like diGIT) numbers (or Keith numbers)". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-17.
- "Sloane's A001599 : Harmonic or Ore numbers". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-17.
- "Sloane's A000129 : Pell numbers". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-17.
- "Sloane's A000045 : Fibonacci numbers". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-17.
- "Sloane's A001110 : Square triangular numbers". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-17.
- "Sloane's A004490 : Colossally abundant numbers". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-17.
- "Sloane's A002201 : Superior highly composite numbers". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-17.
- "Sloane's A001190 : Wedderburn-Etherington numbers". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-17.
- Sloane, N. J. A. (ed.). "Sequence A181098 (Primefree centuries)". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2019-01-27.
- "Sloane's A094133 : Leyland primes". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-17.
- "Wolstenholme primes". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-17.
- "Sloane's A001006 : Motzkin numbers". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-17.
- "Sloane's A000108 : Catalan numbers". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-17.
- "Sloane's A000979 : Wagstaff primes". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-17.
- Sloane, N. J. A. (ed.). "Sequence A003226 (Automorphic numbers)". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2019-04-06.
- "Sloane's A000058 : Sylvester's sequence". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-17.
- "Sloane's A005165 : Alternating factorials". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-17.
- "Sloane's A000110 : Bell or exponential numbers". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-17.
- "Sloane's A088165 : NSW primes". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-17.