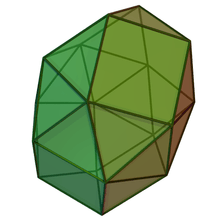
Gyroelongated triangular bicupola

In geometry, the gyroelongated triangular bicupola is one of the Johnson solids (J44). As the name suggests, it can be constructed by gyroelongating a triangular bicupola (either J27 or the cuboctahedron) by inserting a hexagonal antiprism between its congruent halves.

Gyroelongated triangular bicupola
TypeJohnson
J43 - J44 - J45
Faces2+3×6 triangles
6 squares
Edges42
Vertices18
Vertex configuration6(3.4.3.4)
2.6(34.4)
Symmetry groupD3
Dual polyhedron-
Propertiesconvex, chiral
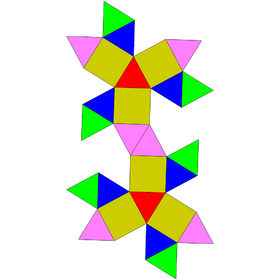
Net

A Johnson solid is one of 92 strictly convex polyhedra that is composed of regular polygon faces but are not uniform polyhedra (that is, they are not Platonic solids, Archimedean solids, prisms, or antiprisms). They were named by Norman Johnson, who first listed these polyhedra in 1966.[1]

The gyroelongated triangular bicupola is one of five Johnson solids which are chiral, meaning that they have a "left-handed" and a "right-handed" form. In the illustration to the right, each square face on the bottom half of the figure is connected by a path of two triangular faces to a square face above it and to the right. In the figure of opposite chirality (the mirror image of the illustrated figure), each bottom square would be connected to a square face above it and to the left. The two chiral forms of J44 are not considered different Johnson solids.

Formulae

The following formulae for volume and surface area can be used if all faces are regular, with edge length a:[2]

gollark: *AMD* outputs more heat? Really?
gollark: Ideally modded minecraft, which has more interesting content.
gollark: It's pretty fun. Especially with mods. Subjectively.
gollark: That's a bizarrely specific list.
gollark: Or, yield, not return.

References
  1. Johnson, Norman W. (1966), "Convex polyhedra with regular faces", Canadian Journal of Mathematics, 18: 169–200, doi:10.4153/cjm-1966-021-8, MR 0185507, Zbl 0132.14603.
  2. Stephen Wolfram, "Gyroelongated triangular bicupola" from Wolfram Alpha. Retrieved July 30, 2010.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.