Square planar molecular geometry

The square planar molecular geometry in chemistry describes the stereochemistry (spatial arrangement of atoms) that is adopted by certain chemical compounds. As the name suggests, molecules of this geometry have their atoms positioned at the corners of a square on the same plane about a central atom.

Square planar molecular geometry
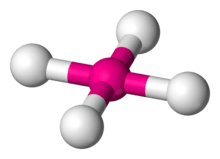ExamplesXeF4, PtCl2−
4
Point groupD4h
Coordination number4
Bond angle(s)90°
μ (Polarity)0
Structure of cisplatin, an example of a molecule with the square planar coordination geometry.

Relationship to other geometries

Square-planar coordination geometry violates the points-on-sphere geometries observed from most compounds (i.e. linear, trigonal, tetrahedral, trigonal bipyramidal, ...). The isolation of both trans- and cis-PtCl2(NH3)2 led Alfred Werner to propose square planar molecular geometry.[1]

XeF2, with a linear geometry, which can gain two fluorine atoms to form...
XeF4, with a square planar geometry, which can gain two fluorine atoms to form...
XeF6, with a distorted octahedral geometry.

Linear

The addition of two ligands to linear compounds, ML2, can afford square planar complexes. For example, XeF2 adds fluorine to give square planar XeF4.

Tetrahedral molecular geometry

In principle, square planar geometry can be achieved by flattening a tetrahedron. As such, the interconversion of tetrahedral and square planar geometries provides an intramolecular pathway for the isomerization of tetrahedral compounds. This pathway does not operate readily for hydrocarbons, but tetrahedral nickel(II) complexes, e.g. NiBr2(PPh3)2, undergo this change reversibly.

Octahedral geometry

Square planar geometry can also be achieved by the removal of a pair of ligands from the z-axis of an octahedron, leaving four ligands in the xy plane. For transition metal compounds, the crystal field splitting diagram for square planar geometry can thus be derived from the octahedral diagram. The removal of the two ligands stabilizes the dz2 level, leaving the dx2−y2 level as the most destabilized. Consequently, the dx2−y2 remains unoccupied in complexes of metals with the d8 configuration. These compounds typically have 16 valence electrons (eight from ligands, eight from the metal).[2]

Examples

Numerous compounds adopt this geometry, examples being especially numerous for transition metal complexes. The noble gas compound XeF4 adopts this structure as predicted by VSEPR theory. The geometry is prevalent for transition metal complexes with d8 configuration, which includes Rh(I), Ir(I), Pd(II), Pt(II), and Au(III). Notable examples include the anticancer drugs cisplatin [PtCl2(NH3)2] and carboplatin. Many homogeneous catalysts are square planar in their resting state, such as Wilkinson's catalyst and Crabtree's catalyst. Other examples include Vaska's complex and Zeise's salt. Certain ligands (such as porphyrins) stabilize this geometry.

Splitting of the energy of the d-orbitals in square planar transition metal complexes
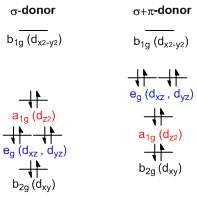
Representative d-orbital splitting diagrams for square planar complexes featuring σ-donor (left) and σ+π-donor (right) ligands.

A general d-orbital splitting diagram for square planar (D4h) transition metal complexes can be derived from the general octahedral (Oh) splitting diagram, in which the dz2 and the dx2−y2 orbitals are degenerate and higher in energy than the degenerate set of dxy, dxz and dyz orbitals. When the two axial ligands are removed to generate a square planar geometry, the dz2 orbital is driven lower in energy as electron-electron repulsion with ligands on the z-axis is no longer present. However, for purely σ-donating ligands the dz2 orbital is still higher in energy than the dxy, dxz and dyz orbitals because of the torus shaped lobe of the dz2 orbital. It bears electron density on the x- and y-axes and therefore interacts with the filled ligand orbitals. The dxy, dxz and dyz orbitals are generally presented as degenerate but they have to split into two different energy levels with respect to the irreducible representations of the point group D4h. Their relative ordering depends on the nature of the particular complex. Furthermore, the splitting of d-orbitals is perturbed by π-donating ligands in contrast to octahedral complexes. In the square planar case strongly π-donating ligands can cause the dxz and dyz orbitals to be higher in energy than the dz2 orbital, whereas in the octahedral case π-donating ligands only affect the magnitude of the d-orbital splitting and the relative ordering of the orbitals is conserved.[3]

gollark: I had that experience yesterday. It is best avoided.
gollark: Oh no, did you look at a PHP program?
gollark: And contains Nim's traditional thousand-line source files.
gollark: Huh, neat, the Nim forum is actually written in Nim.
gollark: What is it even complaining about? All the integrity checks go back fine.

See also

References
  1. George B. Kauffman, Dwaine O. Cowan (1963). cis- and trans-Dichlorodiammineplatinum(II). Inorg. Synth. Inorganic Syntheses. 7. pp. 239–245. doi:10.1002/9780470132388.ch63. ISBN 9780470132388.CS1 maint: uses authors parameter (link)
  2. Miessler, G. L.; Tarr, D. A. (2004). Inorganic Chemistry (3rd ed.). Pearson/Prentice Hall. ISBN 978-0-13-035471-6.
  3. Börgel, Jonas; Campbell, Michael G.; Ritter, Tobias (2016-01-12). "Transition Metal d-Orbital Splitting Diagrams: An Updated Educational Resource for Square Planar Transition Metal Complexes". Journal of Chemical Education. 93 (1): 118–121. Bibcode:2016JChEd..93..118B. doi:10.1021/acs.jchemed.5b00542. ISSN 0021-9584.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.