Filled Julia set

The filled-in Julia set ${\displaystyle \ K(f)}$ of a polynomial ${\displaystyle \ f}$ is :

• a Julia set and its interior,
• non-escaping set

Formal definition

The filled-in Julia set ${\displaystyle \ K(f)}$ of a polynomial ${\displaystyle \ f}$ is defined as the set of all points ${\displaystyle z\,}$ of the dynamical plane that have bounded orbit with respect to ${\displaystyle \ f}$

${\displaystyle \ K(f)\ {\overset {\underset {\mathrm {def} }{}}{=}}\ \{z\in \mathbb {C} :f^{(k)}(z)\not \to \infty \ as\ k\to \infty \}}$
where :

${\displaystyle \mathbb {C} }$ is the set of complex numbers

${\displaystyle \ f^{(k)}(z)}$ is the ${\displaystyle \ k}$ -fold composition of ${\displaystyle f\,}$ with itself = iteration of function ${\displaystyle f\,}$

Relation to the Fatou set

The filled-in Julia set is the (absolute) complement of the attractive basin of infinity.
${\displaystyle K(f)=\mathbb {C} \setminus A_{f}(\infty )}$

The attractive basin of infinity is one of the components of the Fatou set.
${\displaystyle A_{f}(\infty )=F_{\infty }}$

In other words, the filled-in Julia set is the complement of the unbounded Fatou component:
${\displaystyle K(f)=F_{\infty }^{C}.}$

Relation between Julia, filled-in Julia set and attractive basin of infinity

The Julia set is the common boundary of the filled-in Julia set and the attractive basin of infinity

${\displaystyle J(f)\,=\partial K(f)=\partial A_{f}(\infty )}$

where :
${\displaystyle A_{f}(\infty )}$ denotes the attractive basin of infinity = exterior of filled-in Julia set = set of escaping points for ${\displaystyle f}$

${\displaystyle A_{f}(\infty )\ {\overset {\underset {\mathrm {def} }{}}{=}}\ \{z\in \mathbb {C} :f^{(k)}(z)\to \infty \ as\ k\to \infty \}.}$

If the filled-in Julia set has no interior then the Julia set coincides with the filled-in Julia set. This happens when all the critical points of ${\displaystyle f}$ are pre-periodic. Such critical points are often called Misiurewicz points.

Spine

• 
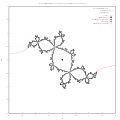  Rabbit Julia set with spine
• 
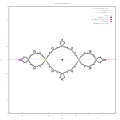  Basilica Julia set with spine

The most studied polynomials are probably those of the form ${\displaystyle f(z)=z^{2}+c}$, which are often denoted by ${\displaystyle f_{c}}$, where ${\displaystyle c}$ is any complex number. In this case, the spine ${\displaystyle S_{c}\,}$ of the filled Julia set ${\displaystyle \ K\,}$ is defined as arc between ${\displaystyle \beta \,}$-fixed point and ${\displaystyle -\beta \,}$,

${\displaystyle S_{c}=\left[-\beta ,\beta \right]\,}$

with such properties:

• spine lies inside ${\displaystyle \ K\,}$.[1] This makes sense when ${\displaystyle K\,}$ is connected and full[2]
• spine is invariant under 180 degree rotation,
• spine is a finite topological tree,
• Critical point ${\displaystyle z_{cr}=0\,}$ always belongs to the spine.[3]
• ${\displaystyle \beta \,}$-fixed point is a landing point of external ray of angle zero ${\displaystyle {\mathcal {R}}_{0}^{K}}$,
• ${\displaystyle -\beta \,}$ is landing point of external ray ${\displaystyle {\mathcal {R}}_{1/2}^{K}}$.

Algorithms for constructing the spine:

• detailed version is described by A. Douady[4]
• Simplified version of algorithm:
  • connect ${\displaystyle -\beta \,}$ and ${\displaystyle \beta \,}$ within ${\displaystyle K\,}$ by an arc,
  • when ${\displaystyle K\,}$ has empty interior then arc is unique,
  • otherwise take the shortest way that contains ${\displaystyle 0}$.[5]

Curve ${\displaystyle R\,}$ :

${\displaystyle R\ {\overset {\underset {\mathrm {def} }{}}{=}}\ R_{1/2}\ \cup \ S_{c}\ \cup \ R_{0}\,}$

divides dynamical plane into two components.

Images

• 
  Filled Julia set for f_c, c=φ−2=-0.38..., where φ means Golden ratio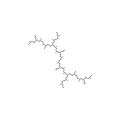
• 
  Filled Julia with no interior = Julia set. It is for c=i.
• 
  Filled Julia set for c=-1+0.1*i. Here Julia set is the boundary of filled-in Julia set.
• 
  Douady rabbit
• 
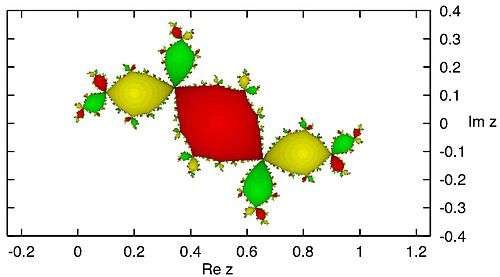
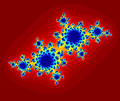  Filled Julia set for c = −0.4+0.6i.
• 
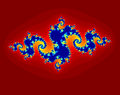  Filled Julia set for c = −0.8 + 0.156i.
• 
  Filled Julia set for c = 0.285 + 0.01i.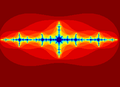
• 
  Filled Julia set for c = -1.476.

Names

• airplane[6]
• Douady rabbit
• dragon
• basilica or San Marco fractal
• cauliflower
• dendrite
• Siegel disc

Notes

1. Douglas C. Ravenel : External angles in the Mandelbrot set: the work of Douady and Hubbard. University of Rochester Archived 2012-02-08 at the Wayback Machine
2. John Milnor : Pasting Together Julia Sets: A Worked Out Example of Mating. Experimental Mathematics Volume 13 (2004)
3. Saaed Zakeri: Biaccessiblility in quadratic Julia sets I: The locally-connected case
4. A. Douady, “Algorithms for computing angles in the Mandelbrot set,” in Chaotic Dynamics and Fractals, M. Barnsley and S. G. Demko, Eds., vol. 2 of Notes and Reports in Mathematics in Science and Engineering, pp. 155–168, Academic Press, Atlanta, Georgia, USA, 1986.
5. K M. Brucks, H Bruin : Topics from One-Dimensional Dynamics Series: London Mathematical Society Student Texts (No. 62) page 257
6. The Mandelbrot Set And Its Associated Julia Sets by Hermann Karcher

References

1. Peitgen Heinz-Otto, Richter, P.H. : The beauty of fractals: Images of Complex Dynamical Systems. Springer-Verlag 1986. ISBN 978-0-387-15851-8.
2. Bodil Branner : Holomorphic dynamical systems in the complex plane. Department of Mathematics Technical University of Denmark, MAT-Report no. 1996-42.