Interior (topology)

In mathematics, specifically in topology, the interior of a subset $S$ of a topological space $X$ is the union of all subsets of $S$ that are open in $X$ . A point that is in the interior of $S$ is an interior point of $S$ .

The point

x

is an interior point of

S

. The point

y

is on the boundary of

S

.

The interior of $S$ is the complement of the closure of the complement of $S$ . In this sense interior and closure are dual notions.

The exterior of a set $S$ is the complement of the closure of $S$ ; it consists of the points that are in neither the set nor its boundary. The interior, boundary, and exterior of a subset together partition the whole space into three blocks (or fewer when one or more of these is empty). The interior and exterior are always open while the boundary is always closed. Sets with empty interior have been called boundary sets.[1]

Definitions

Interior point

If $S$ is a subset of a Euclidean space, then $x$ is an interior point of $S$ if there exists an open ball centered at $x$ which is completely contained in $S$ . (This is illustrated in the introductory section to this article.)

This definition generalizes to any subset $S$ of a metric space $X$ with metric $d$ : $x$ is an interior point of $S$ if there exists $r > 0$ , such that $y$ is in $S$ whenever the distance $d (x, y) < r$ .

This definition generalises to topological spaces by replacing "open ball" with "open set". Let $S$ be a subset of a topological space $X$ . Then $x$ is an interior point of $S$ if $x$ is contained in an open subset of $X$ which is completely contained in $S$ . (Equivalently, $x$ is an interior point of $S$ if $S$ is a neighbourhood of $x$ .)

Interior of a set

The interior of a subset $S$ of a topological space $X$ , denoted by $Int S$ or $S °$ , can be defined in any of the following equivalent ways:

$Int S$ is the largest open subset of $X$ contained (as a subset) in $S$ ;
$Int S$ is the union of all open sets of $X$ contained in $S$ ;
$Int S$ is the set of all interior points of $S$ .

Examples

a

is an interior point of

M

, because there is an ε-neighbourhood of a which is a subset of

M

.

In any space, the interior of the empty set is the empty set.
In any space $X$ , if $S \subseteq X$ , then $int S \subseteq S$ .
If $X$ is the Euclidean space $ℝ$ of real numbers, then $int([0, 1]) = (0, 1)$ .
If $X$ is the Euclidean space $ℝ$ , then the interior of the set $ℚ$ of rational numbers is empty.
If $X$ is the complex plane $\mathbb {C} =\mathbb {R} ^{2}$ , then $\mathrm {int} (\{z\in \mathbb {C$
In any Euclidean space, the interior of any finite set is the empty set.

On the set of real numbers, one can put other topologies rather than the standard one.

If $X = ℝ$ , where $ℝ$ has the lower limit topology, then int([0, 1]) = [0, 1).
If one considers on $ℝ$ the topology in which every set is open, then $int([0, 1]) = [0, 1]$ .
If one considers on $ℝ$ the topology in which the only open sets are the empty set and $ℝ$ itself, then $int([0, 1])$ is the empty set.

These examples show that the interior of a set depends upon the topology of the underlying space. The last two examples are special cases of the following.

In any discrete space, since every set is open, every set is equal to its interior.
In any indiscrete space $X$ , since the only open sets are the empty set and $X$ itself, we have $X = int X$ and for every proper subset $S$ of $X$ , $int S$ is the empty set.

Properties

Let $X$ be a topological space and let $S$ and $T$ be subset of $X$ .

$Int S$ is an open subset of $S$ and an open subset of $X$ .
$S$ is an open subset of $X$ if and only if $S = int S$ .
$Int(Int S) = Int S$ (idempotence).
If $S \subseteq T$ then $Int S \subseteq Int T$ .
If $A$ is an open subset of $X$ , then $A \subseteq S$ if and only if $A \subseteq Int S$ .
If $S$ is closed in $X$ and $Int T = \emptyset$ then $Int (S \cup T) = Int S$ .[2]

Note that these properties are also satisfied if "interior", "subset", "union", "contained in", "largest" and "open" are replaced by "closure", "superset", "intersection", "which contains", "smallest", and "closed", respectively. For more on this matter, see interior operator below.

Interior operator

The interior operator ^o is dual to the closure operator ^—, in the sense that

S^{\circ }=X\backslash {\overline {(X\backslash S)}}

,

and also

{\bar {S}}=X\backslash (X\backslash S)^{\circ }

,

where $X$ is the topological space containing $S$ , and the backslash refers to the set-theoretic difference.

Therefore, the abstract theory of closure operators and the Kuratowski closure axioms can be easily translated into the language of interior operators, by replacing sets with their complements.

In general, the interior operator does not commute with unions. However, in a complete metric space the following result does hold:

Theorem[3] (C. Ursescu) — Let $X$ be a complete metric space and let $S_{1},S_{2},\ldots$ be a sequence of subsets of $X$ .

If each $S i$ is closed in $X$ then $\operatorname {cl} \left(\cup _{i\in \mathbb {N} }\operatorname {int} S_{i}\right)=\operatorname {cl} \operatorname {int} \left(\cup _{i\in \mathbb {N} }S_{i}\right)$ .
If each $S i$ is open in $X$ then $\operatorname {int} \left(\cap _{i\in \mathbb {N} }\operatorname {cl} S_{i}\right)=\operatorname {int} \operatorname {cl} \left(\cap _{i\in \mathbb {N} }S_{i}\right)$ .

Exterior of a set

The exterior of a subset $S$ of a topological space $X$ , denoted $ext S$ or $Ext S$ , is the interior $int(X \ S)$ of its relative complement. Alternatively, it can be defined as $X \ S—$ , the complement of the closure of $S$ . Many properties follow in a straightforward way from those of the interior operator, such as the following.

$ext S$ is an open set that is disjoint with $S$ .
$ext S$ is the union of all open sets that are disjoint with $S$ .
$ext S$ is the largest open set that is disjoint with $S$ .
If $S \subseteq T$ , then $ext(S)$ is a superset of $ext T$ .

Unlike the interior operator, ext is not idempotent, but the following holds:

$ext(ext S)$ is a superset of $int S$ .

Interior-disjoint shapes

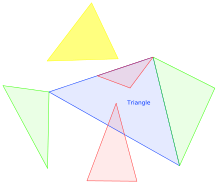
The red shapes are not interior-disjoint with the blue Triangle. The green and the yellow shapes are interior-disjoint with the blue Triangle, but only the yellow shape is entirely disjoint from the blue Triangle.

Two shapes $a$ and $b$ are called interior-disjoint if the intersection of their interiors is empty. Interior-disjoint shapes may or may not intersect in their boundary.

gollark: ?urban apioform

gollark: We are at the very least increasing awareness.

gollark: Where would you get ice? Why would you have it lying around?

gollark: Just like them then.

gollark: Just slow down the molecules in water?

References

Kuratowski, Kazimierz (1922). "Sur l'Operation Ā de l'Analysis Situs" (PDF). Fundamenta Mathematicae. Warsaw: Polish Academy of Sciences. 3: 182–199. ISSN 0016-2736.
Narici 2011, pp. 371-423.
Zalinescu, C (2002). Convex analysis in general vector spaces. River Edge, N.J. London: World Scientific. p. 33. ISBN 981-238-067-1. OCLC 285163112.

Narici, Lawrence; Beckenstein, Edward (2011). Topological Vector Spaces. Pure and applied mathematics (Second ed.). Boca Raton, FL: CRC Press. ISBN 978-1584888666. OCLC 144216834.

External links

Interior at PlanetMath.org.

This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.

[1] Kuratowski, Kazimierz (1922). "Sur l'Operation Ā de l'Analysis Situs" (PDF). Fundamenta Mathematicae. Warsaw: Polish Academy of Sciences. 3: 182–199. ISSN 0016-2736.

[FOOTNOTENarici2011371-423-2] Narici 2011, pp. 371-423.

[Zalinescu_2002_p._33-3] Zalinescu, C (2002). Convex analysis in general vector spaces. River Edge, N.J. London: World Scientific. p. 33. ISBN 981-238-067-1. OCLC 285163112.

Functional analysis (topics, glossary)
Spaces	Hilbert space, Banach space, Fréchet space, topological vector space
Theorems	Hahn–Banach theorem, closed graph theorem, uniform boundedness principle, Kakutani fixed-point theorem, Krein–Milman theorem, min-max theorem, Gelfand–Naimark theorem, Banach–Alaoglu theorem
Operators	bounded operator, compact operator, adjoint operator, unitary operator, Hilbert–Schmidt operator, trace class, unbounded operator
Algebras	Banach algebra, C-algebra, spectrum of a C-algebra, operator algebra, group algebra of a locally compact group, von Neumann algebra
Open problems	invariant subspace problem, Mahler's conjecture
Applications	Besov space, Hardy space, spectral theory of ordinary differential equations, heat kernel, index theorem, calculus of variation, functional calculus, integral operator, Jones polynomial, topological quantum field theory, noncommutative geometry, Riemann hypothesis
Advanced topics	locally convex space, approximation property, balanced set, Schwartz space, weak topology, barrelled space, Banach–Mazur distance, Tomita–Takesaki theory