Elongated pyramid
In geometry, the elongated pyramids are an infinite set of polyhedra, constructed by adjoining an n-gonal pyramid to an n-gonal prism. Along with the set of pyramids, these figures are topologically self-dual.
| Set of elongated pyramids | |
|---|---|
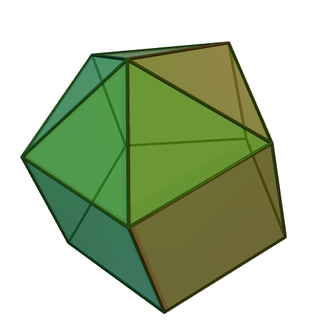 Example Pentagonal form | |
| Faces | n triangles n squares 1 n-gon |
| Edges | 4n |
| Vertices | 2n+1 |
| Symmetry group | Cnv, [n], (*nn) |
| Rotational group | Cn, [n]+, (nn) |
| Dual polyhedron | self-dual |
| Properties | convex |
There are three elongated pyramids that are Johnson solids made from regular triangles and square, and pentagons. Higher forms can be constructed with isosceles triangles.
Forms
| name | faces | |
|---|---|---|
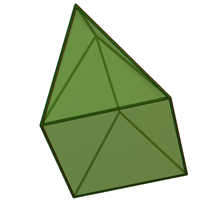 | elongated triangular pyramid (J7) | 3+1 triangles, 3 squares |
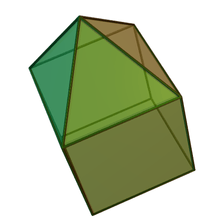 | elongated square pyramid (J8) | 4 triangles, 4+1 squares |
| elongated pentagonal pyramid (J9) | 5 triangles, 5 squares, 1 pentagon |
gollark: Why did you invent such PHPous software?
gollark: Oh no.
gollark: My friend's debian-running server is somehow still on nginx 1.14.2, when my up-to-date unnecessarily-compiled-from-source one is at 1.19.10 or so.
gollark: The Arch arm-none-eabi-gcc was apparently updated about 2 weeks ago, so presumably they aren't getting it from ARM themselves. So I don't see why Debian would be either. Maybe they're just hilariously outdated for no reason.
gollark: ARM publishes them? Aren't they compiled by debian themselves from something something GCC source repositories?
References
- Norman W. Johnson, "Convex Solids with Regular Faces", Canadian Journal of Mathematics, 18, 1966, pages 169–200. Contains the original enumeration of the 92 solids and the conjecture that there are no others.
- Victor A. Zalgaller (1969). Convex Polyhedra with Regular Faces. Consultants Bureau. No ISBN. The first proof that there are only 92 Johnson solids.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.