Bifrustum
An n-agonal bifrustum is a polyhedron composed of three parallel planes of n-agons, with the middle plane largest and usually the top and bottom congruent.
| Set of bifrusta | |
|---|---|
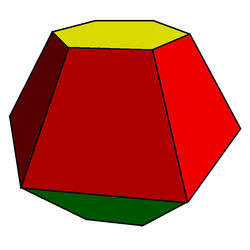 Example hexagonal bifrustum | |
| Faces | 2 n-gons, 2n trapezoids |
| Edges | 5n |
| Vertices | 3n |
| Symmetry group | Dnh, [n,2], (*n22) |
| Dual polyhedron | Elongated bipyramids |
| Properties | convex |
It can be constructed as two congruent frusta combined across a plane of symmetry, and also as a bipyramid with the two polar vertices truncated.
They are duals to the family of elongated bipyramids.
Forms
Three bifrustums are duals to three Johnson solids, J14-16. In general, a n-agonal bifrustum has 2n trapezoids, 2 n-agons, and is dual to the elongated dipyramids.
| Triangular bifrustum | Square bifrustum | Pentagonal bifrustum |
|---|---|---|
 |
 |
 |
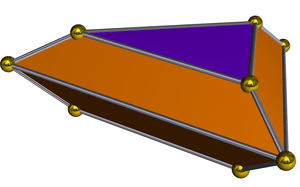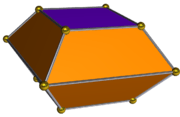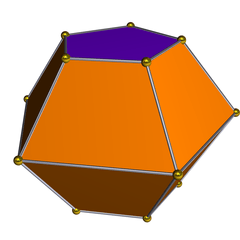| 6 trapezoids, 2 triangles. Dual to elongated triangular bipyramid, J14 | 8 trapezoids, 2 squares. Dual to elongated square bipyramid, J15 | 10 trapezoids, 2 pentagons. Dual to elongated pentagonal bipyramid, J16 |
gollark: I could just make a server-side infipage project.
gollark: I mean, apart from the meta description tags.
gollark: osmarks.tk is not really designed with SEO in mind.
gollark: <@543131534685765673> Everyone would post that everywhere.
gollark: The issue is just that most of the code runs on the client side.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.