5-5 duoprism
In geometry of 4 dimensions, a 5-5 duoprism or pentagonal duoprism is a polygonal duoprism, a 4-polytope resulting from the Cartesian product of two pentagons.
Uniform 5-5 duoprism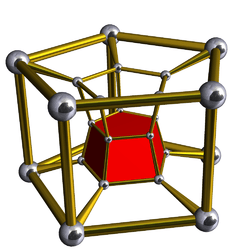 Schlegel diagram | |
|---|---|
| Type | Uniform duoprism |
| Schläfli symbol | {5}×{5} = {5}2 |
| Coxeter diagram | |
| Cells | 10 pentagonal prisms |
| Faces | 25 squares, 10 pentagons |
| Edges | 50 |
| Vertices | 25 |
| Vertex figure |  Tetragonal disphenoid |
| Symmetry | [[5,2,5]] = [10,2+,10], order 200 |
| Dual | 5-5 duopyramid |
| Properties | convex, vertex-uniform, facet-transitive |
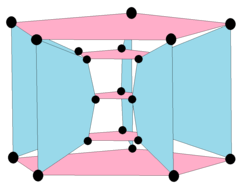
It has 25 vertices, 50 edges, 35 faces (25 squares, and 10 pentagons), in 10 pentagonal prism cells. It has Coxeter diagram ![]()
![]()
![]()
![]()
![]()
![]()
![]()
Images
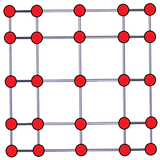 Orthogonal projection |
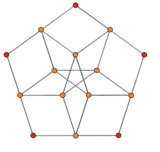 Orthogonal projection |
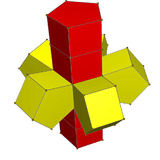 Net |
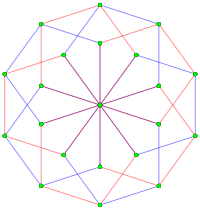
Seen in a skew 2D orthogonal projection, 20 of the vertices are in two decagonal rings, while 5 project into the center. The 5-5 duoprism here has an identical 2D projective appearance to the 3D rhombic triacontahedron. In this projection, the square faces project into wide and narrow rhombi seen in penrose tiling.
 |
 |
 |
| 5-5 duoprism | Penrose tiling | |
|---|---|---|
Related complex polygons
The regular complex polytope 5{4}2, ![]()
![]()
![]()
![]()
![]()
![]()
 Perspective projection of complex polygon, 5{4}2 has 25 vertices and 10 5-edges, shown here with 5 red and 5 blue pentagonal 5-edges. |
 Orthogonal projection with coinciding central vertices |
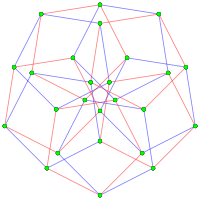 Orthogonal projection, perspective offset to avoid overlapping elements |
Related honeycombs and polytopes
The birectified order-5 120-cell, ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
5-5 duopyramid
| 5-5 duopyramid | |
|---|---|
| Type | Uniform dual duopyramid |
| Schläfli symbol | {5}+{5} = 2{5} |
| Coxeter diagram | |
| Cells | 25 tetragonal disphenoids |
| Faces | 50 isosceles triangles |
| Edges | 35 (25+10) |
| Vertices | 10 (5+5) |
| Symmetry | [[5,2,5]] = [10,2+,10], order 200 |
| Dual | 5-5 duoprism |
| Properties | convex, vertex-uniform, facet-transitive |
The dual of a 5-5 duoprism is called a 5-5 duopyramid or pentagonal duopyramid. It has 25 tetragonal disphenoid cells, 50 triangular faces, 35 edges, and 10 vertices.
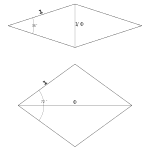
It can be seen in orthogonal projection as a regular 10-gon circle of vertices, divided into two pentagons, seen with colored vertices and edges:
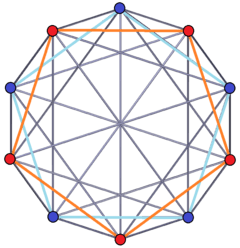 Two pentagons in dual positions |
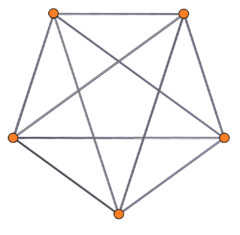 Two pentagons overlapping |
Related complex polygon
The regular complex polygon 2{4}5 has 10 vertices in with a real represention in matching the same vertex arrangement of the 5-5 duopyramid. It has 25 2-edges corresponding to the connecting edges of the 5-5 duopyramid, while the 10 edges connecting the two pentagons are not included. The vertices and edges makes a complete bipartite graph with each vertex from one pentagon is connected to every vertex on the other.[2]
 Orthographic projection |
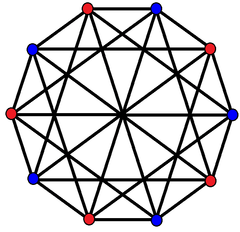 The 2{4}5 with 10 vertices in blue and red connected by 25 2-edges as a complete bipartite graph. |
See also
- 3-3 duoprism
- 3-4 duoprism
- Tesseract (4-4 duoprism)
- Convex regular 4-polytope
- Duocylinder
Notes
- Coxeter, H. S. M.; Regular Complex Polytopes, Cambridge University Press, (1974).
- Regular Complex Polytopes, p.114
References
- Regular Polytopes, H. S. M. Coxeter, Dover Publications, Inc., 1973, New York, p. 124.
- Coxeter, The Beauty of Geometry: Twelve Essays, Dover Publications, 1999, ISBN 0-486-40919-8 (Chapter 5: Regular Skew Polyhedra in three and four dimensions and their topological analogues)
- Coxeter, H. S. M. Regular Skew Polyhedra in Three and Four Dimensions. Proc. London Math. Soc. 43, 33-62, 1937.
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 26)
- Norman Johnson Uniform Polytopes, Manuscript (1991)
- N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D. Dissertation, University of Toronto, 1966
- Catalogue of Convex Polychora, section 6, George Olshevsky.
External links
- The Fourth Dimension Simply Explained—describes duoprisms as "double prisms" and duocylinders as "double cylinders"
- Polygloss - glossary of higher-dimensional terms
- Exploring Hyperspace with the Geometric Product