Reversible process (thermodynamics)
In thermodynamics, a reversible process is a process whose direction can be returned to its original position by inducing infinitesimal changes to some property of the system via its surroundings.[1] Throughout the entire reversible process, the system is in thermodynamic equilibrium with its surroundings. Having been reversed, it leaves no change in either the system or the surroundings. Since it would take an infinite amount of time for the reversible process to finish, perfectly reversible processes are impossible. However, if the system undergoing the changes responds much faster than the applied change, the deviation from reversibility may be negligible. In a reversible cycle, a cyclical reversible process, the system and its surroundings will be returned to their original states if one half cycle is followed by the other half cycle.[2]
| Thermodynamics | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
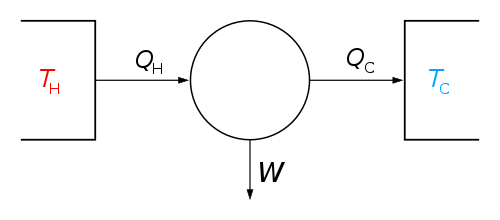 The classical Carnot heat engine | ||||||||||||
|
||||||||||||
| ||||||||||||
Thermodynamic processes can be carried out in one of two ways: reversibly or irreversibly. Reversibility means the reaction operates continuously at quasiequilibrium. In an ideal thermodynamically reversible process, the energy from work performed by or on the system would be maximized, and that from heat would be zero. However, heat cannot fully be converted to work and will always be lost to some degree (to the surroundings). (This is true only in case of a cycle. In case of an ideal process, heat can be completely converted into work, e.g., isothermal expansion of an ideal gas in a piston–cylinder arrangement.) The phenomenon of maximized work and minimized heat can be visualized on a pressure–volume graph as the area beneath the equilibrium curve, representing work done. In order to maximize work, one must follow the equilibrium curve precisely.
Irreversible processes, on the other hand, are a result of straying away from the curve, therefore decreasing the amount of overall work done; an irreversible process can be described as a thermodynamic process that departs from equilibrium. Irreversibility is defined as the difference between the reversible work and the actual work for a process. When described in terms of pressure and volume, it occurs when the pressure (or the volume) of a system changes so dramatically and instantaneously that the volume (or the pressure) does not have time to reach equilibrium. A classic example of irreversibility is allowing a certain volume of gas to be released into a vacuum. By releasing pressure on a sample and thus allowing it to occupy a large space, the system and surroundings are not in equilibrium during the expansion process and there is little work done. However, significant work will be required, with a corresponding amount of energy dissipated as heat flow to the environment, in order to reverse the process (compressing the gas back to its original volume and temperature).[3]
An alternative definition of a reversible process is a process that, after it has taken place, can be reversed and, when reversed, returns the system and its surroundings to their initial states. In thermodynamic terms, a process "taking place" would refer to its transition from one state to another.. ....
Irreversibility
In an irreversible process, finite changes are made; therefore the system is not at equilibrium throughout the process. At the same point in an irreversible cycle, the system will be in the same state, but the surroundings are permanently changed after each cycle.[2] It is the difference between the reversible work and the actual work for a process as shown in the following equation : I = W rev - W a

Boundaries and states
A reversible process changes the state of a system in such a way that the net change in the combined entropy of the system and its surroundings is zero. Reversible processes define the boundaries of how efficient heat engines can be in thermodynamics and engineering: a reversible process is one where no heat is lost from the system as "waste", and the machine is thus as efficient as it can possibly be (see Carnot cycle).
In some cases, it is important to distinguish between reversible and quasistatic processes. Reversible processes are always quasistatic, but the converse is not always true.[1] For example, an infinitesimal compression of a gas in a cylinder where there exists friction between the piston and the cylinder is a quasistatic, but not reversible process.[4] Although the system has been driven from its equilibrium state by only an infinitesimal amount, heat has been irreversibly lost due to friction, and cannot be recovered by simply moving the piston infinitesimally in the opposite direction.
Engineering archaisms
Historically, the term Tesla principle was used to describe (amongst other things) certain reversible processes invented by Nikola Tesla.[5] However, this phrase is no longer in conventional use. The principle stated that some systems could be reversed and operated in a complementary manner. It was developed during Tesla's research in alternating currents where the current's magnitude and direction varied cyclically. During a demonstration of the Tesla turbine, the disks revolved and machinery fastened to the shaft was operated by the engine. If the turbine's operation was reversed, the disks acted as a pump.[6]
See also
References
- Sears, F.W. and Salinger, G.L. (1986), Thermodynamics, Kinetic Theory, and Statistical Thermodynamics, 3rd edition (Addison-Wesley.)
- Zumdahl, Steven S. (2005) "10.2 The Isothermal Expansion and Compression of an Ideal Gas." Chemical Principles. 5th Edition. (Houghton Mifflin Company)
- Lower, S. (2003) Entropy Rules! What is Entropy? Entropy
- Giancoli, D.C. (2000), Physics for Scientists and Engineers (with Modern Physics), 3rd edition (Prentice-Hall.)
- Electrical Experimenter, January 1919. p. 615.
- "Tesla's New Monarch of Machines". The New York Herald Tribune. Tesla Engine Builders Association. Oct 15, 1911. Archived from the original on September 28, 2011.