Octahedral cupola
In 4-dimensional geometry, the octahedral cupola is a 4-polytope bounded by one octahedron and a parallel rhombicuboctahedron, connected by 20 triangular prisms, and 6 square pyramids.[1]
| Octahedral cupola | ||
|---|---|---|
 Schlegel diagram | ||
| Type | Polyhedral cupola | |
| Schläfli symbol | {3,4} v rr{3,4} | |
| Cells | 28 | 1 {3,4} 1 rr{4,3} 8+12 {}×{3} 6 {}v{4} |
| Faces | 82 | 40 triangles 42 squares |
| Edges | 84 | |
| Vertices | 30 | |
| Dual | ||
| Symmetry group | [4,3,1], order 48 | |
| Properties | convex, regular-faced | |
Related polytopes
The octahedral cupola can be sliced off from a runcinated 24-cell, on a hyperplane parallel to an octahedral cell. The cupola can be seen in a B2 and B3 Coxeter plane orthogonal projection of the runcinated 24-cell:
| Runcinated 24-cell | Octahedron (cupola top) |
Rhombicuboctahedron (cupola base) |
|---|---|---|
| B3 Coxeter plane | ||
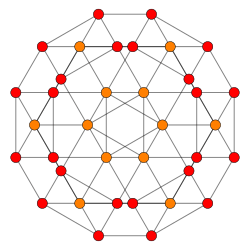 |
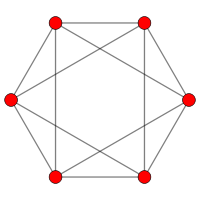 |
 |
| B2 Coxeter plane | ||
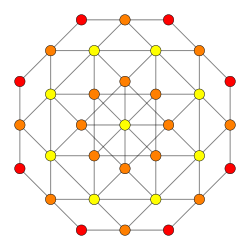 |
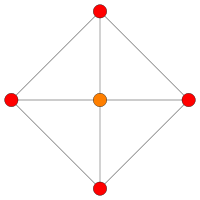 |
 |
gollark: I assume your alts within it have already found out about this.
gollark: Esoserver currently operates a #suggestions channel into which, presumably, suggestions go and are discussed a bit. This seems to work okay without enforcing a particular style of discussion, although most bulk metadiscussion just goes in <#348702212110680064>. We haven't really had any large-scale debates happening, so who knows.
gollark: Which is still more annoying for the writer. So I'm not sure why you would expect people to interpret it as an amazing gift.
gollark: People have different preferences on communication.
gollark: "It has no flaws, since it doesn't have flaws"?
See also
- Octahedral pyramid
- Cubic cupola
- Runcinated 24-cell
References
- Convex Segmentochora Dr. Richard Klitzing, Symmetry: Culture and Science, Vol. 11, Nos. 1-4, 139-181, 2000 (4.107 octahedron || rhombicuboctahedron)
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.