Cubic cupola
In 4-dimensional geometry, the cubic cupola is a 4-polytope bounded by a rhombicuboctahedron, a parallel cube, connected by 6 square prisms, 12 triangular prisms, 8 triangular pyramids.[1]
| Cubic cupola | ||
|---|---|---|
 Schlegel diagram | ||
| Type | Polyhedral cupola | |
| Schläfli symbol | {4,3} v rr{4,3} | |
| Cells | 28 | 1 rr{4,3} 1+6 {4,3} 12 {}×{3} 8 {3,3} |
| Faces | 80 | 32 triangles 48 squares |
| Edges | 84 | |
| Vertices | 32 | |
| Dual | ||
| Symmetry group | [4,3,1], order 48 | |
| Properties | convex, regular-faced | |
Related polytopes
The cubic cupola can be sliced off from a runcinated tesseract, on a hyperplane parallel to cubic cell. The cupola can be seen in an edge-centered (B3) orthogonal projection of the runcinated tesseract:
| Runcinated tesseract | Cube (cupola top) |
Rhombicuboctahedron (cupola base) |
|---|---|---|
| B2 Coxeter plane | ||
 |
 |
 |
| B3 Coxeter plane | ||
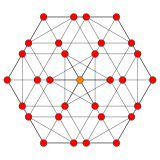 |
 |
 |
gollark: But you get this atrocious misuse of it.
gollark: You have a tree of nodes which need attributes *and* inner contents.
gollark: For HTML, which is technically not XML but similar, it's somewhat sensible.
gollark: Also that it's just bad for most of its uses.
gollark: And XML is just no.
See also
- Cubic pyramid
- Octahedral cupola
- Runcinated tesseract
References
- Convex Segmentochora Dr. Richard Klitzing, Symmetry: Culture and Science, Vol. 11, Nos. 1-4, 139-181, 2000 (4.71 cube || rhombicuboctahedron)
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.