Equiangular polygon

In Euclidean geometry, an equiangular polygon is a polygon whose vertex angles are equal. If the lengths of the sides are also equal (that is, if it is also equilateral) then it is a regular polygon. Isogonal polygons are equiangular polygons which alternate two edge lengths.

A rectangle is an equiangular quadrilateral
A convex equiangular octagon with 4-fold reflective symmetry
A nonconvex equiangular hexagon with 3-fold reflectional symmetry

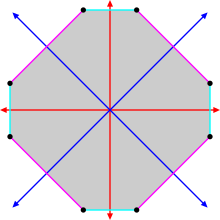
Properties

The only equiangular triangle is the equilateral triangle. Rectangles, including the square, are the only equiangular quadrilaterals (four-sided figures).[1]

For a convex equiangular n-gon each internal angle is 180(1-2/n)°; this is the equiangular polygon theorem.

Viviani's theorem holds for equiangular polygons:[2]

The sum of distances from an interior point to the sides of an equiangular polygon does not depend on the location of the point, and is that polygon's invariant.

A rectangle (equiangular quadrilateral) with integer side lengths may be tiled by unit squares, and an equiangular hexagon with integer side lengths may be tiled by unit equilateral triangles. Some but not all equilateral dodecagons may be tiled by a combination of unit squares and equilateral triangles; the rest may be tiled by these two shapes together with rhombi with 30 and 150 degree angles.[1]

A cyclic polygon is equiangular if and only if the alternate sides are equal (that is, sides 1, 3, 5, ... are equal and sides 2, 4, ... are equal). Thus if n is odd, a cyclic polygon is equiangular if and only if it is regular.[3]

For prime p, every integer-sided equiangular p-gon is regular. Moreover, every integer-sided equiangular pk-gon has p-fold rotational symmetry.[4]

An ordered set of side lengths gives rise to an equiangular n-gon if and only if either of two equivalent conditions holds for the polynomial it equals zero at the complex value it is divisible by [5]

gollark: I think it's more significantly dependent on interest and such.
gollark: You should probably filter by relevant skill or whatever directly instead of things vaguely correlated with those.
gollark: BEE YOU
gollark: What? No. 10% of people not fitting or whatever it is doesn't make there not be a large amount more who do.
gollark: Thus, go learn about all of history and check.

References
  1. Ball, Derek (2002), "Equiangular polygons", The Mathematical Gazette, 86 (507): 396–407, JSTOR 3621131.
  2. Elias Abboud "On Viviani’s Theorem and its Extensions" pp. 2, 11
  3. De Villiers, Michael, "Equiangular cyclic and equilateral circumscribed polygons", Mathematical Gazette 95, March 2011, 102-107.
  4. McLean, K. Robin. "A powerful algebraic tool for equiangular polygons", Mathematical Gazette 88, November 2004, 513-514.
  5. M. Bras-Amorós, M. Pujol: "Side Lengths of Equiangular Polygons (as seen by a coding theorist)", The American Mathematical Monthly, vol. 122, n. 5, pp. 476–478, May 2015. ISSN 0002-9890.
  • Williams, R. The Geometrical Foundation of Natural Structure: A Source Book of Design. New York: Dover Publications, 1979. p. 32
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.