Compact stencil
In mathematics, especially in the areas of numerical analysis called numerical partial differential equations, a compact stencil is a type of stencil that uses only nine nodes for its discretization method in two dimensions. It uses only the center node and the adjacent nodes. For any structured grid utilizing a compact stencil in 1, 2, or 3 dimensions the maximum number of nodes is 3, 9, or 27 respectively. Compact stencils may be compared to non-compact stencils. Compact stencils are currently implemented in many partial differential equation solvers, including several in the topics of CFD, FEA, and other mathematical solvers relating to PDE's.[1][2]
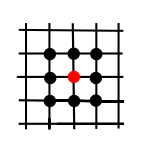
Two Point Stencil Example
The two point stencil for the first derivative of a function is given by:
.
This is obtained from the Taylor series expansion of the first derivative of the function given by:
.
Replacing with , we have:
.
Addition of the above two equations together results in the cancellation of the terms in odd powers of :
.
.
.
Three Point Stencil Example
For example, the three point stencil for the second derivative of a function is given by:
.
This is obtained from the Taylor series expansion of the first derivative of the function given by:
.
Replacing with , we have:
.
Subtraction of the above two equations results in the cancellation of the terms in even powers of :
.
.
.
References
- W. F. Spotz. High-Order Compact Finite Difference Schemes for Computational Mechanics. PhD thesis, University of Texas at Austin, Austin, TX, 1995.
- Communications in Numerical Methods in Engineering, Copyright © 2008 John Wiley & Sons, Ltd.