Apsis
Apsis (Greek: ἀψίς; plural apsides /ˈæpsɪdiːz/, Greek: ἀψῖδες; "orbit") denotes either of the two extreme points (i.e., the farthest or nearest point) in the orbit of a planetary body about its primary body (or simply, "the primary"). The plural term, "apsides," usually implies both apsis points (i.e., farthest and nearest); apsides can also refer to the distance of the extreme range of an object orbiting a host body. For example, the apsides of Earth's orbit of the Sun are two: the apsis for Earth's farthest point from the Sun, dubbed the aphelion; and the apsis for Earth's nearest point, the perihelion (see top figure). (The term "apsis", a cognate with apse, comes via Latin from Greek).[1]
.png)
*The line of apsides is the line connecting positions 1 and 2.
*The table names the (two) apsides of a planetary body (X, "orbiter") orbiting the host body indicated:
| (1) farthest | (X) orbiter | (3) host | (2) nearest |
|---|---|---|---|
| apogee | Moon | Earth | perigee |
| apojove | Ganymede | Jupiter | perijove |
| aphelion | Earth | Sun | perihelion |
| aphelion | Jupiter | Sun | perihelion |
| aphelion | Halley's Comet | Sun | perihelion |
| apastron | exoplanet | star | periastron |
| apocenter | comet, e.g. | primary | pericenter |
| apoapsis | comet, e.g. | primary | periapsis |
For example, the Moon's two apsides are the farthest point, apogee, and the nearest point, perigee, of its orbit around the host Earth. The Earth's two apsides are the farthest point, aphelion, and the nearest point, perihelion, of its orbit around the host Sun. The terms aphelion and perihelion apply in the same way to the orbits of Jupiter and the other planets, the comets, and the asteroids of the Solar System.
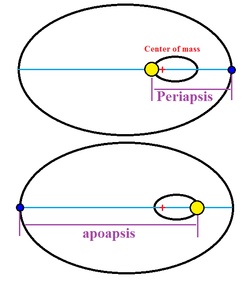
∗Periapsis and apoapsis as distances: The smallest and largest distances between the orbiter and its host body.
| Part of a series on |
| Astrodynamics |
|---|
 |
|
Orbital parameters |
|
Gravitational influences |
|
Preflight engineering |
|
Efficiency measures |
There are two apsides in any elliptic orbit. Each is named by selecting the appropriate prefix: ap-, apo- (from ἀπ(ό), (ap(o)-), meaning 'away from'), or peri- (from περί (peri-), meaning 'near')—then joining it to the reference suffix of the "host" body being orbited. (For example, the reference suffix for Earth is -gee, hence apogee and perigee are the names of the apsides for the Moon, and any other artificial satellites of the Earth. The suffix for the Sun is -helion, hence aphelion and perihelion are the names of the apsides for the Earth and for the Sun's other planets, comets, asteroids, etc., (see table, top figure).)
According to Newton's laws of motion all periodic orbits are ellipses, including: 1) the single orbital ellipse, where the primary body is fixed at one focus point and the planetary body orbits around that focus (see top figure); and 2) the two-body system of interacting elliptic orbits: both bodies orbit their joint center of mass (or barycenter), which is located at a focus point that is common to both ellipses, (see second figure). For such a two-body system, when one mass is sufficiently larger than the other, the smaller ellipse (of the larger body) around the barycenter comprises one of the orbital elements of the larger ellipse (of the smaller body).
The barycenter of the two bodies may lie well within the bigger body—e.g., the Earth–Moon barycenter is about 75% of the way from Earth's center to its surface. If, compared to the larger mass, the smaller mass is negligible (e.g., for satellites), then the orbital parameters are independent of the smaller mass.
When used as a suffix—that is, -apsis—the term can refer to the two distances from the primary body to the orbiting body when the latter is located: 1) at the periapsis point, or 2) at the apoapsis point (compare both graphics, second figure). The line of apsides denotes the distance of the line that joins the nearest and farthest points across an orbit; it also refers simply to the extreme range of an object orbiting a host body (see top figure; see third figure).
In orbital mechanics, the apsides technically refer to the distance measured between the barycenters of the central body and orbiting body. However, in the case of a spacecraft, the terms are commonly used to refer to the orbital altitude of the spacecraft above the surface of the central body (assuming a constant, standard reference radius).
Terminology
The words "pericenter" and "apocenter" are often seen, although periapsis/apoapsis are preferred in technical usage.
- For generic situations where the primary is not specified, the terms pericenter and apocenter are used for naming the extreme points of orbits (see table, top figure); periapsis and apoapsis (or apapsis) are equivalent alternatives, but these terms also frequently refer to distances—that is, the smallest and largest distances between the orbiter and its host body, (see second figure).
- For a body orbiting the Sun, the point of least distance is the perihelion (/ˌpɛrɪˈhiːliən/), and the point of greatest distance is the aphelion (/æpˈhiːliən/);[2] when discussing orbits around other stars the terms become periastron and apastron.
- When discussing a satellite of Earth, including the Moon, the point of least distance is the perigee (/ˈpɛrɪdʒiː/), and of greatest distance, the apogee, (from Ancient Greek: Γῆ (Gē), "land" or "earth").
- There are no natural satellites of the Moon. For man-made objects in lunar orbit, the point of least distance may be called the pericynthion (/ˌpɛrɪˈsɪnθiən/) and the greatest distance the apocynthion (/ˌæpəˈsɪnθiən/); or perilune and apolune are sometimes used.[3]
Etymology
The words perihelion and aphelion were coined by Johannes Kepler[4] to describe the orbital motions of the planets around the Sun. The words are formed from the prefixes peri- (Greek: περί, near) and apo- (Greek: ἀπό, away from), affixed to the Greek word for the sun, (ἥλιος, or hēlíou).[5]
Various related terms are used for other celestial objects. The suffixes -gee, -helion, -astron and -galacticon are frequently used in the astronomical literature when referring to the Earth, Sun, stars, and the galactic center respectively. The suffix -jove is occasionally used for Jupiter, but -saturnium has very rarely been used in the last 50 years for Saturn. The -gee form is also used as a generic closest-approach-to "any planet" term—instead of applying it only to Earth.
During the Apollo program, the terms pericynthion and apocynthion were used when referring to orbiting the Moon; they reference Cynthia, an alternative name for the Greek Moon goddess Artemis.[6] Regarding black holes, the terms perimelasma and apomelasma (from a Greek root) were used by physicist and science-fiction author Geoffrey A. Landis in a 1998 story;[7] which occurred before perinigricon and aponigricon (from Latin) appeared in the scientific literature in 2002,[8] and before peribothron (from Greek bothros, meaning hole or pit) in 2015.[9]
Terminology summary
The suffixes shown below may be added to prefixes peri- or apo- to form unique names of apsides for the orbiting bodies of the indicated host/(primary) system. However, only for the Earth and Sun systems are the unique suffixes commonly used. Typically, for other host systems the generic suffix, -apsis, is used instead.[10]
| Astronomical host object | Sun | Mercury | Venus | Earth | Moon | Mars | Ceres | Jupiter | Saturn |
|---|---|---|---|---|---|---|---|---|---|
| Suffix | ‑helion | ‑hermion | ‑cythe | ‑gee | ‑lune[3] ‑cynthion ‑selene[3] |
‑areion | ‑demeter[11] | ‑jove | ‑chron[3] ‑kronos ‑saturnium ‑krone[12] |
| Origin of the name |
Helios | Hermes | Cytherean | Gaia | Luna Cynthia Selene |
Ares | Demeter | Zeus Jupiter |
Cronos Saturn |
| Astronomical host object |
Star | Galaxy | Barycenter | Black hole |
|---|---|---|---|---|
| Suffix | ‑astron | ‑galacticon | ‑center ‑focus ‑apsis |
‑melasma ‑bothron ‑nigricon |
| Origin of the name |
Lat: astra; stars | Gr: galaxias; galaxy | Gr: melos; black Gr: bothros; hole Lat: niger; black |
Perihelion and aphelion
The perihelion (q) and aphelion (Q) are the nearest and farthest points respectively of a body's direct orbit around the Sun.
Comparing osculating elements at a specific epoch to effectively those at a different epoch will generate differences. The time-of-perihelion-passage as one of six osculating elements is not an exact prediction (other than for a generic 2-body model) of the actual minimum distance to the Sun using the full dynamical model. Precise predictions of perihelion passage require numerical integration.
Inner planets and outer planets
The image below-left features the inner planets: their orbits, orbital nodes, and the points of perihelion (green dot) and aphelion (red dot), as seen from above Earth's northern pole and Earth's ecliptic plane, which is coplanar with Earth's orbital plane. From this orientation, the planets are situated outward from the Sun as Mercury, Venus, Earth, and Mars, with all planets travelling their orbits counterclockwise around the Sun. The reference Earth-orbit is colored yellow and represents the orbital plane of reference. For Mercury, Venus, and Mars, the section of orbit tilted above the plane of reference is here shaded blue; the section below the plane is shaded violet/pink.
The image below-right shows the outer planets: the orbits, orbital nodes, and the points of perihelion (green dot) and aphelion (red dot) of Jupiter, Saturn, Uranus, and Neptune—as seen from above the reference orbital plane, all travelling their orbits counterclockwise. For each planet the section of orbit tilted above the reference orbital plane is colored blue; the section below the plane is violet/pink.
The two orbital nodes are the two end points of the "line of nodes" where a tilted orbit intersects the plane of reference;[13] here they may be 'seen' where the blue section of an orbit becomes violet/pink.
The two images below show the positions of perihelion (q) and the aphelion (Q) in the orbits of the planets of the Solar System.[1]
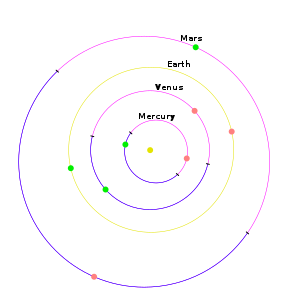 The perihelion and aphelion points of the inner planets of the Solar System
The perihelion and aphelion points of the inner planets of the Solar System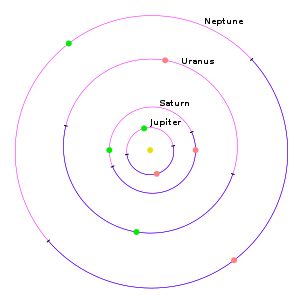 The perihelion and aphelion points of the outer planets of the Solar System
The perihelion and aphelion points of the outer planets of the Solar System
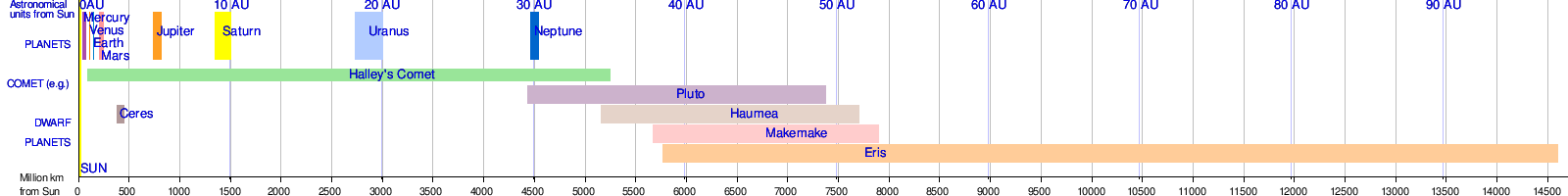
Lines of apsides
The chart shows the extreme range—from the closest approach (perihelion) to farthest point (aphelion)—of several orbiting celestial bodies of the Solar System: the planets, the known dwarf planets, including Ceres, and Halley's Comet. The length of the horizontal bars correspond to the extreme range of the orbit of the indicated body around the Sun. These extreme distances (between perihelion and aphelion) are the lines of apsides of the orbits of various objects around a host body.
Earth perihelion and aphelion
Currently, the Earth reaches perihelion in early January, approximately 14 days after the December solstice. At perihelion, the Earth's center is about 0.98329 astronomical units (AU) or 147,098,070 km (91,402,500 mi) from the Sun's center. In contrast, the Earth reaches aphelion currently in early July, approximately 14 days after the June solstice. The aphelion distance between the Earth's and Sun's centers is currently about 1.01671 AU or 152,097,700 km (94,509,100 mi).
The dates of perihelion and aphelion change over time due to precession and other orbital factors, which follow cyclical patterns known as Milankovitch cycles. In the short term, such dates can vary up to 2 days from one year to another.[14] This significant variation is due to the presence of the Moon: while the Earth–Moon barycenter is moving on a stable orbit around the Sun, the position of the Earth's center which is on average about 4,700 kilometres (2,900 mi) from the barycenter, could be shifted in any direction from it—and this affects the timing of the actual closest approach between the Sun's and the Earth's centers (which in turn defines the timing of perihelion in a given year).[15]
Because of the increased distance at aphelion, only 93.55% of the radiation from the Sun falls on a given area of Earth's surface as does at perihelion, but this does not account for the seasons, which result instead from the tilt of Earth's axis of 23.4° away from perpendicular to the plane of Earth's orbit.[16] Indeed, at both perihelion and aphelion it is summer in one hemisphere while it is winter in the other one. Winter falls on the hemisphere where sunlight strikes least directly, and summer falls where sunlight strikes most directly, regardless of the Earth's distance from the Sun.
In the northern hemisphere, summer occurs at the same time as aphelion, when solar radiation is lowest. Despite this, summers in the northern hemisphere are on average 2.3 °C (4 °F) warmer than in the southern hemisphere, because the northern hemisphere contains larger land masses, which are easier to heat than the seas.[17]
Perihelion and aphelion do however have an indirect effect on the seasons: because Earth's orbital speed is minimum at aphelion and maximum at perihelion, the planet takes longer to orbit from June solstice to September equinox than it does from December solstice to March equinox. Therefore, summers in the northern hemisphere last longer than summers in the southern hemisphere by 92 days versus 89.[18]
Astronomers commonly express the timing of perihelion relative to the First Point of Aries not in terms of days and hours, but rather as an angle of orbital displacement, the so-called longitude of the periapsis (also called longitude of the pericenter). For the orbit of the Earth, this is called the longitude of perihelion, and in 2000 it was about 282.895°; by the year 2010, this had advanced by a small fraction of a degree to about 283.067°.[19]
For the orbit of the Earth around the Sun, the time of apsis is often expressed in terms of a time relative to seasons, since this determines the contribution of the elliptical orbit to seasonal variations. The variation of the seasons is primarily controlled by the annual cycle of the elevation angle of the Sun, which is a result of the tilt of the axis of the Earth measured from the plane of the ecliptic. The Earth's eccentricity and other orbital elements are not constant, but vary slowly due to the perturbing effects of the planets and other objects in the solar system (Milankovitch cycles).
On a very long time scale, the dates of the perihelion and of the aphelion progress through the seasons, and they make one complete cycle in 22,000 to 26,000 years. There is a corresponding movement of the position of the stars as seen from Earth that is called the apsidal precession. (This is closely related to the precession of the axes.) The dates and times of the perihelions and aphelions for several past and future years are listed in the following table:[20]
| Year | Perihelion | Aphelion | ||
|---|---|---|---|---|
| Date | Time (UT) | Date | Time (UT) | |
| 2010 | January 3 | 00:09 | July 6 | 11:30 |
| 2011 | January 3 | 18:32 | July 4 | 14:54 |
| 2012 | January 5 | 00:32 | July 5 | 03:32 |
| 2013 | January 2 | 04:38 | July 5 | 14:44 |
| 2014 | January 4 | 11:59 | July 4 | 00:13 |
| 2015 | January 4 | 06:36 | July 6 | 19:40 |
| 2016 | January 2 | 22:49 | July 4 | 16:24 |
| 2017 | January 4 | 14:18 | July 3 | 20:11 |
| 2018 | January 3 | 05:35 | July 6 | 16:47 |
| 2019 | January 3 | 05:20 | July 4 | 22:11 |
| 2020 | January 5 | 07:48 | July 4 | 11:35 |
| 2021 | January 2 | 13:51 | July 5 | 22:27 |
| 2022 | January 4 | 06:55 | July 4 | 07:11 |
| 2023 | January 4 | 16:17 | July 6 | 20:07 |
| 2024 | January 3 | 00:39 | July 5 | 05:06 |
| 2025 | January 4 | 13:28 | July 3 | 19:55 |
| 2026 | January 3 | 17:16 | July 6 | 17:31 |
| 2027 | January 3 | 02:33 | July 5 | 05:06 |
| 2028 | January 5 | 12:28 | July 3 | 22:18 |
| 2029 | January 2 | 18:13 | July 6 | 05:12 |
Other planets
The following table shows the distances of the planets and dwarf planets from the Sun at their perihelion and aphelion.[21]
| Type of body | Body | Distance from Sun at perihelion | Distance from Sun at aphelion |
|---|---|---|---|
| Planet | Mercury | 46,001,009 km (28,583,702 mi) | 69,817,445 km (43,382,549 mi) |
| Venus | 107,476,170 km (66,782,600 mi) | 108,942,780 km (67,693,910 mi) | |
| Earth | 147,098,291 km (91,402,640 mi) | 152,098,233 km (94,509,460 mi) | |
| Mars | 206,655,215 km (128,409,597 mi) | 249,232,432 km (154,865,853 mi) | |
| Jupiter | 740,679,835 km (460,237,112 mi) | 816,001,807 km (507,040,016 mi) | |
| Saturn | 1,349,823,615 km (838,741,509 mi) | 1,503,509,229 km (934,237,322 mi) | |
| Uranus | 2,734,998,229 km (1.699449110×109 mi) | 3,006,318,143 km (1.868039489×109 mi) | |
| Neptune | 4,459,753,056 km (2.771162073×109 mi) | 4,537,039,826 km (2.819185846×109 mi) | |
| Dwarf planet | Ceres | 380,951,528 km (236,712,305 mi) | 446,428,973 km (277,398,103 mi) |
| Pluto | 4,436,756,954 km (2.756872958×109 mi) | 7,376,124,302 km (4.583311152×109 mi) | |
| Haumea | 5,157,623,774 km (3.204798834×109 mi) | 7,706,399,149 km (4.788534427×109 mi) | |
| Makemake | 5,671,928,586 km (3.524373028×109 mi) | 7,894,762,625 km (4.905578065×109 mi) | |
| Eris | 5,765,732,799 km (3.582660263×109 mi) | 14,594,512,904 km (9.068609883×109 mi) |
Mathematical formulae
These formulae characterize the pericenter and apocenter of an orbit:
- Pericenter
- Maximum speed, , at minimum (pericenter) distance, .
- Apocenter
- Minimum speed, , at maximum (apocenter) distance, .
While, in accordance with Kepler's laws of planetary motion (based on the conservation of angular momentum) and the conservation of energy, these two quantities are constant for a given orbit:
- Specific relative angular momentum
- Specific orbital energy
where:
- a is the semi-major axis:
- μ is the standard gravitational parameter
- e is the eccentricity, defined as
Note that for conversion from heights above the surface to distances between an orbit and its primary, the radius of the central body has to be added, and conversely.
The arithmetic mean of the two limiting distances is the length of the semi-major axis a. The geometric mean of the two distances is the length of the semi-minor axis b.
The geometric mean of the two limiting speeds is
which is the speed of a body in a circular orbit whose radius is .
Time of perihelion
Orbital elements such as the time of perihelion passage are defined at the epoch chosen using an unperturbed two-body solution. To get an accurate time of perihelion passage you need to use an epoch close to the perihelion passage. For example using an epoch of 1996, Comet Hale–Bopp shows perihelion on 1 April 1997.[22] Using an epoch of 2008 shows a less accurate perihelion date of 30 March 1997.[23] Short-period comets can be even more sensitive to the epoch selected. Using an epoch of 2005 shows 101P/Chernykh coming to perihelion on 25 December 2005,[24] but using an epoch of 2011 produces a less accurate unperturbed perihelion date of 10 January 2006.[25]
See also
References
- "the definition of apsis". Dictionary.com.
- Since the Sun, Ἥλιος in Greek, begins with a vowel (H is considered a vowel in Greek), the final o in "apo" is omitted from the prefix. =The pronunciation "Ap-helion" is given in many dictionaries , pronouncing the "p" and "h" in separate syllables. However, the pronunciation /əˈfiːliən/ is also common (e.g., McGraw Hill Dictionary of Scientific and Technical Terms, 5th edition, 1994, p. 114), since in late Greek, 'p' from ἀπό followed by the 'h' from ἥλιος becomes phi; thus, the Greek word is αφήλιον. (see, for example, Walker, John, A Key to the Classical Pronunciation of Greek, Latin, and Scripture Proper Names, Townsend Young 1859 , page 26.) Many dictionaries give both pronunciations
- "Basics of Space Flight". NASA. Retrieved May 30, 2017.
- Klein, Ernest, A Comprehensive Etymological Dictionary of the English Language, Elsevier, Amsterdam, 1965. (Archived version)
- Since the Sun, Ἥλιος in Greek, begins with a vowel, H is the long e vowel in Greek, the final o in "apo" is omitted from the prefix. The pronunciation "Ap-helion" is given in many dictionaries , pronouncing the "p" and "h" in separate syllables. However, the pronunciation /əˈfiːliən/ is also common (e.g., McGraw Hill Dictionary of Scientific and Technical Terms, 5th edition, 1994, p. 114), since in late Greek, 'p' from ἀπό followed by the 'h' from ἥλιος becomes phi; thus, the Greek word is αφήλιον. (see, for example, Walker, John, A Key to the Classical Pronunciation of Greek, Latin, and Scripture Proper Names, Townsend Young 1859 , page 26.) Many dictionaries give both pronunciations
- "Apollo 15 Mission Report". Glossary. Retrieved October 16, 2009.
- Perimelasma, by Geoffrey Landis, first published in Asimov's Science Fiction, January 1998, republished at Infinity Plus
- R. Schödel, T. Ott, R. Genzel, R. Hofmann, M. Lehnert, A. Eckart, N. Mouawad, T. Alexander, M. J. Reid, R. Lenzen, M. Hartung, F. Lacombe, D. Rouan, E. Gendron, G. Rousset, A.-M. Lagrange, W. Brandner, N. Ageorges, C. Lidman, A. F. M. Moorwood, J. Spyromilio, N. Hubin, K. M. Menten (October 17, 2002). "A star in a 15.2-year orbit around the supermassive black hole at the centre of the Milky Way". Nature. 419: 694–696. arXiv:astro-ph/0210426. Bibcode:2002Natur.419..694S. doi:10.1038/nature01121. PMID 12384690.CS1 maint: uses authors parameter (link)
- Koberlein, Brian (March 29, 2015). "Peribothron – Star makes closest approach to a black hole". briankoberlein.com. Retrieved January 10, 2018.
- "MAVEN » Science Orbit".
- "Dawn Journal: 11 Years in Space". www.planetary.org.
- Cecconi, B.; Lamy, L.; Zarka, P.; Prangé, R.; Kurth, W. S.; Louarn, P. (March 4, 2009). "Goniopolarimetric study of the revolution 29 perikrone using the Cassini Radio and Plasma Wave Science instrument high-frequency radio receiver". JGRA. 114 (A3): A03215. doi:10.1029/2008JA013830 – via ui.adsabs.harvard.edu.
- Darling, David. "line of nodes". The Encyclopedia of Astrobiology, Astronomy, and Spaceflight. Retrieved May 17, 2007.
- "Perihelion, Aphelion and the Solstices". timeanddate.com. Retrieved January 10, 2018.
- "Variation in Times of Perihelion and Aphelion". Astronomical Applications Department of the U.S. Naval Observatory. August 11, 2011. Retrieved January 10, 2018.
- "Solar System Exploration: Science & Technology: Science Features: Weather, Weather, Everywhere?". NASA. Retrieved September 19, 2015.
- "Earth at Aphelion". Space Weather. July 2008. Retrieved July 7, 2015.
- Rockport, Steve C. "How much does aphelion affect our weather? We're at aphelion in the summer. Would our summers be warmer if we were at perihelion, instead?". Planetarium. University of Southern Maine. Retrieved July 4, 2020.
- "Data.GISS: Earth's Orbital Parameters". data.giss.nasa.gov.
- Espenak, Fred. "Earth at Perihelion and Aphelion: 2001 to 2050". astropixels. Retrieved December 24, 2019.
- "NASA planetary comparison chart". Archived from the original on August 4, 2016. Retrieved August 4, 2016.
- JPL SBDB: Hale-Bopp (Epoch 1996)
- JPL SBDB: Hale-Bopp
- 101P/Chernykh - A (NK 1293) by Syuichi Nakano
- JPL SBDB: 101P/Chernykh
External links
| Look up apsis in Wiktionary, the free dictionary. |
- Apogee – Perigee Photographic Size Comparison, perseus.gr
- Aphelion – Perihelion Photographic Size Comparison, perseus.gr
- Earth's Seasons: Equinoxes, Solstices, Perihelion, and Aphelion, 2000–2020, usno.navy.mil
- Dates and times of Earth's perihelion and aphelion, 2000–2025 from the United States Naval Observatory
- List of asteroids currently closer to the Sun than Mercury (These objects will be close to perihelion)