Topology
Topology is a branch of mathematics dealing with the properties of space - whether it is contiguous, contains holes, and so on.
Cellular automata can be characterized in entirely topological terms, thus they can be studied within the scope of topology; see Cellular automaton § Generalizations and topological characterization.
A surface – e.g. the grid used to play the Game of Life – may be infinite, like a plane or a cylinder, or finite, like a sphere or a torus. A finite surface has to be curved to form a cylinder, (infinite in only one direction), torus or Klein bottle. The image shows how to "roll" and connect the sides in each case:
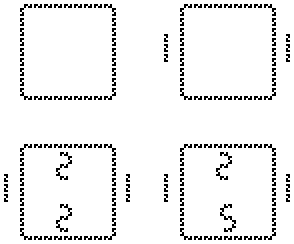
Topologies
- Infinite plane: default.
- Finite plane: cells outside of the plane are always considered to be dead.
- Cylinder: "rolling" the plane and connecting the opposite sides marked "1".
- Torus: "rolling" the cylinder and connecting the opposite circles marked "2".
- Klein bottle: "rolling" the cylinder, "twisting" it in the fourth dimension and connecting the opposite circles marked "2" and "S"; note that the "S" becomes a "2" after twisting.
- Cross-surface: like the Klein bottle, but "twisting" the opposite sides while creating the cylinder and then "twisting" the opposite circles when creating the cross-surface.
- Sphere: joining adjacent sides, rather than opposite sides as is done for the torus.
For how spaceships travel on certain planes, see Bounded grids.
This article is issued from Conwaylife. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.