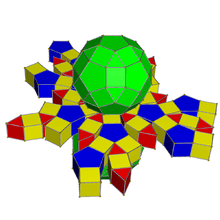
Rhombicosidodecahedral prism
In geometry, a rhombicosidodecahedral prism or small rhombicosidodecahedral prism is a convex uniform polychoron (four-dimensional polytope).
| Rhombicosidodecahedral prism | |
|---|---|
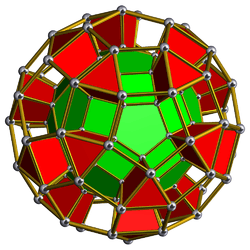 Schlegel diagram One rhombicosidodecahedron and triangular prisms show | |
| Type | Prismatic uniform polychoron |
| Uniform index | 61 |
| Schläfli symbol | t0,2,3{3,5,2} or rr{3,5}×{} |
| Coxeter-Dynkin |      |
| Cells | 64 total: 2 rr{5,3} 12 {}x{5} 20 {}x{3} 30 {4,3} |
| Faces | 244 total:40 {3} 180 {4} 24 {5} |
| Edges | 300 |
| Vertices | 120 |
| Vertex figure | 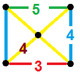 Trapezoidal pyramid |
| Symmetry group | [5,3,2], order 240 |
| Properties | convex |
It is one of 18 convex uniform polyhedral prisms created by using uniform prisms to connect pairs of Platonic solids or Archimedean solids in parallel hyperplanes.
Alternative names
- (small) rhombicosidodecahedral dyadic prism (Norman W. Johnson)
- Sriddip (Jonathan Bowers: for small-rhombicosidodecahedral prism)
- (small) rhombicosidodecahedral hyperprism
gollark: I made something to display a game of life grid ingame by using a command computer.
gollark: There are many mods adding ingame computers.
gollark: Command blocks are kind of annoying.
gollark: What?
gollark: Pretty awful, this being at home in the UK, but it works.
External links
- 6. Convex uniform prismatic polychora - Model 61, George Olshevsky.
- Klitzing, Richard. "4D uniform polytopes (polychora) x x3o5x - sriddip".
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.