Negative pedal curve

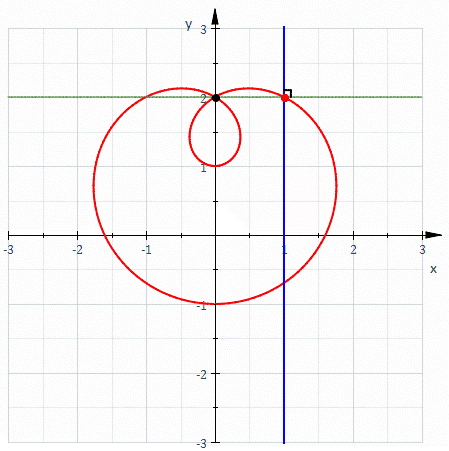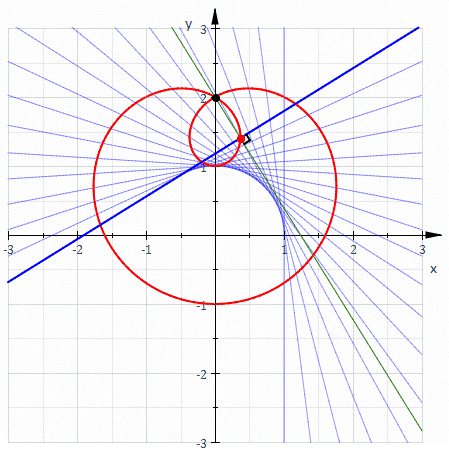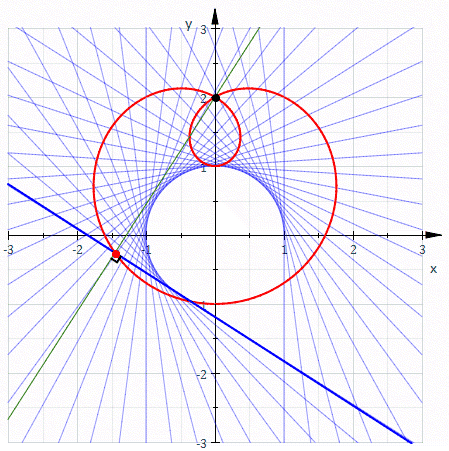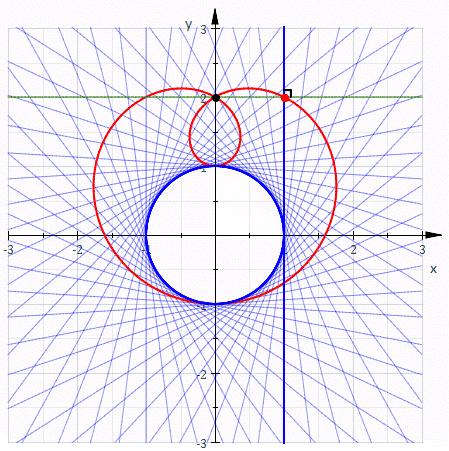
In geometry, a negative pedal curve is a plane curve that can be constructed from another plane curve C and a fixed point P on that curve. For each point X ≠ P on the curve C, the negative pedal curve has a tangent that passes through X and is perpendicular to line XP. Constructing the negative pedal curve is the inverse operation to constructing a pedal curve.

Circle — negative pedal curve of a limaçon

Definition

In the plane, for every point X other than P there is a unique line through X perpendicular to XP. For a given curve in the plane and a given fixed point P, called the pedal point, the negative pedal curve is the envelope of the lines XP for which X lies on the given curve.

Parameterization

For a parametrically defined curve, its negative pedal curve with pedal point (0; 0) is defined as

Properties

The negative pedal curve of a pedal curve with the same pedal point is the original curve.

gollark: Religion is like bees: extant.
gollark: No, since March there was a τ-class bee event. Now it is φ-class.
gollark: Hmm. Apparently the UK has experienced a Φ-class bee event.
gollark: The original definition was, yes
gollark: See, imagine if you could do that *automatically* using within-image meme provenance data.

See also
  • Fish curve, the negative pedal curve of an ellipse with squared eccentricity 1/2
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.