Mass-spring-damper model
The mass-spring-damper model consists of discrete mass nodes distributed throughout an object and interconnected via a network of springs and dampers. This model is well-suited for modelling object with complex material properties such as nonlinearity and viscoelasticity. Packages such as MATLAB may be used to run simulations of such models.[1] Objects may be described as volumetric meshes for simulation in this manner. As well as engineering simulation, these systems have applications in computer graphics and computer animation[2]
Derivation (Single Mass)
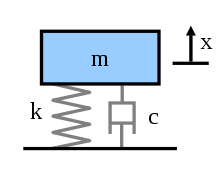
Deriving the equations of motion for this model is usually done by examining the sum of forces on the mass:
By rearranging this equation, we can derive the standard form:[3] where
is the undamped natural frequency
is the damping ratio
See also
- Numerical methods
- Soft body dynamics#Spring/mass models
- Finite element analysis
References
- "Solving mass spring damper systems in MATLAB" (PDF).
- "Fast Simulation of Mass-Spring Systems" (PDF).
- Longoria, Prof. R.G. "Modeling and Experimentation: Mass-Spring-Damper System Dynamics" (PDF). Retrieved 2019-11-19.