Image (category theory)

In category theory, a branch of mathematics, the image of a morphism is a generalization of the image of a function.

General Definition

Given a category ${\displaystyle C}$ and a morphism ${\displaystyle f\colon X\to Y}$ in ${\displaystyle C}$, the image [1] of ${\displaystyle f}$ is a monomorphism ${\displaystyle m\colon I\to Y}$ satisfying the following universal property:

1. There exists a morphism ${\displaystyle e\colon X\to I}$ such that ${\displaystyle f=m\,e}$.
2. For any object ${\displaystyle I'}$ with a morphism ${\displaystyle e'\colon X\to I'}$ and a monomorphism ${\displaystyle m'\colon I'\to Y}$ such that ${\displaystyle f=m'\,e'}$, there exists a unique morphism ${\displaystyle v\colon I\to I'}$ such that ${\displaystyle m=m'\,v}$.

Remarks:

1. such a factorization does not necessarily exist.
2. ${\displaystyle e}$ is unique by definition of ${\displaystyle m}$ monic.
3. ${\displaystyle m'e'=f=me=m've\implies e'=ve}$ by ${\displaystyle m}$ monic.
4. ${\displaystyle v}$ is monic.
5. ${\displaystyle m=m'\,v}$ already implies that ${\displaystyle v}$ is unique.

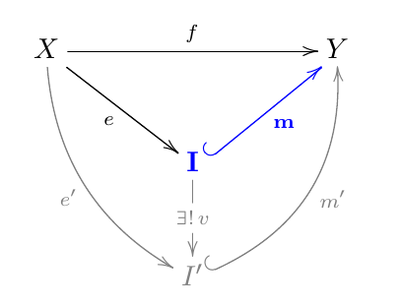
The image of ${\displaystyle f}$ is often denoted by ${\displaystyle {\text{Im}}f}$ or ${\displaystyle {\text{Im}}(f)}$.

Proposition: If ${\displaystyle C}$ has all equalizers then the ${\displaystyle e}$ in the factorization ${\displaystyle f=m\,e}$ of (1) is an epimorphism. [2]

Proof —

Let ${\displaystyle \alpha ,\,\beta }$ be such that ${\displaystyle \alpha \,e=\beta \,e}$, one needs to show that ${\displaystyle \alpha =\beta }$. Since the equalizer of ${\displaystyle (\alpha ,\beta )}$ exists, ${\displaystyle e}$ factorizes as ${\displaystyle e=q\,e'}$ with ${\displaystyle q}$ monic. But then ${\displaystyle f=(m\,q)\,e'}$ is a factorization of ${\displaystyle f}$ with ${\displaystyle (m\,q)}$ monomorphism. Hence by the universal property of the image there exists a unique arrow ${\displaystyle v:I\to Eq_{\alpha ,\beta }}$ such that ${\displaystyle m=m\,q\,v}$ and since ${\displaystyle m}$ is monic ${\displaystyle {\text{id}}_{I}=q\,v}$. Furthermore, one has ${\displaystyle m\,q=(mqv)\,q}$ and by the monomorphism property of ${\displaystyle mq}$ one obtains ${\displaystyle {\text{id}}_{Eq_{\alpha ,\beta }}=v\,q}$.

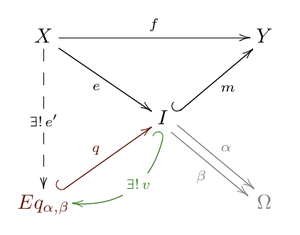
This means that ${\displaystyle I\equiv Eq_{\alpha ,\beta }}$ and thus that ${\displaystyle {\text{id}}_{I}=q\,v}$ equalizes ${\displaystyle (\alpha ,\beta )}$, whence ${\displaystyle \alpha =\beta }$.

Second definition

In a category ${\displaystyle C}$ with all finite limits and colimits, the image is defined as the equalizer ${\displaystyle (Im,m)}$ of the so-called cokernel pair ${\displaystyle (Y\sqcup _{X}Y,i_{1},i_{2})}$ .[3]

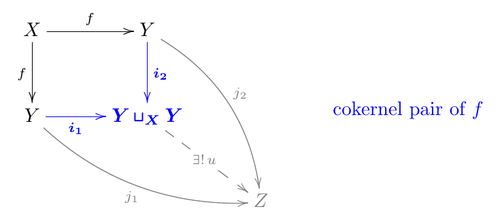
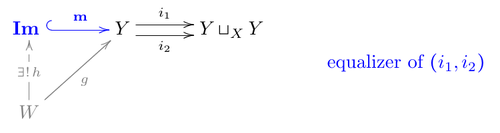
Remarks:

1. Finite bicompleteness of the category ensures that pushouts and equalizers exist.
2. ${\displaystyle (Im,m)}$ can be called regular image as ${\displaystyle m}$ is a regular monomorphism, i.e. the equalizer of a pair of morphism. (Recall also that an equalizer is automatically a monomorphism).
3. In an abelian category, the cokernel pair property can be written ${\displaystyle i_{1}\,f=i_{2}\,f\ \Leftrightarrow \ (i_{1}-i_{2})\,f=0=0\,f}$ and the equalizer condition ${\displaystyle i_{1}\,m=i_{2}\,m\ \Leftrightarrow \ (i_{1}-i_{2})\,m=0\,m}$. Moreover, all monomorphisms are regular.

Theorem — If ${\displaystyle f}$ always factorizes through regular monomorphisms, then the two definitions coincide.

Proof —

First definition implies the second: Assume that (1) holds with ${\displaystyle m}$ regular monomorphism.

• Equalization: one needs to show that ${\displaystyle i_{1}\,m=i_{2}\,m}$ . As the cokernel pair of ${\displaystyle f,\ i_{1}\,f=i_{2}\,f}$ and by previous proposition, since ${\displaystyle C}$ has all equalizers, the arrow ${\displaystyle e}$ in the factorization ${\displaystyle f=m\,e}$ is an epimorphism, hence ${\displaystyle i_{1}\,f=i_{2}\,f\ \Rightarrow \ i_{1}\,m=i_{2}\,m}$.
• Universality: in a category with all colimits (or at least all pushouts) ${\displaystyle m}$ itself admits a cokernel pair ${\displaystyle (Y\sqcup _{I}Y,c_{1},c_{2})}$

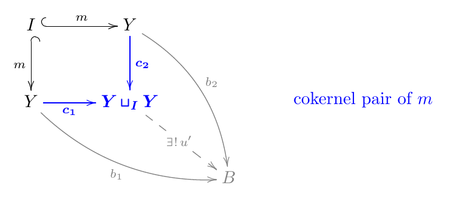
Moreover, as a regular monomorphism, ${\displaystyle (I,m)}$ is the equalizer of a pair of morphisms ${\displaystyle b_{1},b_{2}:Y\longrightarrow B}$ but we claim here that it is also the equalizer of ${\displaystyle c_{1},c_{2}:Y\longrightarrow Y\sqcup _{I}Y}$.

Indeed, by construction ${\displaystyle b_{1}\,m=b_{2}\,m}$ thus the "cokernel pair" diagram for ${\displaystyle m}$ yields a unique morphism ${\displaystyle u':Y\sqcup _{I}Y\longrightarrow B}$ such that ${\displaystyle b_{1}=u'\,c_{1},\ b_{2}=u'\,c_{2}}$. Now, a map ${\displaystyle m':I'\longrightarrow Y}$ which equalizes ${\displaystyle (c_{1},c_{2})}$ also satisfies ${\displaystyle b_{1}\,m'=u'\,c_{1}\,m'=u'\,c_{2}\,m'=b_{2}\,m'}$, hence by the equalizer diagram for ${\displaystyle (b_{1},b_{2})}$, there exists a unique map ${\displaystyle h':I'\to I}$ such that ${\displaystyle m'=m\,h'}$.

Finally, use the cokernel pair diagram (of ${\displaystyle f}$) with ${\displaystyle j_{1}:=c_{1},\ j_{2}:=c_{2},\ Z:=Y\sqcup _{I}Y}$ : there exists a unique ${\displaystyle u:Y\sqcup _{X}Y\longrightarrow Y\sqcup _{I}Y}$ such that ${\displaystyle c_{1}=u\,i_{1},\ c_{2}=u\,i_{2}}$. Therefore, any map ${\displaystyle g}$ which equalizes ${\displaystyle (i_{1},i_{2})}$ also equalizes ${\displaystyle (c_{1},c_{2})}$ and thus uniquely factorizes as ${\displaystyle g=m\,h'}$. This exactly means that ${\displaystyle (I,m)}$ is the equalizer of ${\displaystyle (i_{1},i_{2})}$.

Second definition implies the first:

• Factorization: taking ${\displaystyle m':=f}$ in the equalizer diagram (${\displaystyle m'}$ corresponds to ${\displaystyle g}$), one obtains the factorization ${\displaystyle f=m\,h}$.
• Universality: let ${\displaystyle f=m'\,e'}$ be a factorization with ${\displaystyle m'}$ regular monomorphism, i.e. the equalizer of some pair ${\displaystyle (d_{1},d_{2})}$.

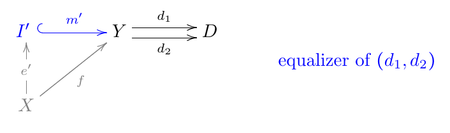
Then ${\displaystyle d_{1}\,m'=d_{2}\,m'\ \Rightarrow \ d_{1}\,f=d_{1}\,m'\,e=d_{2}\,m'\,e=d_{2}\,f}$ so that by the "cokernel pair" diagram (of ${\displaystyle f}$), with ${\displaystyle j_{1}:=d_{1},\ j_{2}:=d_{2},\ Z:=D}$, there exists a unique ${\displaystyle u'':Y\sqcup _{X}Y\longrightarrow D}$ such that ${\displaystyle d_{1}=u''\,i_{1},\ d_{2}=u''\,i_{2}}$.

Now, from ${\displaystyle i_{1}\,m=i_{2}\,m}$ (m from the equalizer of (i₁, i₂) diagram), one obtains ${\displaystyle d_{1}\,m=u''\,i_{1}\,m=u''\,i_{2}\,m=d_{2}\,m}$, hence by the universality in the (equalizer of (d₁, d₂) diagram, with f replaced by m), there exists a unique ${\displaystyle v:Im\longrightarrow I'}$ such that ${\displaystyle m=m'\,v}$.

Examples

In the category of sets the image of a morphism ${\displaystyle f\colon X\to Y}$ is the inclusion from the ordinary image ${\displaystyle \{f(x)~|~x\in X\}}$ to ${\displaystyle Y}$. In many concrete categories such as groups, abelian groups and (left- or right) modules, the image of a morphism is the image of the correspondent morphism in the category of sets.

In any normal category with a zero object and kernels and cokernels for every morphism, the image of a morphism ${\displaystyle f}$ can be expressed as follows:

im f = ker coker f

In an abelian category (which is in particular binormal), if f is a monomorphism then f = ker coker f, and so f = im f.

See also

• Subobject
• Coimage
• Image (mathematics)

References

1. Mitchell, Barry (1965), Theory of categories, Pure and applied mathematics, 17, Academic Press, ISBN 978-0-124-99250-4, MR 0202787 Section I.10 p.12
2. Mitchell, Barry (1965), Theory of categories, Pure and applied mathematics, 17, Academic Press, ISBN 978-0-124-99250-4, MR 0202787 Proposition 10.1 p.12
3. Kashiwara, Masaki; Schapira, Pierre (2006), "Categories and Sheaves", Grundlehren der Mathematischen Wissenschaften, 332, Berlin Heidelberg: Springer, pp. 113–114 Definition 5.1.1