Effective nuclear charge

The effective nuclear charge (often symbolized as ${\displaystyle Z_{\mathrm {eff} }}$ or ${\displaystyle Z^{\ast }}$) is the net positive charge experienced by an electron in a polyelectronic atom. The term "effective" is used because the shielding effect of negatively charged electrons prevents higher orbital electrons from experiencing the full nuclear charge of the nucleus due to the repelling effect of inner-layer electrons. The effective nuclear charge experienced by the electron is also called the core charge. It is possible to determine the strength of the nuclear charge by the oxidation number of the atom. Most of the physical and chemical properties of the elements can be explained on the basis of electronic configuration. Consider the behavior of ionization energies in the periodic table. It is known that the magnitude of ionization potential depends upon the following factors:

Effective nuclear charge diagram

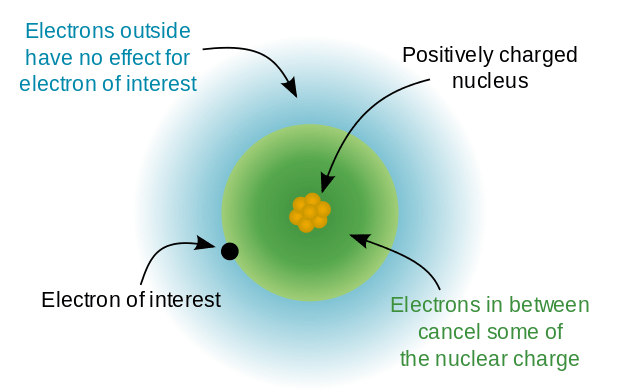
(a) Size of atom;

(b) The nuclear charge;

(c) The screening effect of the inner shells, and;

(d) The extent to which the outermost electron penetrates into the charge cloud set up by the inner lying electron.

Calculations

In an atom with one electron, that electron experiences the full charge of the positive nucleus. In this case, the effective nuclear charge can be calculated by Coulomb's law.

However, in an atom with many electrons, the outer electrons are simultaneously attracted to the positive nucleus and repelled by the negatively charged electrons. The effective nuclear charge on such an electron is given by the following equation:

${\displaystyle Z_{\mathrm {eff} }=Z-S}$

where

Z is the number of protons in the nucleus (atomic number), and

S is the shielding constant.

S can be found by the systematic application of various rule sets, the simplest of which is known as "Slater's rules" (named after John C. Slater). Douglas Hartree defined the effective Z of a Hartree–Fock orbital to be:

${\displaystyle Z_{\mathrm {eff} }={\frac {\langle r\rangle _{\rm {H}}}{\langle r\rangle _{Z}}}}$

where

${\displaystyle \langle r\rangle _{\rm {H}}}$ is the mean radius of the orbital for hydrogen, and

${\displaystyle \langle r\rangle _{Z}}$ is the mean radius of the orbital for a proton configuration with nuclear charge Z.

Values

Updated effective nuclear charge values were provided by Clementi et al. in 1963 and 1967.[1][2] In their work, screening constants were optimized to produce effective nuclear charge values that agree with SCF calculations. Though useful as a predictive model, the resulting screening constants contain little chemical insight as a qualitative model of atomic structure.

Effective Nuclear Charges

	H																	He
Z	1																	2
1s	1.000																	1.688
	Li	Be											B	C	N	O	F	Ne
Z	3	4											5	6	7	8	9	10
1s	2.691	3.685											4.680	5.673	6.665	7.658	8.650	9.642
2s	1.279	1.912											2.576	3.217	3.847	4.492	5.128	5.758
2p													2.421	3.136	3.834	4.453	5.100	5.758
	Na	Mg											Al	Si	P	S	Cl	Ar
Z	11	12											13	14	15	16	17	18
1s	10.626	11.609											12.591	13.575	14.558	15.541	16.524	17.508
2s	6.571	7.392											8.214	9.020	9.825	10.629	11.430	12.230
2p	6.802	7.826											8.963	9.945	10.961	11.977	12.993	14.008
3s	2.507	3.308											4.117	4.903	5.642	6.367	7.068	7.757
3p													4.066	4.285	4.886	5.482	6.116	6.764
	K	Ca	Sc	Ti	V	Cr	Mn	Fe	Co	Ni	Cu	Zn	Ga	Ge	As	Se	Br	Kr
Z	19	20	21	22	23	24	25	26	27	28	29	30	31	32	33	34	35	36
1s	18.490	19.473	20.457	21.441	22.426	23.414	24.396	25.381	26.367	27.353	28.339	29.325	30.309	31.294	32.278	33.262	34.247	35.232
2s	13.006	13.776	14.574	15.377	16.181	16.984	17.794	18.599	19.405	20.213	21.020	21.828	22.599	23.365	24.127	24.888	25.643	26.398
2p	15.027	16.041	17.055	18.065	19.073	20.075	21.084	22.089	23.092	24.095	25.097	26.098	27.091	28.082	29.074	30.065	31.056	32.047
3s	8.680	9.602	10.340	11.033	11.709	12.368	13.018	13.676	14.322	14.961	15.594	16.219	16.996	17.790	18.596	19.403	20.219	21.033
3p	7.726	8.658	9.406	10.104	10.785	11.466	12.109	12.778	13.435	14.085	14.731	15.369	16.204	17.014	17.850	18.705	19.571	20.434
4s	3.495	4.398	4.632	4.817	4.981	5.133	5.283	5.434	5.576	5.711	5.842	5.965	7.067	8.044	8.944	9.758	10.553	11.316
3d			7.120	8.141	8.983	9.757	10.528	11.180	11.855	12.530	13.201	13.878	15.093	16.251	17.378	18.477	19.559	20.626
4p													6.222	6.780	7.449	8.287	9.028	9.338
	Rb	Sr	Y	Zr	Nb	Mo	Tc	Ru	Rh	Pd	Ag	Cd	In	Sn	Sb	Te	I	Xe
Z	37	38	39	40	41	42	43	44	45	46	47	48	49	50	51	52	53	54
1s	36.208	37.191	38.176	39.159	40.142	41.126	42.109	43.092	44.076	45.059	46.042	47.026	48.010	48.992	49.974	50.957	51.939	52.922
2s	27.157	27.902	28.622	29.374	30.125	30.877	31.628	32.380	33.155	33.883	34.634	35.386	36.124	36.859	37.595	38.331	39.067	39.803
2p	33.039	34.030	35.003	35.993	36.982	37.972	38.941	39.951	40.940	41.930	42.919	43.909	44.898	45.885	46.873	47.860	48.847	49.835
3s	21.843	22.664	23.552	24.362	25.172	25.982	26.792	27.601	28.439	29.221	30.031	30.841	31.631	32.420	33.209	33.998	34.787	35.576
3p	21.303	22.168	23.093	23.846	24.616	25.474	26.384	27.221	28.154	29.020	29.809	30.692	31.521	32.353	33.184	34.009	34.841	35.668
4s	12.388	13.444	14.264	14.902	15.283	16.096	17.198	17.656	18.582	18.986	19.865	20.869	21.761	22.658	23.544	24.408	25.297	26.173
3d	21.679	22.726	25.397	25.567	26.247	27.228	28.353	29.359	30.405	31.451	32.540	33.607	34.678	35.742	36.800	37.839	38.901	39.947
4p	10.881	11.932	12.746	13.460	14.084	14.977	15.811	16.435	17.140	17.723	18.562	19.411	20.369	21.265	22.181	23.122	24.030	24.957
5s	4.985	6.071	6.256	6.446	5.921	6.106	7.227	6.485	6.640	(empty)	6.756	8.192	9.512	10.629	11.617	12.538	13.404	14.218
4d			15.958	13.072	11.238	11.392	12.882	12.813	13.442	13.618	14.763	15.877	16.942	17.970	18.974	19.960	20.934	21.893
5p													8.470	9.102	9.995	10.809	11.612	12.425

See also

• Atomic orbitals
• Core charge
• d-block contraction (or scandide contraction)
• Electronegativity
• Lanthanide contraction
• Shielding effect
• Slater-type orbitals
• Valence electrons
• Weak charge

References

1. Clementi, E.; Raimondi, D. L. (1963). "Atomic Screening Constants from SCF Functions". J. Chem. Phys. 38 (11): 2686–2689. Bibcode:1963JChPh..38.2686C. doi:10.1063/1.1733573.
2. Clementi, E.; Raimondi, D. L.; Reinhardt, W. P. (1967). "Atomic Screening Constants from SCF Functions. II. Atoms with 37 to 86 Electrons". Journal of Chemical Physics. 47: 1300–1307. Bibcode:1967JChPh..47.1300C. doi:10.1063/1.1712084.

Resources

• Brown, Theodore; LeMay, H.E.; & Bursten, Bruce (2002). Chemistry: The Central Science (8th revised edition). Upper Saddle River, New Jersey 07458: Prentice-Hall. ISBN 0-13-061142-5.