Brownian noise

In science, Brownian noise (Sample ), also known as Brown noise or red noise, is the kind of signal noise produced by Brownian motion, hence its alternative name of random walk noise. The term "Brown noise" does not come from the color, but after Robert Brown, who documented the erratic motion for multiple types of inanimate particles in water. The term "red noise" comes from the "white noise"/"white light" analogy; red noise is strong in longer wavelengths, similar to the red end of the visible spectrum.

Colors of noise
White
Pink
Red (Brownian)
Grey

Spectrum of Brownian noise, with a slope of –20 dB per decade

Explanation

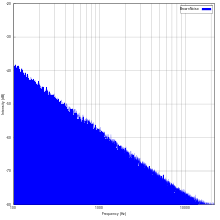
The graphic representation of the sound signal mimics a Brownian pattern. Its spectral density is inversely proportional to f ², meaning it has more energy at lower frequencies, even more so than pink noise. It decreases in power by 6 dB per octave (20 dB per decade) and, when heard, has a "damped" or "soft" quality compared to white and pink noise. The sound is a low roar resembling a waterfall or heavy rainfall. See also violet noise, which is a 6 dB increase per octave.

Strictly, Brownian motion has a Gaussian probability distribution, but "red noise" could apply to any signal with the 1/f ² frequency spectrum.

Power spectrum

A Brownian motion, also called a Wiener process, is obtained as the integral of a white noise signal:

${\displaystyle W(t)=\int _{0}^{t}{\frac {dW(\tau )}{d\tau }}d\tau }$

meaning that Brownian motion is the integral of the white noise ${\displaystyle dW(t)}$, whose power spectral density is flat:[1]

${\displaystyle S_{0}=\left|{\mathcal {F}}\left[{\frac {dW(t)}{dt}}\right](\omega )\right|^{2}={\text{const}}.}$

Note that here ${\displaystyle {\mathcal {F}}}$ denotes the Fourier transform, and ${\displaystyle S_{0}}$ is a constant. An important property of this transform is that the derivative of any distribution transforms as[2]

${\displaystyle {\mathcal {F}}\left[{\frac {dW(t)}{dt}}\right](\omega )=i\omega {\mathcal {F}}[W(t)](\omega ),}$

from which we can conclude that the power spectrum of Brownian noise is

${\displaystyle S(\omega )={\big |}{\mathcal {F}}[W(t)](\omega ){\big |}^{2}={\frac {S_{0}}{\omega ^{2}}}.}$

An individual Brownian motion trajectory presents a spectrum ${\displaystyle S(\omega )=S_{0}/\omega ^{2}}$, where the amplitude ${\displaystyle S_{0}}$ is a random variable, even in the limit of an infinitely long trajectory [3].

Production

Brown noise can be produced by integrating white noise.[4][5] That is, whereas (digital) white noise can be produced by randomly choosing each sample independently, Brown noise can be produced by adding a random offset to each sample to obtain the next one. A leaky integrator might be used in audio applications to ensure the signal does not "wander off". Note that while the first sample is random across the entire range that the sound sample can take on, the remaining offsets from there on are a tenth or thereabouts, leaving room for the signal to bounce around.

Sample

References

1. Gardiner, C. W. Handbook of stochastic methods. Berlin: Springer Verlag.
2. Barnes, J. A. & Allan, D. W. (1966). "A statistical model of flicker noise". Proceedings of the IEEE. 54 (2): 176–178. doi:10.1109/proc.1966.4630. and references therein
3. Krapf, Diego; Marinari, Enzo; Metzler, Ralf; Oshanin, Gleb; Xu, Xinran; Squarcini, Alessio (2018-02-09). "Power spectral density of a single Brownian trajectory: what one can and cannot learn from it". New Journal of Physics. 20 (2): 023029. arXiv:1801.02986. Bibcode:2018NJPh...20b3029K. doi:10.1088/1367-2630/aaa67c.
4. "Integral of White noise". 2005.
5. Bourke, Paul (October 1998). "Generating noise with different power spectra laws".