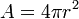Lunar bukkake hypothesis
The lunar bukkake hypothesis is a young-Earth creationist conjecture regarding the origin of craters on the Moon. It has been proposed and repeated by YouTube creationist NephilimFree, though he didn't give it the derogatory name used here.[note 1]
| Rescued from the Global Flood |
| Twelve of the clean kind |
v - t - e |
How it works
One of the many problems with young-Earth creationism is that the Moon (among other bodies in the solar system) has a heavily cratered surface. The number of craters, given the known rate of meteor impacts across the solar system, is far too great for it to have all been freshly formed 6000 years ago. Barring Goddidit or any other form of magic, this presents an issue for creationists.
According to NephilimFree, the water from Noah's global flood lay under the Earth's crust — in line with hydroplate "theory".[1] During the flood, the crust dropped down and this forced all the water to shoot upwards, again, in line with the hydroplate idea. However, the extension to explain the craters on the moon suggests that the water, now traveling far beyond hypersonic (merely "supersonic" according to Nephilimfree) speed, would have gone up and splattered the moon (hence the name of the hypothesis) and caused all the craters to form (on the far side as well, apparently). NephilimFree also expands this ad-hoc speculation theory to explain the existence of comets and a similar suggestion has been touted as the explanation for water on other planets.[2]
Minor scientific problems
Conservation of energy
Primarily, the reason this cannot work is an issue of conservation of energy. This is simple to illustrate. Consider a 10 km thick section of rock; its width and breadth don't matter much, but make it a 1km by 1km slice for the sake of unit numbers. If this drops straight down into a 1 km deep underground ocean, under gravity, the amount of energy released is the equivalent of just a 1 km section dropping 10 km down (see diagram). Given a perfect system where energy cannot be lost (to friction, for example), this is enough energy to send another lump of rock 10 km up. The density of water is about a quarter or so of the Earth's crust so it can go a little higher, about 40 km up. Not exactly enough to get to the moon, which is nearly 10,000 times further away.[3]
Adding in friction, such as resistance of rock and air, the height that the water can reach as a result of these hydroplates dropping becomes much smaller. This is difficult to quantise precisely, but it certainly won't improve things for this creationist nonsense. So by the same principle that means the fat kid is the ruler of the see-saw, we can demonstrate the concept is fundamentally flawed before getting into the details of these creationist insights.
In order to reproduce the effects as told we can add an energy input by pushing the earth's crust down (as opposed to gravity alone) with some sort of force. This solution is analogus to intelligent falling.
The target size
Assume, briefly, that a severe enough earthquake can cause water to shoot up at escape velocity — and enough water at that. The moon is a relatively small target, about 30 arc minutes (half a degree) across in the sky.[note 3] Therefore the odds of hitting it with any of the ice meteors produced by NephilimFree's method is very low, unless the amount of water released in this manner was much, much, much greater than even NephilimFree claims. Assuming a reasonably even distribution of water flying up into space, we can calculate how much would eventually hit the moon. The surface of a sphere is calculated from a well-known classroom equation:
So the Earth's surface therefore works out to 500,000,000 km2 thanks to a mean radius of just over 6,000 km. Assuming 1 km of water under the crust we have, simply,[note 4] 500,000,000 (5 x 108) km3 of water. But how thinly spread would this water be once it's been fired half a million kilometres to the moon? A sphere that would stretch to the moon would have a surface of approximately 2,000,000,000,000 (2 x 1012) km2 - 4000 times larger than the Earth by surface area. Thus dividing our 1 km depth of water we find that there would be only 25 centimetres depth of water that would statistically hit the moon. Hardly the grand splattering required to generate the 300,000 or so craters on the moon's surface.[4]
Alternatively, you can move the moon much, much closer (like at Roche limit
The theory also hardly explains the even more cratered surface of the far side of the moon or the craters on every other body that has been examined in the solar system.
Nozzle velocity
NephilmFree has defended his claim with the idea that the "nozzle size" matters. That is, when you try to squeeze water through a smaller space, it speeds up. This is true, but only in a system where pressure can build up behind the bottle neck (either Neph is misapplying[note 6] Bernoulli's principle
The water would have to reach Earth's escape velocity (at least) in order to hit the moon. It's a common misconception that this is the speed you have to achieve to "break free" of the Earth's gravitational pull - it's just the speed you have to be travelling when you cut your engines off and stop applying additional force. This value actually decreases with altitude; thus providing you constantly push yourself upwards with consistently applied force you could break free of the Earth's pull at any speed. This is why rockets or even a space elevator can leave the Earth's orbit at a lower speed - they're constantly applying force with their motors and engines. However, in the case of water shooting up from the Earth's surface, the "misconception" is actually fairly good representation as the water cannot gain more momentum from motors or engines. In this hypothesis it has only one chance to receive energy in the form of momentum so it would have to be accelerated to the surface escape velocity. The water would have to be shooting up from Earth's surface at 11.2 km/s (40,320 km/h) in order to escape the Earth's gravity and make its way towards the moon. The accelerating force required to do this is far, far above what you could get[note 7] from even dropping ten cubic kilometres of rock onto some water, regardless of NephilimFree's erroneous claims about nozzle velocity (which is what the above discussion about conservation of momentum confirms).
The only way to accelerate water to the 11.2 km/s required would be if a force other than gravity acted on the rock pushing down, taking all these heavy tectonic plates and pushing them a kilometre down into the Earth in a fraction of a second. We can estimate the mass of the Earth's crust at 1.913x1022 kg,[5] a number so large that even if it was a gross over-estimate it would still be huge. Kinetic energy is 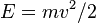 , so plugging the mass and speed required results in 1.2 x1030 Joules of energy involved - this is over a billion times the energy released when a 10 km asteroid whacks into the Earth.[6] Yes, for the energy and physics of this hypothesis to work out you need a billion extinction level events happening in under a second. And yet, Noah's flimsy little Ark is expected to survive it!
, so plugging the mass and speed required results in 1.2 x1030 Joules of energy involved - this is over a billion times the energy released when a 10 km asteroid whacks into the Earth.[6] Yes, for the energy and physics of this hypothesis to work out you need a billion extinction level events happening in under a second. And yet, Noah's flimsy little Ark is expected to survive it!
Vaporization of water
If water were accelerated to a velocity sufficient for it to reach the moon, passage through the atmosphere would surely vaporize it (water at that velocity would be like a meteor in the air, very hot), and even if liquid water were to reach above the atmosphere, it would not freeze in space, as the pressure went to zero, it would evaporate in the passage to the Moon, (and isolated ice crystals would rapidly sublimate), so it would then be, at most, a kind of wind, not like the impact of a solid mass.
Comet composition
An additional claim is that the ejected water then goes on to explain the existence of comets in the Solar System - although certainly not their highly elliptical and far-reaching orbits. This can be demonstrated to be bullshit by actually looking at the composition of comet ice, which has been measured with spectroscopic methods by various spacecraft.[8] As a result of this, we know that comets can be around 0.1% hydrogen cyanide (HCN) and anywhere up to 15-20% carbon monoxide (CO). In the case of the HCN, this doesn't seem like much but would make drinking about 1 litre of water (assuming Noah's flood waters had the same composition as comets) to be lethal. In order for the CO to be present in such high concentrations, the water would have to have been on Earth under a partial pressure of CO. As it stands, CO dissolves poorly in water and a significantly high pressure would be needed to force the gas to dissolve. Working backwards from the comet concentration we can determine that the atmosphere during Noah's great flood would be about 0.01 atmospheres of CO. Like the cyanide, this concentration is above the lethal dose - which is around 1% CO to kill within minutes.[9] In short, if this was the case surviving the flood would have required far more than a sturdy boat - God's orders to Noah should therefore have involved instructions to build space-age life support systems!
Temperature
Using Geothermal gradient
Fossils on the moon
Bearing some similarity to known conspiracy theories and far-out ideas about history,[11] NephilmFree has also suggested that lunar bukkake theory would result in us finding fossilized remains on the moon. This would be quite consistent with huge quantities of water splashing up and hitting the moon, although not entirely consistent with water travelling at the 11.2 km/s escape velocity for it to leave the Earth's surface, which would have shredded any remains pretty quick - by comparison, a water jet cutter
Notes
- If you don't get it already, you're probably not the sort that wants to know, so don't bother looking it up
File:Wikipedia's W.svg . In case you do look it up, it's not about noodles or cuisineFile:Wikipedia's W.svg . - You can satisfy yourself that this hand-waving simplification works by imagining a point mass of 10 cubic km of rock dropping only 1 km (thus the force is proportional to 10 x 1) compared to just 1 cubic km of rock dropping 10 km (thus the force is proportional to 1 x 10).
- "I used to bullseye womp rats in my T-16 back home. They're not much bigger than 30 arc minutes!"
- This is a simplification. We'd have to integrate the volume between a sphere of 6000 km and 6001 km to get a real value. The size of the spheres in question make the difference negligible and less than the margin of error thanks to the fact the Earth isn't a perfect sphere anyway. Also, that's difficult.
- Roche limit of 9481km - earth's mean radius of 6371km = 3110km
- By "misapplying" we meant "totally lacking understanding of"
- Doing the math would get you no more than say 650 m/s (velocity increase is proportional to the square root of pressure increase so it will never be much), slightly less than Mach 2 but still not enough to reach even 10% of the surface escape velocity.
References
- The Center for "Scientific" Creation - Hydroplate "theory"
- CNAV - Ice on Mercury
- Why do people laugh at creationists? (part 33)
- ESA - Moon facts
- An estimate, for various reasons, discussed here.
- Effects of an Asteroid Impact on Earth
- Woods, T. N.; Feldman, P. D.; Dymond, K. F.; Sahnow, D. J.; Rocket ultraviolet spectroscopy of comet Halley and abundance of carbon monoxide and carbon. Nature, 324, 436-438 (1986)
- NASA.gov - Comets (archive)
- Why Do People Laugh At Creationists? (Part 34)
- Checking the phase diagram validates that it is still in liquid form (~300 MPa, 500K).
- Overcoming Bias - Dinos on the Moon