23
5
The Bernoulli numbers (specifically, the second Bernoulli numbers) are defined by the following recursive definition:
Where 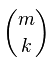 denotes a combination.
denotes a combination.
Given a nonnegative integer m as input, output the decimal representation OR a reduced fraction for the mth second Bernoulli number. If you output a decimal representation, you must have at least 6 decimal places (digits after the decimal point) of precision, and it must be accurate when rounded to 6 decimal places. For example, for m = 2, 0.166666523 is acceptable because it rounds to 0.166667. 0.166666389 is not acceptable, because it rounds to 0.166666. Trailing zeroes may be omitted. Scientific notation may be used for decimal representations.
Here is the input and expected output for m up to and including 60, in scientific notation rounded to 6 decimal places, and as reduced fractions:
0 -> 1.000000e+00 (1/1)
1 -> 5.000000e-01 (1/2)
2 -> 1.666667e-01 (1/6)
3 -> 0.000000e+00 (0/1)
4 -> -3.333333e-02 (-1/30)
5 -> 0.000000e+00 (0/1)
6 -> 2.380952e-02 (1/42)
7 -> 0.000000e+00 (0/1)
8 -> -3.333333e-02 (-1/30)
9 -> 0.000000e+00 (0/1)
10 -> 7.575758e-02 (5/66)
11 -> 0.000000e+00 (0/1)
12 -> -2.531136e-01 (-691/2730)
13 -> 0.000000e+00 (0/1)
14 -> 1.166667e+00 (7/6)
15 -> 0.000000e+00 (0/1)
16 -> -7.092157e+00 (-3617/510)
17 -> 0.000000e+00 (0/1)
18 -> 5.497118e+01 (43867/798)
19 -> 0.000000e+00 (0/1)
20 -> -5.291242e+02 (-174611/330)
21 -> 0.000000e+00 (0/1)
22 -> 6.192123e+03 (854513/138)
23 -> 0.000000e+00 (0/1)
24 -> -8.658025e+04 (-236364091/2730)
25 -> 0.000000e+00 (0/1)
26 -> 1.425517e+06 (8553103/6)
27 -> 0.000000e+00 (0/1)
28 -> -2.729823e+07 (-23749461029/870)
29 -> 0.000000e+00 (0/1)
30 -> 6.015809e+08 (8615841276005/14322)
31 -> 0.000000e+00 (0/1)
32 -> -1.511632e+10 (-7709321041217/510)
33 -> 0.000000e+00 (0/1)
34 -> 4.296146e+11 (2577687858367/6)
35 -> 0.000000e+00 (0/1)
36 -> -1.371166e+13 (-26315271553053477373/1919190)
37 -> 0.000000e+00 (0/1)
38 -> 4.883323e+14 (2929993913841559/6)
39 -> 0.000000e+00 (0/1)
40 -> -1.929658e+16 (-261082718496449122051/13530)
41 -> 0.000000e+00 (0/1)
42 -> 8.416930e+17 (1520097643918070802691/1806)
43 -> 0.000000e+00 (0/1)
44 -> -4.033807e+19 (-27833269579301024235023/690)
45 -> 0.000000e+00 (0/1)
46 -> 2.115075e+21 (596451111593912163277961/282)
47 -> 0.000000e+00 (0/1)
48 -> -1.208663e+23 (-5609403368997817686249127547/46410)
49 -> 0.000000e+00 (0/1)
50 -> 7.500867e+24 (495057205241079648212477525/66)
51 -> 0.000000e+00 (0/1)
52 -> -5.038778e+26 (-801165718135489957347924991853/1590)
53 -> 0.000000e+00 (0/1)
54 -> 3.652878e+28 (29149963634884862421418123812691/798)
55 -> 0.000000e+00 (0/1)
56 -> -2.849877e+30 (-2479392929313226753685415739663229/870)
57 -> 0.000000e+00 (0/1)
58 -> 2.386543e+32 (84483613348880041862046775994036021/354)
59 -> 0.000000e+00 (0/1)
60 -> -2.139995e+34 (-1215233140483755572040304994079820246041491/56786730)
Reference implementation (in Python 3):
def factorial(n):
if n < 1:
return 1
else:
return n * factorial(n - 1)
def combination(m,k):
if k <= m:
return factorial(m)/(factorial(k) * factorial(m - k))
else:
return 0
def Bernoulli(m):
if m == 0:
return 1
else:
t = 0
for k in range(0, m):
t += combination(m, k) * Bernoulli(k) / (m - k + 1)
return 1 - t
Rules
- This is code-golf, so shortest code in bytes wins
- You may not use any functions, either built-in or included in an external library, that compute either type of Bernoulli numbers or Bernoulli polynomials.
- Your answer must give correct output for all inputs up to and including 60.
Leaderboard
The Stack Snippet at the bottom of this post generates the leaderboard from the answers a) as a list of shortest solution per language and b) as an overall leaderboard.
To make sure that your answer shows up, please start your answer with a headline, using the following Markdown template:
## Language Name, N bytes
where N is the size of your submission. If you improve your score, you can keep old scores in the headline, by striking them through. For instance:
## Ruby, <s>104</s> <s>101</s> 96 bytes
If there you want to include multiple numbers in your header (e.g. because your score is the sum of two files or you want to list interpreter flag penalties separately), make sure that the actual score is the last number in the header:
## Perl, 43 + 2 (-p flag) = 45 bytes
You can also make the language name a link which will then show up in the snippet:
## [><>](http://esolangs.org/wiki/Fish), 121 bytes
<style>body { text-align: left !important} #answer-list { padding: 10px; width: 290px; float: left; } #language-list { padding: 10px; width: 290px; float: left; } table thead { font-weight: bold; } table td { padding: 5px; }</style><script src="https://ajax.googleapis.com/ajax/libs/jquery/2.1.1/jquery.min.js"></script> <link rel="stylesheet" type="text/css" href="//cdn.sstatic.net/codegolf/all.css?v=83c949450c8b"> <div id="language-list"> <h2>Shortest Solution by Language</h2> <table class="language-list"> <thead> <tr><td>Language</td><td>User</td><td>Score</td></tr> </thead> <tbody id="languages"> </tbody> </table> </div> <div id="answer-list"> <h2>Leaderboard</h2> <table class="answer-list"> <thead> <tr><td></td><td>Author</td><td>Language</td><td>Size</td></tr> </thead> <tbody id="answers"> </tbody> </table> </div> <table style="display: none"> <tbody id="answer-template"> <tr><td>{{PLACE}}</td><td>{{NAME}}</td><td>{{LANGUAGE}}</td><td>{{SIZE}}</td><td><a href="{{LINK}}">Link</a></td></tr> </tbody> </table> <table style="display: none"> <tbody id="language-template"> <tr><td>{{LANGUAGE}}</td><td>{{NAME}}</td><td>{{SIZE}}</td><td><a href="{{LINK}}">Link</a></td></tr> </tbody> </table><script>var QUESTION_ID = 65382; var ANSWER_FILTER = "!t)IWYnsLAZle2tQ3KqrVveCRJfxcRLe"; var COMMENT_FILTER = "!)Q2B_A2kjfAiU78X(md6BoYk"; var OVERRIDE_USER = 45941; var answers = [], answers_hash, answer_ids, answer_page = 1, more_answers = true, comment_page; function answersUrl(index) { return "https://api.stackexchange.com/2.2/questions/" + QUESTION_ID + "/answers?page=" + index + "&pagesize=100&order=desc&sort=creation&site=codegolf&filter=" + ANSWER_FILTER; } function commentUrl(index, answers) { return "https://api.stackexchange.com/2.2/answers/" + answers.join(';') + "/comments?page=" + index + "&pagesize=100&order=desc&sort=creation&site=codegolf&filter=" + COMMENT_FILTER; } function getAnswers() { jQuery.ajax({ url: answersUrl(answer_page++), method: "get", dataType: "jsonp", crossDomain: true, success: function (data) { answers.push.apply(answers, data.items); answers_hash = []; answer_ids = []; data.items.forEach(function(a) { a.comments = []; var id = +a.share_link.match(/\d+/); answer_ids.push(id); answers_hash[id] = a; }); if (!data.has_more) more_answers = false; comment_page = 1; getComments(); } }); } function getComments() { jQuery.ajax({ url: commentUrl(comment_page++, answer_ids), method: "get", dataType: "jsonp", crossDomain: true, success: function (data) { data.items.forEach(function(c) { if (c.owner.user_id === OVERRIDE_USER) answers_hash[c.post_id].comments.push(c); }); if (data.has_more) getComments(); else if (more_answers) getAnswers(); else process(); } }); } getAnswers(); var SCORE_REG = /<h\d>\s*([^\n,<]*(?:<(?:[^\n>]*>[^\n<]*<\/[^\n>]*>)[^\n,<]*)*),.*?(\d+)(?=[^\n\d<>]*(?:<(?:s>[^\n<>]*<\/s>|[^\n<>]+>)[^\n\d<>]*)*<\/h\d>)/; var OVERRIDE_REG = /^Override\s*header:\s*/i; function getAuthorName(a) { return a.owner.display_name; } function process() { var valid = []; answers.forEach(function(a) { var body = a.body; a.comments.forEach(function(c) { if(OVERRIDE_REG.test(c.body)) body = '<h1>' + c.body.replace(OVERRIDE_REG, '') + '</h1>'; }); var match = body.match(SCORE_REG); if (match) valid.push({ user: getAuthorName(a), size: +match[2], language: match[1], link: a.share_link, }); else console.log(body); }); valid.sort(function (a, b) { var aB = a.size, bB = b.size; return aB - bB }); var languages = {}; var place = 1; var lastSize = null; var lastPlace = 1; valid.forEach(function (a) { if (a.size != lastSize) lastPlace = place; lastSize = a.size; ++place; var answer = jQuery("#answer-template").html(); answer = answer.replace("{{PLACE}}", lastPlace + ".") .replace("{{NAME}}", a.user) .replace("{{LANGUAGE}}", a.language) .replace("{{SIZE}}", a.size) .replace("{{LINK}}", a.link); answer = jQuery(answer); jQuery("#answers").append(answer); var lang = a.language; lang = jQuery('<a>'+lang+'</a>').text(); languages[lang] = languages[lang] || {lang: a.language, lang_raw: lang.toLowerCase(), user: a.user, size: a.size, link: a.link}; }); var langs = []; for (var lang in languages) if (languages.hasOwnProperty(lang)) langs.push(languages[lang]); langs.sort(function (a, b) { if (a.lang_raw > b.lang_raw) return 1; if (a.lang_raw < b.lang_raw) return -1; return 0; }); for (var i = 0; i < langs.length; ++i) { var language = jQuery("#language-template").html(); var lang = langs[i]; language = language.replace("{{LANGUAGE}}", lang.lang) .replace("{{NAME}}", lang.user) .replace("{{SIZE}}", lang.size) .replace("{{LINK}}", lang.link); language = jQuery(language); jQuery("#languages").append(language); } }</script>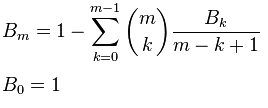

@MorganThrapp The reference implementation is only to make clearer the definition of a Bernoulli number, not to actually solve the problem. – Mego – 2015-11-30T21:04:10.527
Ah, gotcha. I thought it was a fully functional implementation. – Morgan Thrapp – 2015-11-30T21:05:10.993
@MorganThrapp I wrote the code, so it's far from fully functional. :P – Mego – 2015-11-30T21:06:31.857
@FryAmTheEggman `) No, because "decimal digits" refers to digits behind the decimal point. I'll clarify in the question. 2) Sure. – Mego – 2015-11-30T21:43:10.800
2@Mego No standard float (not even quad precision) can store B_60 to quad precision. Should we then use an extended precision format if we're outputting as decimals? – lirtosiast – 2015-11-30T22:39:27.240
@ThomasKwa A double (64-bit float) should be able to represent
-2.139995e34without much trouble. The way I read the rules, it would only really be a problem if you're not using scientific notation for the output. Getting 6 digits after the decimal point would indeed require a lot of precision if you don't write the number in scientific notation. – Reto Koradi – 2015-12-01T00:47:13.827@ThomasKwa You should use whatever is necessary to get the output in the required precision. – Mego – 2015-12-01T03:11:15.853
8I don't like the precision requirement. Some languages don't have the tools to work with floats to sufficient accuracy for B_60, and I'd rather not deal with such issues when golfing a mathematical problem. It's frustrating to write up a solution and then find that it's invalid due to what seems like a technicality. – xnor – 2015-12-01T03:28:46.283
There is a slight trap in that even if you use the exact denominators and integer rounding to reduce errors in the intermediate Bernoulli numbers to 1ulp, the naïve binomial-weighted sum has so much cancellation that things go wildly wrong. – Peter Taylor – 2015-12-02T12:18:35.277
@xnor That will always be an issue with most mathematics-based challenges. That does not mean they shouldn't be posted. – Mego – 2015-12-03T03:11:09.800
@Mego I'm not saying math challenges shouldn't be posted, just that I'd prefer more lax rules about precision. – xnor – 2015-12-03T05:58:07.767
2@xnor 6 digits of accuracy seems incredibly lax already. – primo – 2015-12-03T12:58:03.633