Watkins snark

In the mathematical field of graph theory, the Watkins snark is a snark with 50 vertices and 75 edges.[1][2] It was discovered by John J. Watkins in 1989.[3]

Watkins snark
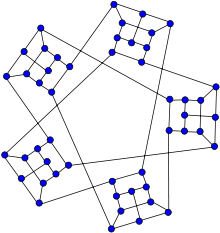The Watkins snark
Named afterJ. J. Watkins
Vertices50
Edges75
Radius7
Diameter7
Girth5
Automorphisms5
Chromatic number3
Chromatic index4
Book thickness3
Queue number2
PropertiesSnark
Table of graphs and parameters

As a snark, the Watkins graph is a connected, bridgeless cubic graph with chromatic index equal to 4. The Watkins snark is also non-planar and non-hamiltonian. It has book thickness 3 and queue number 2.[4]

Another well known snark on 50 vertices is the Szekeres snark, the fifth known snark, discovered by George Szekeres in 1973.[5]

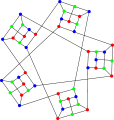  • The chromatic number of the Watkins snark is 3.
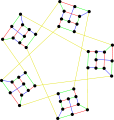  • The chromatic index of the Watkins snark is 4.

Edges

[[1,2], [1,4], [1,15], [2,3], [2,8], [3,6], [3,37], [4,6], [4,7], [5,10], [5,11], [5,22], [6,9], [7,8], [7,12], [8,9], [9,14], [10,13], [10,17], [11,16], [11,18], [12,14], [12,33], [13,15], [13,16], [14,20], [15,21], [16,19], [17,18], [17,19], [18,30], [19,21], [20,24], [20,26], [21,50], [22,23], [22,27], [23,24], [23,25], [24,29], [25,26], [25,28], [26,31], [27,28], [27,48], [28,29], [29,31], [30,32], [30,36], [31,36], [32,34], [32,35], [33,34], [33,40], [34,41], [35,38], [35,40], [36,38], [37,39], [37,42], [38,41], [39,44], [39,46], [40,46], [41,46], [42,43], [42,45], [43,44], [43,49], [44,47], [45,47], [45,48], [47,50], [48,49], [49,50]]

gollark: Yes, Macron has its own internal database system outperforming everything else in existence.
gollark: Explicitness isn't necessarily a good thing as foolish Go programmers claim.
gollark: I have reached a decision: I will ignore the race condition for now 😎.
gollark: Replaced retroactively in the past, yes.
gollark: Not really. Those particular implementations were in C. If C were replaced with another language, similar things would probably exist if there was demand.

References
  1. Weisstein, Eric W. "Watkins Snark". MathWorld.
  2. Watkins, J. J. and Wilson, R. J. "A Survey of Snarks." In Graph Theory, Combinatorics, and Applications (Ed. Y. Alavi, G. Chartrand, O. R. Oellermann, and A. J. Schwenk). New York: Wiley, pp. 1129-1144, 1991
  3. Watkins, J. J. "Snarks." Ann. New York Acad. Sci. 576, 606-622, 1989.
  4. Wolz, Jessica; Engineering Linear Layouts with SAT. Master Thesis, University of Tübingen, 2018
  5. Szekeres, G. (1973). "Polyhedral decompositions of cubic graphs". Bull. Austral. Math. Soc. 8 (03): 367–387. doi:10.1017/S0004972700042660.


This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.