Stoneley wave
A Stoneley wave is a boundary wave (or interface wave) that typically propagates along a solid-solid interface.[2] When found at a liquid-solid interface, this wave is also referred to as a Scholte wave.[3] The wave is of maximum intensity at the interface and decreases exponentially away from it. It is named after the British seismologist Dr. Robert Stoneley (1894–1976), Emeritus Professor of Seismology at the University of Cambridge, who discovered it on October 1, 1924.[4]
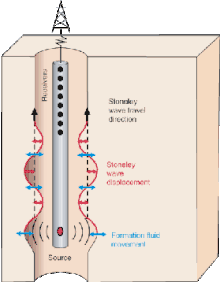
Occurrence and use
Stoneley waves are most commonly generated during borehole sonic logging and vertical seismic profiling. They propagate along the walls of a fluid-filled borehole. They make up a large part of the low-frequency component of the signal from the seismic source and their attenuation is sensitive to fractures and formation permeability. Therefore, analysis of Stoneley waves can make it possible to estimate these rock properties. The standard data processing of sonic logs to derive wave velocity and energy content is explained in[5] and.[6]
Comparison to other waves
| Wave Types in Solids | Particle Vibrations |
| Longitudinal | Parallel to wave direction |
| Transverse (Shear) | Perpendicular to wave direction |
| Surface - Rayleigh | Elliptical orbit - symmetrical mode |
| Plate Wave – Lamb | Component perpendicular to surface (extensional wave) |
| Plate Wave – Love | Parallel to plane layer, perpendicular to wave direction |
| Stoneley (Leaky Rayleigh Waves) | Wave guided along interface |
| Sezawa | Antisymmetric mode |
Effects of Permeability on Stoneley Wave Propagation
Permeability can influence Stoneley wave propagation in three ways. Stoneley waves can be partly reflected at sharp impedance contrasts such as fractures, lithology, or borehole diameter changes. Moreover, as formation permeability increases, Stoneley wave velocity decreases, thereby inducing dispersion. The third effect is the attenuation of Stoneley waves.[8]
References
- "Figure F3. Stoneley wave motion (figure after Qobi et al., 2001)".
- Sheriff, Robert E. (2002). Encyclopedic Dictionary of Applied Geophysics. Society of Exploration Geophysicists. ISBN 978-1-56080-118-4.
- http://downloads.hindawi.com/journals/jam/2012/313207.pdf Rayleigh's, Stoneley's, and Scholte's Interface Waves in Elastic Models Using a Boundary Element Method, Esteban Flores-Mendez,Manuel Carbajal-Romero, Norberto Flores-Guzmán, Ricardo Sánchez-Martínez and Alejandro Rodríguez-Castellanos
- Stoneley, R. (October 1, 1924). "Elastic waves at the surface of separation of two solids". Proc. R. Soc. Lond. A. 106 (738): 416–428. Bibcode:1924RSPSA.106..416S. doi:10.1098/rspa.1924.0079.
- http://www.slb.com/~/media/Files/resources/oilfield_review/ors06/spr06/03_borehole_acoustic_waves.pdf Schlumberger Oilfield Glossary – Borehole Acoustic Waves
- "Introduction".
- http://www.ndt-ed.org/EducationResources/CommunityCollege/Ultrasonics/Physics/modepropagation.htm
- "Method: Aquistion and Processing of Acoustic Waves in Boreholes".