Period-doubling bifurcation

In mathematics, a period doubling bifurcation in a discrete dynamical system is a bifurcation in which a slight change in a parameter value in the system's equations leads to the system switching to a new behavior with twice the period of the original system. With the doubled period, it takes twice as many iterations as before for the numerical values visited by the system to repeat themselves.

A period doubling cascade is a sequence of doublings and further doublings of the repeating period, as the parameter is adjusted further and further.

Period doubling bifurcations can also occur in continuous dynamical systems, namely when a new limit cycle emerges from an existing limit cycle, and the period of the new limit cycle is twice that of the old one.

Examples

Logistic map

Bifurcation diagram for the logistic map. It shows the attractor values, like ${\displaystyle x_{*}}$ and ${\displaystyle x'_{*}}$, as a function of the parameter ${\displaystyle r}$.

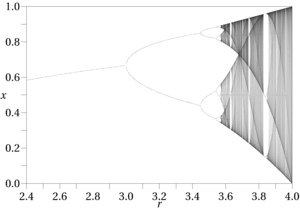
Consider the following simple dynamics: ${\displaystyle x_{n+1}=rx_{n}(1-x_{n})}$ where ${\displaystyle x_{n}}$, the value of ${\displaystyle x}$ at time ${\displaystyle n}$, lies in the ${\displaystyle [0,1]}$ interval and changes over time according to the parameter ${\displaystyle r\in (0,4]}$. This classic example is a simplified version of the logistic map.

For ${\displaystyle r}$ between 1 and 3, ${\displaystyle x_{n}}$ converges to the stable fixed point ${\displaystyle x_{*}=(r-1)/r}$. Then, for ${\displaystyle r}$ between 3 and 3.44949, ${\displaystyle x_{n}}$ converges to a permanent oscillation between two values ${\displaystyle x_{*}}$ and ${\displaystyle x'_{*}}$ that depend on ${\displaystyle r}$. As ${\displaystyle r}$ grows larger, oscillations between 4 values, then 8, 16, 32, etc. appear. These period-doublings culminate at ${\displaystyle r\approx 3.56995}$ from where more complex regimes appear. As ${\displaystyle r}$ increases, there are some intervals where most starting values will converge to one or a small number of stable oscillations, such as near ${\displaystyle r=3.83}$. See figure.

In the interval where the period is ${\displaystyle 2^{n}}$ for some positive integer ${\displaystyle n}$, not all the points actually have period ${\displaystyle 2^{n}}$. These are single points, rather than intervals. These points are said to be in unstable orbits, since nearby points do not approach the same orbit as them. See Sharkovskii's theorem.

Logistical map for a modified Phillips curve

Bifurcation diagram for the modified Phillips curve.

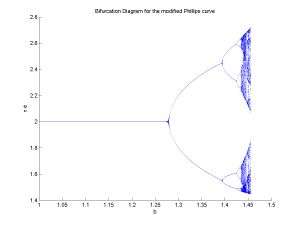
Consider the following logistical map for a modified Phillips curve:

${\displaystyle \pi _{t}=f(u_{t})+b\pi _{t}^{e}}$

${\displaystyle \pi _{t+1}=\pi _{t}^{e}+c(\pi _{t}-\pi _{t}^{e})}$

${\displaystyle f(u)=\beta _{1}+\beta _{2}e^{-u}\,}$

${\displaystyle b>0,0\leq c\leq 1,{\frac {df}{du}}<0}$

where :

• ${\displaystyle \pi }$ is the actual inflation
• ${\displaystyle \pi ^{e}}$ is the expected inflation,
• u is the level of unemployment,
• ${\displaystyle m-\pi }$ is the money supply growth rate.

Keeping ${\displaystyle \beta _{1}=-2.5,\ \beta _{2}=20,\ c=0.75}$ and varying ${\displaystyle b}$, the system undergoes period doubling bifurcations, and after a point becomes chaotic, as illustrated in the bifurcation diagram on the right.

Complex quadratic map

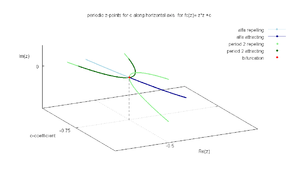
Bifurcation from period 1 to 2 for complex quadratic map

Period doubling cascade in an exponential mapping of the Mandelbrot set

Period-halving bifurcation

Period-halving bifurcations (L) leading to order, followed by period doubling bifurcations (R) leading to chaos.

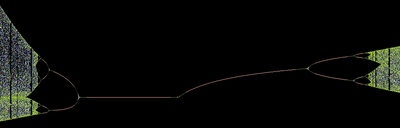
A period halving bifurcation in a dynamical system is a bifurcation in which the system switches to a new behavior with half the period of the original system. A series of period-halving bifurcations leads the system from chaos to order.

See also

• Feigenbaum constants

References

• Kuznetsov, Yuri A. (2004). Elements of Applied Bifurcation Theory. Applied Mathematical Sciences. 112 (3rd ed.). Springer-Verlag. ISBN 0-387-21906-4. Zbl 1082.37002.

External links

• Connecting period-doubling cascades to chaos