Schläfli orthoscheme

In geometry, Schläfli orthoscheme is a type of simplex. They are defined by a sequence of edges ${\displaystyle (v_{0}v_{1}),(v_{1}v_{2}),\dots ,(v_{d-1}v_{d})\,}$ that are mutually orthogonal. These were introduced by Ludwig Schläfli, who called them orthoschemes and studied their volume in the Euclidean, Lobachevsky and the spherical geometry. H. S. M. Coxeter later named them after Schläfli.[1] J.-P. Sydler and Børge Jessen studied them extensively in connection with Hilbert's third problem.

Orthoschemes, also called path-simplices in the applied mathematics literature, are a special case of a more general class of simplices studied by Fiedler (1957),[2] and later rediscovered by Coxeter (1991).[1] These simplices are the convex hulls of trees in which all edges are mutually perpendicular. In the orthoscheme, the underlying tree is a path. In three dimensions, an orthoscheme is also called a birectangular tetrahedron.

Properties

A cube dissected into six orthoschemes.

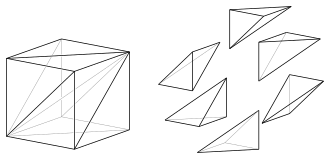
• All 2-faces are right triangles.
• All facets of a d-dimensional orthoscheme are (d − 1)-dimensional orthoschemes.
• The midpoint of the longest edge is the center of the circumscribed sphere.
• The case when ${\displaystyle |v_{0}v_{1}|=|v_{1}v_{2}|=\cdots =|v_{d-1}v_{d}|}$ is a generalized Hill tetrahedron.
• In 3- and 4-dimensional Euclidean space, every convex polytope is scissor congruent to an orthoscheme.
• Every hypercube in d-dimensional space can be dissected into d! congruent orthoschemes. A similar dissection into the same number of orthoschemes applies more generally to every hyperrectangle but in this case the orthoschemes may not be congruent.
• In 3-dimensional hyperbolic and spherical spaces, the volume of orthoschemes can be expressed in terms of the Lobachevsky function, or in terms of dilogarithms.[3]

Dissection into orthoschemes

Unsolved problem in mathematics: Can every simplex be dissected into a bounded number of orthoschemes? (more unsolved problems in mathematics)

Main article: Dissection into orthoschemes

Hugo Hadwiger conjectured in 1956 that every simplex can be dissected into finitely many orthoschemes.[4] The conjecture has been proven in spaces of five or fewer dimensions,[5] but remains unsolved in higher dimensions.[6]

See also

• Goursat tetrahedron
• Order polytope

References

1. Coxeter, H. S. M. (1991), "Orthogonal trees", Proc. 7th ACM Symp. Computational Geometry, pp. 89–97
2. Fiedler, M. (1957), "Über qualitative Winkeleigenschaften der Simplexe", Czechoslovak Math. J., 7: 463–478
3. Vinberg, E. B. (1993), "Volumes of non-Euclidean polyhedra", Russian Math. Surveys, 48:2: 15–45, doi:10.1070/rm1993v048n02abeh001011
4. Hadwiger, Hugo (1956), "Ungelöste Probleme", Elemente der Mathematik, 11: 109–110
5. Tschirpke, Katrin (1994), "The dissection of five-dimensional simplices into orthoschemes", Beiträge zur Algebra und Geometrie, 35 (1): 1–11, MR 1287191
6. Brandts, Jan; Korotov, Sergey; Křížek, Michal; Šolc, Jakub (2009), "On nonobtuse simplicial partitions" (PDF), SIAM Review, 51 (2): 317–335, doi:10.1137/060669073, MR 2505583. See in particular Conjecture 23, p. 327.