Lebesgue's universal covering problem

Lebesgue's universal covering problem is an unsolved problem in geometry that asks for the convex shape of smallest area that can cover any planar set of diameter one. The diameter of a set by definition is the least upper bound of the distances between all pairs of points in the set. A shape covers a set if it contains a congruent subset. In other words the set may be rotated, translated or reflected to fit inside the shape.

Unsolved problem in mathematics: What is the minimum area of a convex shape that can cover every planar set of diameter one? (more unsolved problems in mathematics)

An equilateral triangle of diameter 1 doesn’t fit inside a circle of diameter 1

For other uses of "universal cover" or "universal covering", see Covering space § Universal covers.

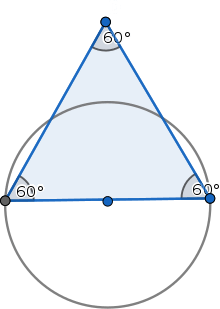
The problem was posed by Henri Lebesgue in a letter to Gyula Pál in 1914. It was published in a paper by Pál in 1920 along with Pál's analysis.[1] He showed that a cover for all curves of constant width one is also a cover for all sets of diameter one and that a cover can be constructed by taking a regular hexagon with an inscribed circle of diameter one and removing two corners from the hexagon to give a cover of area ${\displaystyle 2-{\frac {2}{\sqrt {3}}}\approx 0.84529946}$.

The shape outlined in black is Pál's solution to Lebesgue's universal covering problem. Within it, planar shapes with diameter one have been included: a circle (in blue), a Reuleaux triangle (in red) and a square (in green).

Known bounds

In 1936 Roland Sprague showed that a part of Pál's cover could be removed near one of the other corners while still retaining its property as a cover.[2] This reduced the upper bound on the area to ${\displaystyle a\leq 0.844137708436}$. In 1992 Hansen showed that two more very small regions of Sprague's solution could be removed bringing the upper bound down to ${\displaystyle a\leq 0.844137708398}$. Hansen's construction was the first to make use of the freedom to use reflections.[3] In 2015 John Baez, Karine Bagdasaryan and Philip Gibbs showed that if the corners removed in Pál's cover are cut off at a different angle then it is possible to reduce the area further giving an upper bound of ${\displaystyle a\leq 0.8441153}$.[4] In October 2018 Philip Gibbs published a paper on arXiv using high-school geometry and claiming a further reduction to 0.8440935944.[5][6]

The best known lower bound for the area was provided by Peter Brass and Mehrbod Sharifi using a combination of three shapes in optimal alignment giving ${\displaystyle a\geq 0.832}$.[7]

See also

• Moser's worm problem, what is the minimum area of a shape that can cover every unit-length curve?
• Moving sofa problem, the problem of finding a maximum-area shape that can be rotated and translated through an L-shaped corridor
• Kakeya set, a set of minimal area that can accommodate every unit-length line segment (with translations allowed, but not rotations)

References

1. Pál, J. (1920). "'Über ein elementares Variationsproblem". Danske Mat.-Fys. Meddelelser III. 2.
2. Sprague, R. (1936). "Über ein elementares Variationsproblem". Matematiska Tidsskrift Ser. B: 96–99. JSTOR 24530328.
3. Hansen, H. C. (1992). "Small universal covers for sets of unit diameter". Geometriae Dedicata. 42: 205–213. doi:10.1007/BF00147549. MR 1163713.
4. Baez, John C.; Bagdasaryan, Karine; Gibbs, Philip (2015). "The Lebesgue universal covering problem". Journal of Computational Geometry. 6: 288–299. doi:10.20382/jocg.v6i1a12. MR 3400942.
5. Gibbs, Philip (23 October 2018). "An Upper Bound for Lebesgue's Covering Problem". arXiv:1810.10089.
6. "Amateur Mathematician Finds Smallest Universal Cover". Quanta Magazine. Archived from the original on 2019-01-14. Retrieved 2018-11-16.
7. Brass, Peter; Sharifi, Mehrbod (2005). "A lower bound for Lebesgue's universal cover problem". International Journal of Computational Geometry and Applications. 15 (5): 537–544. doi:10.1142/S0218195905001828. MR 2176049.