Kozai mechanism

In celestial mechanics, the Kozai mechanism or Lidov–Kozai mechanism or Kozai–Lidov mechanism, also known as the Kozai, Lidov–Kozai or Kozai–Lidov effect, oscillations, cycles or resonance, is a dynamical phenomenon affecting the orbit of a binary system perturbed by a distant third body under certain conditions, causing the orbit's argument of pericenter to oscillate about a constant value, which in turn leads to a periodic exchange between its eccentricity and inclination. The process occurs on timescales much longer than the orbital periods. It can drive an initially near-circular orbit to arbitrarily high eccentricity, and flip an initially moderately inclined orbit between a prograde and a retrograde motion.

The effect has been found to be an important factor shaping the orbits of irregular satellites of the planets, trans-Neptunian objects, extrasolar planets, and multiple star systems.[1] It is hypothesized to enable black hole mergers.[2] It was first described in 1961 by Mikhail Lidov while analyzing the orbits of artificial and natural satellites of planets.[3] In 1962, Yoshihide Kozai published this same result in application to the orbits of asteroids perturbed by Jupiter.[4] The citations of the initial papers by Kozai and Lidov have risen sharply in the 21st century. As of 2017, the mechanism is among the most studied astrophysical phenomena.[5]

Background

Hamiltonian mechanics

Main article: Hamiltonian mechanics

In Hamiltonian mechanics, a physical system is specified by a function, called Hamiltonian and denoted ${\textstyle {\mathcal {H}}}$, of canonical coordinates in phase space. The canonical coordinates consist of the generalized coordinates ${\textstyle x_{i}}$ in configuration space and their conjugate momenta ${\textstyle p_{i}}$. The number of ${\textstyle (x_{i},p_{i})}$ pairs required to describe a given system is the number of its degrees of freedom. The coordinates are usually chosen in such a way as to simplify the calculations involved in solving a particular problem. One set of canonical coordinates can be changed to another by a canonical transformation. The equations of motion for the system are obtained from the Hamiltonian through Hamilton's canonical equations, which relate time derivatives of the coordinates to partial derivatives of the Hamiltonian with respect to the conjugate momenta.

Orbital elements

Main article: Orbital elements

The Keplerian orbital elements

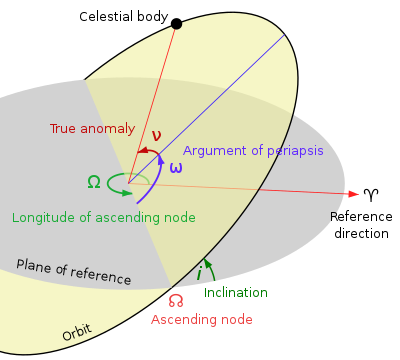
An elliptical orbit in three dimensions is uniquely described by a set of six coordinates, called orbital elements. The traditional choice are the Keplerian elements, which consist of the eccentricity, semimajor axis, inclination, longitude of the ascending node, argument of periapsis, and true anomaly. In celestial mechanics calculations, it is common to use a set of orbital elements introduced in nineteenth century by Charles-Eugène Delaunay.[6] The Delaunay elements form a canonical set of action-angle coordinates and consist of the mean anomaly ${\textstyle l}$, the argument of periapsis ${\textstyle g}$ and the longitude of the ascending node ${\textstyle h}$, along with their conjugate momenta denoted by ${\textstyle L}$, ${\textstyle G}$, and ${\textstyle H}$, respectively.[7]

The three-body problem

Main articles: Three-body problem and Perturbation theory

The dynamics of a system composed of three bodies system acting under their mutual gravitational attraction is complex. In general, the behaviour of a three-body system depends sensitively on the initial conditions. Thus, the three-body problem, the problem of determining the motions of the three bodies, cannot be solved analytically except in special cases.[8] Instead, numerical methods are used.[9]

The Lidov-Kozai mechanism is a feature of hierarchical triple systems,[10] that is systems in which one of the bodies, called the "perturber", is located far from the other two, which are said to comprise the inner binary. The perturber and the centre of mass of the inner binary comprise the outer binary.[11] Such systems are often studied by using the methods of perturbation theory to write the Hamiltonian of a hierarchical three-body system as a sum of two terms responsible for the isolated evolution of the inner and the outer binary, and a third term coupling the two orbits,[12]

${\displaystyle {\mathcal {H}}={\mathcal {H}}_{\rm {in}}+{\mathcal {H}}_{\rm {out}}+{\mathcal {H}}_{\rm {pert}}.}$

The coupling term is then expanded in the orders of parameter ${\textstyle \alpha }$, defined as the ratio of the semi-major axes of the inner and the outer binary and hence small in a hierarchical system.[12] Since the perturbative series converges rapidly, the qualitative behaviour of a hierarchical three-body system is determined by the initial terms in the expansion, referred to as the quadrupole (${\textstyle \propto \alpha ^{2}}$), octupole (${\textstyle \propto \alpha ^{3}}$) and hexadecapole (${\textstyle \propto \alpha ^{4}}$) order terms,[13]

${\displaystyle {\mathcal {H}}_{\rm {pert}}={\mathcal {H}}_{\rm {quad}}+{\mathcal {H}}_{\rm {oct}}+{\mathcal {H}}_{\rm {hex}}+O(\alpha ^{5}).}$

For many systems, a satisfactory description is found already at the lowest, quadrupole order in the perturbative expansion. The octupole term becomes dominant in certain regimes and is responsible for a long-term variation in the amplitude of the Lidov-Kozai oscillations.[14]

Secular approximation

The Lidov-Kozai mechanism is a secular effect, that is, it occurs on timescales much longer compared to the orbital periods of the inner and the outer binary. In order to simplify the problem and make it more tractable computationally, the hierarchical three-body Hamiltonian can be secularised, that is averaged over the rapidly varying mean anomalies of the two orbits. Through this process, the problem is reduced to that of two interacting massive wire loops.[15]

Overview of the mechanism

Test particle limit

The simplest treatment of the Lidov-Kozai mechanism assumes that one of the inner binary's components, the secondary, is a test particle - an idealized point-like object with negligible mass compared to the other two bodies, the primary and the distant perturber. These assumptions are valid, for instance, in the case of an artificial satellite in a low-Earth orbit that is perturbed by the Moon, or a short-period comet that is perturbed by Jupiter.

Under these approximations, the orbit-averaged equations of motion for the secondary have a conserved quantity: the component of the secondary's orbital angular momentum parallel to the angular momentum of the primary/perturber angular momentum. This conserved quantity can be expressed in terms of the secondary's eccentricity e and inclination i relative to the plane of the outer binary:

${\displaystyle L_{z}={\sqrt {(1-e^{2})}}\cos i=\mathrm {const} .}$

Conservation of L_z means that orbital eccentricity can be "traded for" inclination. Thus, near-circular, highly inclined orbits can become very eccentric. Since increasing eccentricity while keeping the semimajor axis constant reduces the distance between the objects at periapsis, this mechanism can cause comets (perturbed by Jupiter) to become sungrazing.

Lidov-Kozai oscillations will be present if L_z is lower than a certain value. At the critical value of L_z, a "fixed-point" orbit appears, with constant inclination given by

${\displaystyle i_{crit}=\arccos \left({\sqrt {\frac {3}{5}}}\right)\approx 39.2^{o}}$

For values of L_z less than this critical value, there is a one-parameter family of orbital solutions having the same L_z but different amounts of variation in e or i. Remarkably, the degree of possible variation in i is independent of the masses involved, which only set the timescale of the oscillations.[16]

Timescale

The basic timescale associated with Kozai oscillations is[17]

${\displaystyle T_{\mathrm {Kozai} }=2\pi {\frac {\sqrt {GM}}{Gm_{2}}}{\frac {a_{2}^{3}}{a^{3/2}}}\left(1-e_{2}^{2}\right)^{3/2}={\frac {P_{2}^{2}}{P}}\left(1-e_{2}^{2}\right)^{3/2}}$

where a indicates semimajor axis, P is orbital period, e is eccentricity and m is mass; variables with subscript "2" refer to the outer (perturber) orbit and variables lacking subscripts refer to the inner orbit; M is the mass of the primary. The period of oscillation of all three variables (e, i, ω – the last being the argument of periapsis) is the same, but depends on how "far" the orbit is from the fixed-point orbit, becoming very long for the separatrix orbit that separates librating orbits from oscillating orbits.

Astrophysical implications

Solar System

The Lidov-Kozai mechanism causes the argument of pericenter (ω) to librate about either 90° or 270°, which is to say that its periapse occurs when the body is farthest from the equatorial plane. This effect is part of the reason that Pluto is dynamically protected from close encounters with Neptune.

The Lidov-Kozai mechanism places restrictions on the orbits possible within a system, for example

• for a regular moon: if the orbit of a planet's moon is highly inclined to the planet's orbit, the eccentricity of the moon's orbit will increase until, at closest approach, the moon is destroyed by tidal forces.
• for irregular satellites: the growing eccentricity will result in a collision with a regular moon, the planet, or alternatively, the growing apocenter may push the satellite outside the Hill sphere. Recently, the Hill-stability radius has been found as a function of satellite inclination, also explains the non-uniform distribution of irregular satellite inclinations.[18]

The mechanism has been invoked in searches for Planet X, hypothetical planets orbiting the Sun beyond the orbit of Neptune.[19]

A number of moons have been found to be in the Lidov-Kozai resonance with their planet, including Jupiter's Carpo and Euporie,[20] Saturn's Kiviuq and Ijiraq,[21] Uranus's Margaret,[22] and Neptune's Sao and Neso.[23]

Some sources identify the Soviet space probe Luna 3 as the first example of an artificial satellite undergoing Lidov-Kozai oscillations. Launched in 1959 into a highly inclined, eccentric, geocentric orbit, it was the first mission to photograph the far side of the Moon. It burned in the Earth's atmosphere after completing eleven revolutions.[24] However, according to Gkolias et al., a different mechanism must have driven the decay of the probe's orbit since the Lidov-Kozai oscillations would have been thwarted by effects due to the oblateness of the Earth's figure.[25]

Extrasolar planets

The Lidov-Kozai mechanism, in combination with tidal friction, is able to produce Hot Jupiters, which are gas giant exoplanets orbiting their stars on tight orbits.[26][27]

Black holes

The mechanism is thought to affect the growth of central black holes in dense star clusters. It also drives the evolution of certain classes of binary black holes[12] and may play a role in enabling black hole mergers.[28]

History and development

The effect was first described in 1961 by the Soviet space scientist Mikhail Lidov while analyzing the orbits of artificial and natural satellites of planets. Originally published in Russian, the result was translated into English in 1962.[3][29] Lidov presented his work at the Conference on General and Applied Problems of Theoretical Astronomy held in Moscow on November 20–25, 1961.[30] Among the participants of that conference was a Japanese astronomer Yoshihide Kozai[30] who soon published this same result, in application to the orbits of the asteroids perturbed by Jupiter.[4] Since Lidov was the first to discover it, many authors use the term Lidov–Kozai mechanism. Many, however, name it Kozai–Lidov or just Kozai mechanism.

The number of citations of the 1961 and 1962 papers by Lidov and Kozai has risen sharply in the 21st century. As of 2017, the Kozai mechanism is among the most studied astrophysical phenomena.[5]

Notes

1. Shevchenko 2017, p. v
2. Tremaine and Yavetz 2014
3. Lidov 1961, 1962
4. Kozai 1962
5. Shevchenko 2016, p. vi
6. Shevchenko 2017, p. 17
7. Shevchenko 2017, pp. 68-69
8. Valtonen 2005, p. 221
9. Musielak and Quarles 2014, p. 2,10
10. Li et al. 2014, p. 86
11. Naoz et al. 2013, sec. I
12. Naoz et al. 2013
13. Naoz 2016, pp. 4-5
14. Katz et al. 2011
15. Naoz 2016, p. 4
16. Merritt 2013
17. Merritt 2013, p. 575
18. Grishin et al. 2017
19. de la Fuente Marcos et al. 2014
20. Brozović and Jacobson 2017
21. Shevchenko 2017, p. 100
22. Brozović and Jacobson 2009
23. Brozović et al. 2011
24. Shevchenko 2017, pp. 9-10
25. Gkolias et al. 2016
26. Fabrycky and Tremaine 2007
27. Naoz et al. 2011
28. Blaes et al. 2002
29. Nakamura and Orchiston 2017, p. 88
30. Grebnikov 1962

References

• Lidov, Mikhail L. (1961). "Эволюция орбит искусственных спутников под воздействием гравитационных возмущений внешних тел". Iskusstvennye Sputniki Zemli (in Russian). 8: 5–45.
• Lidov, Mikhail L. (1962). "The evolution of orbits of artificial satellites of planets under the action of gravitational perturbations of external bodies". Planetary and Space Science. 9 (10): 719–759. Bibcode:1962P&SS....9..719L. doi:10.1016/0032-0633(62)90129-0. (translation of the 1961 paper)
• Lidov, Mikhail L. (1963). "On approximate analysis of the evolution of orbits of artificial satellites". Problems of Motion of Artificial Celestial Bodies. Proceedings of the Conference on General and Practical Topics of Theoretical Astronomy, Held in Moscow on 20–25 November 1961. Publication of the Academy of Sciences of the USSR, Moscow 1963.
• Kozai, Yoshihide (1962). "Secular perturbations of asteroids with high inclination and eccentricity". The Astronomical Journal. 67: 591. Bibcode:1962AJ.....67..591K. doi:10.1086/108790.
• Shevchenko, Ivan I. (2017). "The Lidov-Kozai Effect - Applications in Exoplanet Research and Dynamical Astronomy". Astrophysics and Space Science Library. 441. Cham: Springer International Publishing. doi:10.1007/978-3-319-43522-0. ISBN 978-3-319-43520-6. ISSN 0067-0057.
• Li, Gongjie; Naoz, Smadar; Holman, Matt; Loeb, Abraham (2014). "Chaos in the Test Particle Eccentric Kozai-Lidov Mechanism". The Astrophysical Journal. IOP Publishing. 791 (2): 86. arXiv:1405.0494. Bibcode:2014ApJ...791...86L. doi:10.1088/0004-637x/791/2/86. ISSN 1538-4357.
• Merritt, David (2013). Dynamics and Evolution of Galactic Nuclei. Princeton Series in Astrophysics. Princeton, NJ: Princeton University Press. ISBN 978-0-691-12101-7. OCLC 863632625.
• Brozović, Marina; Jacobson, Robert A. (2017). "The Orbits of Jupiter's Irregular Satellites". The Astronomical Journal. 153:147 (4): 147. Bibcode:2017AJ....153..147B. doi:10.3847/1538-3881/aa5e4d.
• Brozovic, M.; Jacobson, R. A. (2009). "The Orbits of the Outer Uranian Satellites". The Astronomical Journal. 137 (4): 3834–42. Bibcode:2009AJ....137.3834B. doi:10.1088/0004-6256/137/4/3834.
• Brozović, Marina; Jacobson, Robert A.; Sheppard, Scott S. (2011). "The Orbits of Neptune's Outer Sallites". The Astronomical Journal. 141 (4): 135. Bibcode:2011AJ....141..135B. doi:10.1088/0004-6256/141/4/135.
• de la Fuente Marcos, Carlos; de la Fuente Marcos, Raul (2014). "Extreme trans-Neptunian objects and the Kozai mechanism: signalling the presence of trans-Plutonian planets". Monthly Notices of the Royal Astronomical Society: Letters. 443 (1): L59–L63. arXiv:1406.0715. Bibcode:2014MNRAS.443L..59D. doi:10.1093/mnrasl/slu084.
• Katz, Boaz; Dong, Subo; Malhotra, Renu (2011). "Long-Term Cycling of Kozai-Lidov Cycles: Extreme Eccentricities and Inclinations Excited by a Distant Eccentric Perturber". Physical Review Letters. American Physical Society. 107 (18): 181101. arXiv:1106.3340. Bibcode:2011PhRvL.107r1101K. doi:10.1103/PhysRevLett.107.181101. ISSN 0031-9007. PMID 22107620.CS1 maint: ref=harv (link)
• Lithwick, Yoram; Naoz, Smadar (2011). "The Eccentric Kozai Mechanism for a Test Particle". The Astrophysical Journal. IOP Publishing. 742 (2): 94. arXiv:1106.3329. Bibcode:2011ApJ...742...94L. doi:10.1088/0004-637x/742/2/94. ISSN 0004-637X.CS1 maint: ref=harv (link)
• Naoz, Smadar; Farr, Will M.; Lithwick, Yoram; Rasio, Frederic A.; Teyssandier, Jean (2011). "Hot Jupiters from secular planet–planet interactions". Nature. Springer Nature. 473 (7346): 187–189. arXiv:1011.2501. Bibcode:2011Natur.473..187N. doi:10.1038/nature10076. ISSN 0028-0836. PMID 21562558.CS1 maint: ref=harv (link)
• Naoz, Smadar; Farr, Will M.; Lithwick, Yoram; Rasio, Frederic A.; Teyssandier, Jean (2013). "Secular dynamics in hierarchical three-body systems". Monthly Notices of the Royal Astronomical Society. Oxford University Press (OUP). 431 (3): 2155–2171. arXiv:1107.2414. Bibcode:2013MNRAS.431.2155N. doi:10.1093/mnras/stt302. ISSN 1365-2966.CS1 maint: ref=harv (link)
• Valtonen, M. J. (2005). The three-body problem. Cambridge, UK New York: Cambridge University Press. ISBN 978-0-521-85224-1.CS1 maint: ref=harv (link)
• Musielak, Z E; Quarles, B (2014). "The three-body problem". Reports on Progress in Physics. IOP Publishing. 77 (6): 065901. arXiv:1508.02312. Bibcode:2014RPPh...77f5901M. doi:10.1088/0034-4885/77/6/065901. ISSN 0034-4885. PMID 24913140.CS1 maint: ref=harv (link)
• Fabrycky, Daniel; Tremaine, Scott (2007). "Shrinking Binary and Planetary Orbits by Kozai Cycles with Tidal Friction". The Astrophysical Journal. 669 (2): 1298–1315. arXiv:0705.4285. Bibcode:2007ApJ...669.1298F. doi:10.1086/521702. ISSN 0004-637X.CS1 maint: ref=harv (link)
• Blaes, Omer; Lee, Man Hoi; Socrates, Aristotle (2002). "The Kozai Mechanism and the Evolution of Binary Supermassive Black Holes". The Astrophysical Journal. 578 (2): 775–786. arXiv:astro-ph/0203370. Bibcode:2002ApJ...578..775B. doi:10.1086/342655. ISSN 0004-637X.
• Nakamura, Tsuko; Orchiston, Wayne, eds. (2017). "The Emergence of Astrophysics in Asia". Historical & Cultural Astronomy. Cham: Springer International Publishing. doi:10.1007/978-3-319-62082-4. ISBN 978-3-319-62080-0. ISSN 2509-310X.
• Grebnikov, E. A. (1962). "Conference on General and Applied Problems of Theoretical Astronomy". Soviet Astronomy. 6: 440–. Bibcode:1962SvA.....6..440G. ISSN 0038-5301.CS1 maint: ref=harv (link)
• Tremaine, Scott; Yavetz, Tomer D. (2014). "Why do Earth satellites stay up?". American Journal of Physics. American Association of Physics Teachers (AAPT). 82 (8): 769–777. arXiv:1309.5244. Bibcode:2014AmJPh..82..769T. doi:10.1119/1.4874853. ISSN 0002-9505.CS1 maint: ref=harv (link)
• Verrier, P. E.; Evans, N. W. (2009). "High-inclination planets and asteroids in multistellar systems". Monthly Notices of the Royal Astronomical Society. Oxford University Press (OUP). 394 (4): 1721–1726. arXiv:0812.4528. Bibcode:2009MNRAS.394.1721V. doi:10.1111/j.1365-2966.2009.14446.x. ISSN 0035-8711.CS1 maint: ref=harv (link)
• Naoz, Smadar (2016). "The Eccentric Kozai-Lidov Effect and Its Applications". Annual Review of Astronomy and Astrophysics. Annual Reviews. 54 (1): 441–489. arXiv:1601.07175. Bibcode:2016ARA&A..54..441N. doi:10.1146/annurev-astro-081915-023315. ISSN 0066-4146.CS1 maint: ref=harv (link)
• Gkolias, Ioannis; Daquin, Jérôme; Gachet, Fabien; Rosengren, Aaron J. (2016). "From Order to Chaos in Earth Satellite Orbits". The Astronomical Journal. American Astronomical Society. 152 (5): 119. arXiv:1606.04180. Bibcode:2016AJ....152..119G. doi:10.3847/0004-6256/152/5/119. ISSN 1538-3881.CS1 maint: ref=harv (link)
• Grishin, Evgeni; Perets, Hagai B.; Zenati, Yossef; Michaely, Erez (2017). "Generalized Hill-Stability Criteria for Hierarchical Three-Body Systems at Arbitrary Inclinations". Monthly Notices of the Royal Astronomical Society. Oxford University Press (OUP). 466 (1): 276–285. arXiv:1609.05912. Bibcode:2017MNRAS.466..276G. doi:10.1093/mnras/stw3096. ISSN 1365-2966.CS1 maint: ref=harv (link)