Kelvin functions

In applied mathematics, the Kelvin functions ber_ν(x) and bei_ν(x) are the real and imaginary parts, respectively, of

${\displaystyle J_{\nu }\left(xe^{\frac {3\pi i}{4}}\right),\,}$

where x is real, and J_ν(z), is the ν^th order Bessel function of the first kind. Similarly, the functions ker_ν(x) and kei_ν(x) are the real and imaginary parts, respectively, of

${\displaystyle K_{\nu }\left(xe^{\frac {\pi i}{4}}\right),\,}$

where K_ν(z) is the ν^th order modified Bessel function of the second kind.

These functions are named after William Thomson, 1st Baron Kelvin.

While the Kelvin functions are defined as the real and imaginary parts of Bessel functions with x taken to be real, the functions can be analytically continued for complex arguments xe^iφ, 0 ≤ φ < 2π. With the exception of ber_n(x) and bei_n(x) for integral n, the Kelvin functions have a branch point at x = 0.

Below, Γ(z) is the gamma function and ψ(z) is the digamma function.

ber(x)

ber(x) for x between 0 and 20.

${\displaystyle \mathrm {ber} (x)/e^{x/{\sqrt {2}}}}$ for x between 0 and 50.

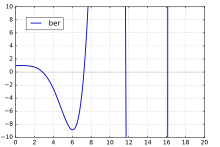
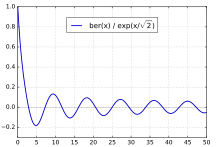
For integers n, ber_n(x) has the series expansion

${\displaystyle \mathrm {ber} _{n}(x)=\left({\frac {x}{2}}\right)^{n}\sum _{k\geq 0}{\frac {\cos \left[\left({\frac {3n}{4}}+{\frac {k}{2}}\right)\pi \right]}{k!\Gamma (n+k+1)}}\left({\frac {x^{2}}{4}}\right)^{k},}$

where Γ(z) is the gamma function. The special case ber₀(x), commonly denoted as just ber(x), has the series expansion

${\displaystyle \mathrm {ber} (x)=1+\sum _{k\geq 1}{\frac {(-1)^{k}}{[(2k)!]^{2}}}\left({\frac {x}{2}}\right)^{4k}}$

and asymptotic series

${\displaystyle \mathrm {ber} (x)\sim {\frac {e^{\frac {x}{\sqrt {2}}}}{\sqrt {2\pi x}}}\left(f_{1}(x)\cos \alpha +g_{1}(x)\sin \alpha \right)-{\frac {\mathrm {kei} (x)}{\pi }}}$,

where

${\displaystyle \alpha ={\frac {x}{\sqrt {2}}}-{\frac {\pi }{8}},}$

${\displaystyle f_{1}(x)=1+\sum _{k\geq 1}{\frac {\cos(k\pi /4)}{k!(8x)^{k}}}\prod _{l=1}^{k}(2l-1)^{2}}$

${\displaystyle g_{1}(x)=\sum _{k\geq 1}{\frac {\sin(k\pi /4)}{k!(8x)^{k}}}\prod _{l=1}^{k}(2l-1)^{2}.}$

bei(x)

bei(x) for x between 0 and 20.

${\displaystyle \mathrm {bei} (x)/e^{x/{\sqrt {2}}}}$ for x between 0 and 50.

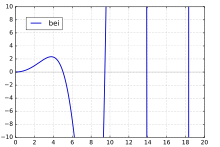
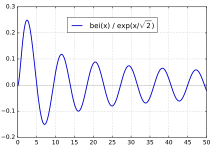
For integers n, bei_n(x) has the series expansion

${\displaystyle \mathrm {bei} _{n}(x)=\left({\frac {x}{2}}\right)^{n}\sum _{k\geq 0}{\frac {\sin \left[\left({\frac {3n}{4}}+{\frac {k}{2}}\right)\pi \right]}{k!\Gamma (n+k+1)}}\left({\frac {x^{2}}{4}}\right)^{k}.}$

The special case bei₀(x), commonly denoted as just bei(x), has the series expansion

${\displaystyle \mathrm {bei} (x)=\sum _{k\geq 0}{\frac {(-1)^{k}}{[(2k+1)!]^{2}}}\left({\frac {x}{2}}\right)^{4k+2}}$

and asymptotic series

${\displaystyle \mathrm {bei} (x)\sim {\frac {e^{\frac {x}{\sqrt {2}}}}{\sqrt {2\pi x}}}[f_{1}(x)\sin \alpha -g_{1}(x)\cos \alpha ]-{\frac {\mathrm {ker} (x)}{\pi }},}$

where α, ${\displaystyle f_{1}(x)}$, and ${\displaystyle g_{1}(x)}$ are defined as for ber(x).

ker(x)

ker(x) for x between 0 and 14.

${\displaystyle \mathrm {ker} (x)e^{x/{\sqrt {2}}}}$ for x between 0 and 50.

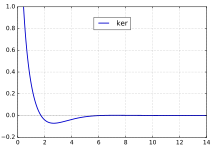
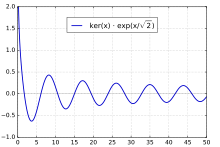
For integers n, ker_n(x) has the (complicated) series expansion

${\displaystyle {\begin{aligned}&\mathrm {ker} _{n}(x)=-\ln \left({\frac {x}{2}}\right)\mathrm {ber} _{n}(x)+{\frac {\pi }{4}}\mathrm {bei} _{n}(x)\\&+{\frac {1}{2}}\left({\frac {x}{2}}\right)^{-n}\sum _{k=0}^{n-1}\cos \left[\left({\frac {3n}{4}}+{\frac {k}{2}}\right)\pi \right]{\frac {(n-k-1)!}{k!}}\left({\frac {x^{2}}{4}}\right)^{k}\\&+{\frac {1}{2}}\left({\frac {x}{2}}\right)^{n}\sum _{k\geq 0}\cos \left[\left({\frac {3n}{4}}+{\frac {k}{2}}\right)\pi \right]{\frac {\psi (k+1)+\psi (n+k+1)}{k!(n+k)!}}\left({\frac {x^{2}}{4}}\right)^{k}.\end{aligned}}}$

The special case ker₀(x), commonly denoted as just ker(x), has the series expansion

${\displaystyle \mathrm {ker} (x)=-\ln \left({\frac {x}{2}}\right)\mathrm {ber} (x)+{\frac {\pi }{4}}\mathrm {bei} (x)+\sum _{k\geq 0}(-1)^{k}{\frac {\psi (2k+1)}{[(2k)!]^{2}}}\left({\frac {x^{2}}{4}}\right)^{2k}}$

and the asymptotic series

${\displaystyle \mathrm {ker} (x)\sim {\sqrt {\frac {\pi }{2x}}}e^{-{\frac {x}{\sqrt {2}}}}[f_{2}(x)\cos \beta +g_{2}(x)\sin \beta ],}$

where

${\displaystyle \beta ={\frac {x}{\sqrt {2}}}+{\frac {\pi }{8}},}$

${\displaystyle f_{2}(x)=1+\sum _{k\geq 1}(-1)^{k}{\frac {\cos(k\pi /4)}{k!(8x)^{k}}}\prod _{l=1}^{k}(2l-1)^{2}}$

${\displaystyle g_{2}(x)=\sum _{k\geq 1}(-1)^{k}{\frac {\sin(k\pi /4)}{k!(8x)^{k}}}\prod _{l=1}^{k}(2l-1)^{2}.}$

kei(x)

kei(x) for x between 0 and 14.

${\displaystyle \mathrm {kei} (x)e^{x/{\sqrt {2}}}}$ for x between 0 and 50.

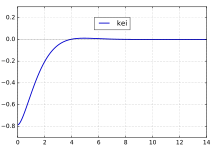
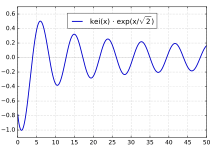
For integer n, kei_n(x) has the series expansion

${\displaystyle {\begin{aligned}&\mathrm {kei} _{n}(x)=-\ln \left({\frac {x}{2}}\right)\mathrm {bei} _{n}(x)-{\frac {\pi }{4}}\mathrm {ber} _{n}(x)\\&-{\frac {1}{2}}\left({\frac {x}{2}}\right)^{-n}\sum _{k=0}^{n-1}\sin \left[\left({\frac {3n}{4}}+{\frac {k}{2}}\right)\pi \right]{\frac {(n-k-1)!}{k!}}\left({\frac {x^{2}}{4}}\right)^{k}\\&+{\frac {1}{2}}\left({\frac {x}{2}}\right)^{n}\sum _{k\geq 0}\sin \left[\left({\frac {3n}{4}}+{\frac {k}{2}}\right)\pi \right]{\frac {\psi (k+1)+\psi (n+k+1)}{k!(n+k)!}}\left({\frac {x^{2}}{4}}\right)^{k}.\end{aligned}}}$

The special case kei₀(x), commonly denoted as just kei(x), has the series expansion

${\displaystyle \mathrm {kei} (x)=-\ln \left({\frac {x}{2}}\right)\mathrm {bei} (x)-{\frac {\pi }{4}}\mathrm {ber} (x)+\sum _{k\geq 0}(-1)^{k}{\frac {\psi (2k+2)}{[(2k+1)!]^{2}}}\left({\frac {x^{2}}{4}}\right)^{2k+1}}$

and the asymptotic series

${\displaystyle \mathrm {kei} (x)\sim -{\sqrt {\frac {\pi }{2x}}}e^{-{\frac {x}{\sqrt {2}}}}[f_{2}(x)\sin \beta +g_{2}(x)\cos \beta ],}$

where β, f₂(x), and g₂(x) are defined as for ker(x).

See also

• Bessel function

References

• Abramowitz, Milton; Stegun, Irene Ann, eds. (1983) [June 1964]. "Chapter 9". Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables. Applied Mathematics Series. 55 (Ninth reprint with additional corrections of tenth original printing with corrections (December 1972); first ed.). Washington D.C.; New York: United States Department of Commerce, National Bureau of Standards; Dover Publications. p. 379. ISBN 978-0-486-61272-0. LCCN 64-60036. MR 0167642. LCCN 65-12253.
• Olver, F. W. J.; Maximon, L. C. (2010), "Bessel functions", in Olver, Frank W. J.; Lozier, Daniel M.; Boisvert, Ronald F.; Clark, Charles W. (eds.), NIST Handbook of Mathematical Functions, Cambridge University Press, ISBN 978-0-521-19225-5, MR 2723248

External links

• Weisstein, Eric W. "Kelvin Functions." From MathWorld—A Wolfram Web Resource.
• GPL-licensed C/C++ source code for calculating Kelvin functions at codecogs.com: