Geometric transformation
In mathematics, a geometric transformation is any bijection of a set to itself (or to another such set) with some salient geometrical underpinning.[1] More specifically, it is a function whose domain and range are sets of points — most often both or both — such that the function is injective so that its inverse exists.[2] The study of geometry may be approached via the study of these transformations.[3]
Geometric transformations can be classified by the dimension of their operand sets (thus distinguishing between, say, planar transformations and spatial transformations). They can also be classified according to the properties they preserve:
- Displacements preserve distances and oriented angles (e.g., translations);[4]
- Isometries preserve angles and distances (e.g., Euclidean transformations);[5][6]
- Similarities preserve angles and ratios between distances (e.g., resizing);[7]
- Affine transformations preserve parallelism (e.g., scaling, shear);[6][8]
- Projective transformations preserve collinearity;[9]
Each of these classes contains the previous one.[9]
- Möbius transformations using complex coordinates on the plane (as well as circle inversion) preserve the set of all lines and circles, but may interchange lines and circles.
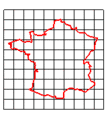 Original image (based on the map of France)
Original image (based on the map of France)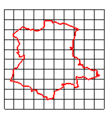
.gif)
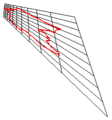 Projective transformation
Projective transformation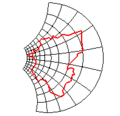 inversion
inversion
- Diffeomorphisms (bidifferentiable transformations) are the transformations that are affine in the first order; they contain the preceding ones as special cases, and can be further refined.[10]
- Conformal transformations preserve angles, and are, in the first order, similarities.
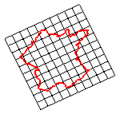
- Equiareal transformations, preserve areas in the planar case or volumes in the three dimensional case.[11] and are, in the first order, affine transformations of determinant 1.
- Homeomorphisms (bicontinuous transformations) preserve the neighborhoods of points.
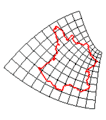 Conformal transformation
Conformal transformation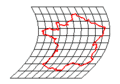
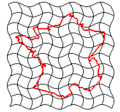
Transformations of the same type form groups that may be sub-groups of other transformation groups.
References
- "The Definitive Glossary of Higher Mathematical Jargon — Transformation". Math Vault. 2019-08-01. Retrieved 2020-05-02.
- Zalman Usiskin, Anthony L. Peressini, Elena Marchisotto – Mathematics for High School Teachers: An Advanced Perspective, page 84.
- Venema, Gerard A. (2006), Foundations of Geometry, Pearson Prentice Hall, p. 285, ISBN 9780131437005
- "Geometry Translation". www.mathsisfun.com. Retrieved 2020-05-02.
- "Geometric Transformations — Euclidean Transformations". pages.mtu.edu. Retrieved 2020-05-02.
- Geometric transformation, p. 131, at Google Books
- "Transformations". www.mathsisfun.com. Retrieved 2020-05-02.
- "Geometric Transformations — Affine Transformations". pages.mtu.edu. Retrieved 2020-05-02.
- Leland Wilkinson, D. Wills, D. Rope, A. Norton, R. Dubbs – Geometric transformation, p. 182, at Google Books
- stevecheng (2013-03-13). "first fundamental form" (PDF). planetmath.org. Retrieved 2014-10-01.
- Geometric transformation, p. 191, at Google Books Bruce E. Meserve – Fundamental Concepts of Geometry, page 191.]
Further reading
| Wikimedia Commons has media related to Transformations (geometry). |
- Adler, Irving (2012) [1966], A New Look at Geometry, Dover, ISBN 978-0-486-49851-5
- Dienes, Z. P.; Golding, E. W. (1967) . Geometry Through Transformations (3 vols.): Geometry of Distortion, Geometry of Congruence, and Groups and Coordinates. New York: Herder and Herder.
- David Gans – Transformations and geometries.
- Hilbert, David; Cohn-Vossen, Stephan (1952). Geometry and the Imagination (2nd ed.). Chelsea. ISBN 0-8284-1087-9.
- John McCleary – Geometry from a Differentiable Viewpoint.
- Modenov, P. S.; Parkhomenko, A. S. (1965) . Geometric Transformations (2 vols.): Euclidean and Affine Transformations, and Projective Transformations. New York: Academic Press.
- A. N. Pressley – Elementary Differential Geometry.
- Yaglom, I. M. (1962, 1968, 1973, 2009) . Geometric Transformations (4 vols.). Random House (I, II & III), MAA (I, II, III & IV).