Fiduccia–Mattheyses algorithm

A classical approach to solve the Hypergraph bipartitioning problem is an iterative heuristic by Fiduccia and Mattheyses.[1] This heuristic is commonly called the FM algorithm.

Introduction

FM algorithm is a linear time heuristic for improving network partitions. New features to K-L heuristic:

  • Aims at reducing net-cut costs; the concept of cutsize is extended to hypergraphs.
  • Only a single vertex is moved across the cut in a single move.
  • Vertices are weighted.
  • Can handle "unbalanced" partitions; a balance factor is introduced.
  • A special data structure is used to select vertices to be moved across the cut to improve running time.
  • Time complexity O(P), where P is the total # of terminals.
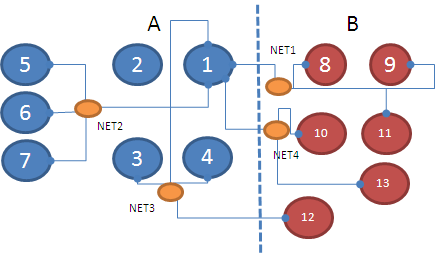
Example of FM

F–M heuristic: notation

Input: A hypergraph with a vertex (cell) set and a hyperedge (net) set

  • n(i): # of cells in Net i; e.g., n(1) = 4
  • s(i): size of Cell i
  • p(i): # of pins of Cell i; e.g., p(1) = 4
  • C: total # of cells; e.g., C = 13
  • N: total # of nets; e.g., N = 4
  • P: total # of pins; P = p(1) + … + p(C) = n(1) + … + n(N)
  • Area ratio r, 0< r<1

Output: 2 partitions

  • Cutsetsize is minimized
  • |A|/(|A|+|B|) ≈ r
gollark: Did you know? Haskell is safe from side channel attacks because its performance is so inconsistent that you can't infer anything from it.
gollark: Hmm, can you do Rowhammer in Haskell?
gollark: And as we know from [1249712481724170249 SIDE CHANNEL ATTACKS] this is a side effect.
gollark: To execute it, state must be stored !!IN MEMORY!!!
gollark: What if ODromedaryCamel?

See also

References
  1. Fiduccia; Mattheyses (1982). "A Linear-Time Heuristic for Improving Network Partitions" (PDF). 19th Design Automation Conference. Retrieved 23 October 2013.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.