Extension of a topological group

In mathematics, more specifically in topological groups, an extension of topological groups, or a topological extension, is a short exact sequence ${\displaystyle 0\to H{\stackrel {\imath }{\to }}X{\stackrel {\pi }{\to }}G\to 0}$ where ${\displaystyle H,X}$ and ${\displaystyle G}$ are topological groups and ${\displaystyle i}$ and ${\displaystyle \pi }$ are continuous homomorphisms which are also open onto their images.[1] Every extension of topological groups is therefore a group extension.

Classification of extensions of topological groups

We say that the topological extensions

${\displaystyle 0\rightarrow H{\stackrel {i}{\rightarrow }}X{\stackrel {\pi }{\rightarrow }}G\rightarrow 0}$

and

${\displaystyle 0\to H{\stackrel {i'}{\rightarrow }}X'{\stackrel {\pi '}{\rightarrow }}G\rightarrow 0}$

are equivalent (or congruent) if there exists a topological isomorphism ${\displaystyle T:X\to X'}$ making commutative the diagram of Figure 1.

Figure 1

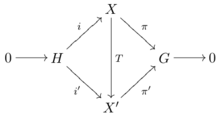
We say that the topological extension

${\displaystyle 0\rightarrow H{\stackrel {i}{\rightarrow }}X{\stackrel {\pi }{\rightarrow }}G\rightarrow 0}$

is a split extension (or splits) if it is equivalent to the trivial extension

${\displaystyle 0\rightarrow H{\stackrel {i_{H}}{\rightarrow }}H\times G{\stackrel {\pi _{G}}{\rightarrow }}G\rightarrow 0}$

where ${\displaystyle i_{H}:H\to H\times G}$ is the natural inclusion over the first factor and ${\displaystyle \pi _{G}:H\times G\to G}$ is the natural projection over the second factor.

It is easy to prove that the topological extension ${\displaystyle 0\rightarrow H{\stackrel {i}{\rightarrow }}X{\stackrel {\pi }{\rightarrow }}G\rightarrow 0}$ splits if and only if there is a continuous homomorphism ${\displaystyle R:X\rightarrow H}$ such that ${\displaystyle R\circ i}$ is the identity map on ${\displaystyle H}$

Note that the topological extension ${\displaystyle 0\rightarrow H{\stackrel {i}{\rightarrow }}X{\stackrel {\pi }{\rightarrow }}G\rightarrow 0}$ splits if and only if the subgroup ${\displaystyle i(H)}$ is a topological direct summand of ${\displaystyle X}$

Examples

• Take ${\displaystyle \mathbb {R} }$ the real numbers and ${\displaystyle \mathbb {Z} }$ the integer numbers. Take ${\displaystyle \imath }$ the natural inclusion and ${\displaystyle \pi }$ the natural projection. Then

${\displaystyle 0\to \mathbb {Z} {\stackrel {\imath }{\to }}\mathbb {R} {\stackrel {\pi }{\to }}\mathbb {R} /\mathbb {Z} \to 0}$

is an extension of topological abelian groups. Indeed it is an example of a non-splitting extension.

Extensions of locally compact abelian groups (LCA)

An extension of topological abelian groups will be a short exact sequence ${\displaystyle 0\to H{\stackrel {\imath }{\to }}X{\stackrel {\pi }{\to }}G\to 0}$ where ${\displaystyle H,X}$ and ${\displaystyle G}$ are locally compact abelian groups and ${\displaystyle i}$ and ${\displaystyle \pi }$ are relatively open continuous homomorphisms.[2]

• Let be an extension of locally compact abelian groups

${\displaystyle 0\to H{\stackrel {\imath }{\to }}X{\stackrel {\pi }{\to }}G\to 0.}$

Take ${\displaystyle H^{\wedge },X^{\wedge }}$ and ${\displaystyle G^{\wedge }}$ the Pontryagin duals of ${\displaystyle H,X}$ and ${\displaystyle G}$ and take ${\displaystyle i^{\wedge }}$ and ${\displaystyle \pi ^{\wedge }}$ the dual maps of ${\displaystyle i}$ and ${\displaystyle \pi }$. Then the sequence

${\displaystyle 0\to G^{\wedge }{\stackrel {\pi ^{\wedge }}{\to }}X^{\wedge }{\stackrel {\imath ^{\wedge }}{\to }}H^{\wedge }\to 0}$

is an extension of locally compact abelian groups.

References

1. Cabello Sánchez, Félix (2003). "Quasi-homomorphisms". Fundam. Math. 178 (3): 255–270. doi:10.4064/fm178-3-5. Zbl 1051.39032.
2. Fulp, R.O.; Griffith, P.A. (1971). "Extensions of locally compact abelian groups. I, II" (PDF). Trans. Am. Math. Soc. 154: 341–356, 357–363. doi:10.1090/S0002-9947-1971-99931-0. MR 0272870. Zbl 0216.34302.