Disclination

In crystallography, a disclination is a line defect in which rotational symmetry is violated.[1] In analogy with dislocations in crystals, the term, disinclination, for liquid crystals first used by Frederick Charles Frank and since then has been modified to its current usage, disclination.[2] It is a defect in the orientation of director whereas a dislocation is a defect in positional order.[3]

Example in two dimensions
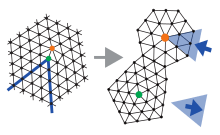
Formation of two disclinations (right) out of a dislocation (left) on an otherwise hexagonal background

In 2D, disclinations and dislocations are point defects instead of line defects as in 3D. They are topological defects and play a central role in melting of 2D crystals within the KTHNY theory, based on two Kosterlitz–Thouless transitions.

Equally sized discs (spheres, particles, atoms) form a hexagonal crystal as dense packing in two dimensions. In such a crystal, each particle has six nearest neighbours. Local strain and twist (for example induced by thermal motion) can cause configurations where discs (or particles) have a coordination number different of six, typically five or seven. Disclinations are topological defects, therefore they can only be created in pairs. This implies that there are - except surface/boarder effects - always as many 5-folded as 7-folded disclinations present in a perfectly plane 2D crystal. A "bound" pair of 5-7-folded disclination is a dislocation. If a lot dislocations are thermally dissociated into isolated disclination, then the monolayer of particles is an isotropic fluid in two dimensions. A 2D crystal is free of disclinations.

For a 7-folded disclination (sketched orange in the figure) a "piece of cake" has to be added (blue triangle), while it is removed for a 5-folded disclination. This visualized why disclinations destroy orientational order, while dislocations only destroy translational order in the far field.

The reason why they are called topological defects is due to the fact that isolated disclinations cannot be created locally by an affine transformation without cutting the hexagonal crystal up to infinity (or at least up to its boarder). The "piece of cake" has  60° in an undisturbed hexagonal crystal. For a 5-folded disclination, it is stretched to  72° and for a 7-folded disclination it is compressed to about  51,4°. Thus, disclination store elastic energy by disturbing the director field.

gollark: Which reminds me, is there some "epoll" equivalent allowing stuff to wait on process termination? Right now it runs a thread for each one, which is bad.
gollark: Yes, praise osmarksßsystemd™.
gollark: ALL are to use osmarksßsystemd™, which is written in Rust, uses TOML for unit files, and is eventually to use SQLite for something or other.
gollark: (it can't actually switch users either; this is a minor detail)
gollark: See, osmarksßsystemd™ doesn't do this.

See also

References
  1. M. Murayama, J. M. Howe, H. Hidaka, S. Takaki. Atomic-Level Observation of Disclination Dipoles in Mechanically Milled, Nanocrystalline Fe. Science 29 (2002) 2433. doi:10.1126/science.1067430
  2. S. Chandrasekhar, Liquid Crystals, p.123, Cambridge University Press 1977, ISBN 0-521-21149-2
  3. . Pure Appl. Chem. 73 (2001) 845.

Further reading
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.