Density (polytope)

In geometry, the density of a star polyhedron is a generalization of the concept of winding number from two dimensions to higher dimensions, representing the number of windings of the polyhedron around the center of symmetry of the polyhedron. It can be determined by passing a ray from the center to infinity, passing only through the facets of the polytope and not through any lower dimensional features, and counting how many facets it passes through. For polyhedra for which this count does not depend on the choice of the ray, and for which the central point is not itself on any facet, the density is given by this count of crossed facets.

The boundary of the regular enneagram {9/4} winds around its centre 4 times, so it has a density of 4.

The same calculation can be performed for any convex polyhedron, even one without symmetries, by choosing any point interior to the polyhedron as its center. For these polyhedra, the density will be 1. More generally, for any non-self-intersecting (acoptic) polyhedron, the density can be computed as 1 by a similar calculation that chooses a ray from an interior point that only passes through facets of the polyhedron, adds one when this ray passes from the interior to the exterior of the polyhedron, and subtracts one when this ray passes from the exterior to the interior of the polyhedron. However, this assignment of signs to crossings does not generally apply to star polyhedra, as they do not have a well-defined interior and exterior.

Tessellations with overlapping faces can similarly define density as the number of coverings of faces over any given point.[1]

Polygons

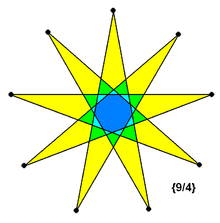
The density of a star polygon is the number of times that the polygonal boundary winds around its center; it is the winding number of the boundary around the central point.

For a regular star polygon {p/q}, the density is q.

It can be visually determined by counting the minimum number of edge crossings of a ray from the center to infinity.

Polyhedra

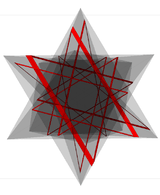The nonconvex great icosahedron, {3,5/2} has a density of 7 as demonstrated in this transparent and cross-sectional view on the right.

Arthur Cayley used density as a way to modify Euler's polyhedron formula (V − E + F = 2) to work for the regular star polyhedra, where d_v is the density of a vertex figure, d_f of a face and D of the polyhedron as a whole:

d_v V − E + d_f F = 2D [2]

For example, the great icosahedron, {3, 5/2}, has 20 triangular faces (d_f = 1), 30 edges and 12 pentagrammic vertex figures (d_v = 2), giving

2·12 − 30 + 1·20 = 14 = 2D.

This implies a density of 7. The unmodified Euler's polyhedron formula fails for the small stellated dodecahedron {5/2, 5} and its dual great dodecahedron {5, 5/2}, for which V − E + F = −6.

The regular star polyhedra exist in two dual pairs, with each figure having the same density as its dual: one pair (small stellated dodecahedron—great dodecahedron) has a density of 3, while the other (great stellated dodecahedron–great icosahedron) has a density of 7.

Hess further generalised the formula for star polyhedra with different kinds of face, some of which may fold backwards over others. The resulting value for density corresponds to the number of times the associated spherical polyhedron covers the sphere.

This allowed Coxeter et al. to determine the densities of the majority of the uniform polyhedra.[3]

For hemipolyhedra, some of whose faces pass through the center, the density cannot be defined. Non-orientable polyhedra also do not have well-defined densities.

4-polytopes

There are 10 regular star 4-polytopes (called the Schläfli–Hess 4-polytopes), which have densities between 4, 6, 20, 66, 76, and 191. They come in dual pairs, with the exception of the self-dual density-6 and density-66 figures.

Notes

1. Coxeter, H. S. M; The Beauty of Geometry: Twelve Essays (1999), Dover Publications, LCCN 99-35678, ISBN 0-486-40919-8 (206–214, Density of regular honeycombs in hyperbolic space)
2. Cromwell, P.; Polyhedra, CUP hbk (1997), pbk. (1999). (Page 258)
3. Coxeter, 1954 (Section 6, Density and Table 7, Uniform polyhedra)

References

• Coxeter, H. S. M.; Regular Polytopes, (3rd edition, 1973), Dover edition, ISBN 0-486-61480-8
• Coxeter, H. S. M.; Longuet-Higgins, M. S.; Miller, J. C. P. (1954), "Uniform polyhedra", Philosophical Transactions of the Royal Society of London. Series A. Mathematical and Physical Sciences, 246 (916): 401–450, doi:10.1098/rsta.1954.0003, ISSN 0080-4614, JSTOR 91532, MR 0062446
• Wenninger, Magnus J. (1979), "An introduction to the notion of polyhedral density", Spherical models, CUP Archive, pp. 132–134, ISBN 978-0-521-22279-2

External links

• Weisstein, Eric W. "Polygon density". MathWorld.