Dasymetric map

The dasymetric map (from Greek δασύς/dasýs "dense" and μέτρο/métro "measure") is a type of thematic map that uses areal symbols to visualize a geographic field by refining a choropleth map with ancillary information about the distribution of the variable.

Comparison of a choropleth map (top) and a dasymetric map (bottom) of the population of the San Francisco Bay Area in 2000.[1]

History
Scrope's 1833 map of world population density, possibly the first dasymetric map.

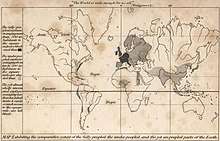
The earliest maps using this kind of approach include an 1833 map of world population density by George Julius Poulett Scrope[2] and an 1838 map of population density in Ireland by Henry Drury Harness, although the methods used to create these maps were never documented.[3][4]

The term "dasymetric" was coined in 1911 by Semenov-Tian-Shansky, who first fully developed and documented the technique, defining them as maps "on which population density, irrespective of any administrative boundaries, is shown as it is distributed in reality, i.e. by natural spots of concentration and rarefaction."[5]. He proposed several methods for improving on choropleth maps, some of which can more properly termed isarithmic maps, but the dasymetric technique he most fully developed and applied is still used today, albeit using digital data and tools such as GIS.

Beyond Russia, the technique was popularized in the 1930s by J.K. Wright, who has sometimes been incorrectly credited with its invention.[6]

Like other forms of thematic mapping, the dasymetric method was created and historically used because of the need for accurate visualization methods of population data. Dasymetric maps are not widely used because of a lack of standardized dasymetric mapping techniques that are accessible to the public. This leads to methods which are highly subjective with inconsistent criteria.[7] Although fields such as public health still rely on choropleth maps, dasymetric maps are becoming more prevalent in developing fields such as aerial interpolation and population estimation using remote sensing.[7]

Methods

Considered a hybrid or compromise between isopleth and choropleth maps,[8][9] a dasymetric map utilizes data aggregated over predetermined geographical districts like a choropleth map, but the boundaries of these districts are modified using ancillary information. A simple and common example is to use data defining regions that are known to be uninhabited, such as water bodies and government-owned land, then cropping these regions out of the choropleth districts, so that they appear empty on the final map. If the variable being mapped is area-dependent (such as population density), the values need to be recalculated by the areas of the refined districts.

Other methods and approaches in dasymetric mapping include areal interpolation, filtered areal weighting using the binary method, filtering with land cover data, and cadastral-based expert dasymetric system.[7][10]

Dasymetric maps are used instead of choropleth maps because they represent underlying data distributions more accurately. Choropleth maps and dasymetric maps differ in three main ways. First, dasymetric zones are generated using ancillary data while boundaries on choropleth maps use units used for more general purposes (such as U.S. county boundaries). Second, choropleth zones have varying levels of internal homogeny while dasymetric maps are designed to be internally homogenous.[11] Last, choropleth mapping methods are standardized while dasymetric methods are under researched.[12]  

gollark: Nvidia push CUDA adoption by having a pretty good AI research team make things which indirectly use CUDA.
gollark: Inertia, and it actually works and Nvidia makes useful things with it.
gollark: CUDA is actually used everywhere for goodish reasons, though.
gollark: Yes.
gollark: It's specific to AMD.

References
  1. "Western Geographic Science Center". Retrieved 13 November 2015.
  2. Scrope, George Julius Poulett (1833). Principles of Political Economy, deduced from the natural laws of social welfare, and applied to the present state of Britain. Longman, Rees, Orme, Brown, Green, Longman.
  3. Harness, Henry D. (1838). Atlas to Accompany the Second Report of the Railway Commissioners, Ireland. H.M.S.O., Dublin.
  4. Robinson, Arthur H. (1955). "The 1837 Maps of Henry Drury Harness" (PDF). The Geographical Journal. 121 (4). pp. 440–450.
  5. Petrov, Andrey (2012). "One Hundred Years of Dasymetric Mapping: Back to the Origin". The Cartographic Journal. 49 (3): 256–264. doi:10.1179/1743277412Y.0000000001.
  6. Wright J.K. (1936). ‘A method of mapping densities of population’, Geographical Review, 26, pp. 103–110.
  7. Lwin, K.K (March 2010). "Development of GIS Tool for Dasymetric Mapping". International Journal of Geoinformatics. 6: 11–18.
  8. "Dasymetric Mapping and Areal Interpolation: Implementation and Evaluation. – Cartography and Geographic Information Science". Accessmylibrary.com. Retrieved 2011-12-14.
  9. http://www.geog.ucsb.edu/~kclarke/G118/Lecture08.html
  10. Maantay, Juliana Astrud; Maroko, Andrew R.; Herrmann, Christopher (2007-01-01). "Mapping Population Distribution in the Urban Environment: The Cadastral-based Expert Dasymetric System (CEDS)". Cartography and Geographic Information Science. 34 (2): 77–102. doi:10.1559/152304007781002190. ISSN 1523-0406. S2CID 10649009.
  11. Mennis, Jeremy (2003). "Generating Surface Models of Population Using Dasymetric Mapping". The Professional Geographer. 55: 31–42. doi:10.1111/0033-0124.10042 (inactive 2020-06-06).
  12. Eicher, Cory L. (2001). "Dasymetric mapping and areal interpolation: Implementation and evaluation". Cartography and Geographic Information Science. 28 (2): 125–138. doi:10.1559/152304001782173727.

Further reading
  • Petrov, A. "Setting the record straight: On the Russian origins of dasymetric mapping." Cartographica, 2008, 44(2)
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.