Contrary (logic)

Contrary is the relationship between two propositions when they cannot both be true (although both may be false). Thus, we can make an immediate inference that if one is true, the other must be false.

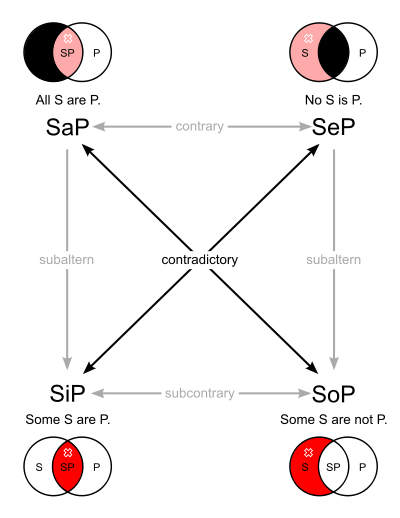
This diagram shows the contrary relationship between categorical propositions in the square of opposition of Aristotelian logic.

The law holds for the A and E propositions of the Aristotelian square of opposition. For example, the A proposition 'every man is honest' and the E proposition 'no man is honest' cannot both be true at the same time, since no one can be honest and not honest at the same time. But both can be false, if some men are honest, and some men are not. For if some men are honest, the proposition 'no man is honest' is false. And if some men are not honest, the proposition 'every man is honest' is false also.

Note that the A and E propositions are contraries only if the A proposition, 'every man is honest', is understood to mean 'there is at least one man and every man is honest', unlike the standard interpretation of the formula of modern logic with the universal quantifier, . Modern presentations of the square of opposition and variants usually make this explicit.[1]

See also
  1. Westerståhl. "Classical vs. modern squares of opposition and beyond" (PDF). Beziau and Payette (eds.), The Square of Opposition, Peter Lang, Bern, 195-229.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.