Brownian surface

A Brownian surface is a fractal surface generated via a fractal elevation function.[1][2][3]

A single realisation of three-dimensional Brownian surface

As with Brownian motion, Brownian surfaces are named after 19th-century biologist Robert Brown.

Example

For instance, in the three-dimensional case, where two variables X and Y are given as coordinates, the elevation function between any two points (x1, y1) and (x2, y2) can be set to have a mean or expected value that increases as the vector distance between (x1, y1) and (x2, y2).[1] There are, however, many ways of defining the elevation function. For instance, the fractional Brownian motion variable may be used, or various rotation functions may be used to achieve more natural looking surfaces.[2]

Generation of fractional Brownian surfaces

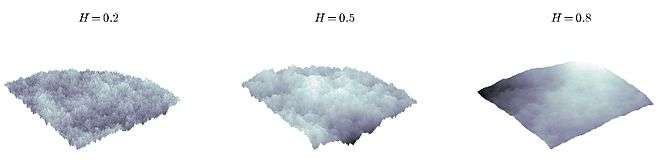
Efficient generation of fractional Brownian surfaces poses significant challenges.[4] Since the Brownian surface represents a Gaussian process with a nonstationary covariance function, one can use the Cholesky decomposition method. A more efficient method is Stein's method,[5] which generates an auxiliary stationary Gaussian process using the circulant embedding approach and then adjusts this auxiliary process to obtain the desired nonstationary Gaussian process. The figure below shows three typical realizations of fractional Brownian surfaces for different values of the roughness or Hurst parameter. The Hurst parameter is always between zero and one, with values closer to one corresponding to smoother surfaces. These surfaces were generated using a Matlab implementation of Stein's method.

Fractional Brownian surfaces for different values of the Hurst parameter. The larger the parameter, the smoother the surface.
gollark: Ah, so the reason your "compiler" is fast is that it's just a simple lazy thing to convert syntax to slightly different forms.
gollark: ... But if it's that simple your interpreter could do that...
gollark: It probably does, though, though they have a penalty due to theirs being more generalized.
gollark: Probably true, but *it's been written by developers better than you are at C*.
gollark: ```3. Python's object model can lead to inefficient memory access```

See also

References
  1. Russ, John C. (1994). Fractal surfaces, Volume 1. p. 167. ISBN 0-306-44702-9.
  2. Xie, Heping (1993). Fractals in rock mechanics. p. 73. ISBN 90-5410-133-4.
  3. Vicsek, Tamás (1992). Fractal growth phenomena. p. 40. ISBN 981-02-0668-2.
  4. Kroese, D.P.; Botev, Z.I. (2015). "Spatial Process Generation". Lectures on Stochastic Geometry, Spatial Statistics and Random Fields, Volume II: Analysis, Modeling and Simulation of Complex Structures, Springer-Verlag, Berlin: 369–404. arXiv:1308.0399. Bibcode:2013arXiv1308.0399K. doi:10.1007/978-3-319-10064-7_12.
  5. Stein, M. L. (2002). "Fast and exact simulation of fractional Brownian motion". Journal of Computational and Graphical Statistics. 11 (3): 587–599. doi:10.1198/106186002466.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.