Boxcar function

In mathematics, a boxcar function is any function which is zero over the entire real line except for a single interval where it is equal to a constant, A.[1] The boxcar function can be expressed in terms of the uniform distribution as

${\displaystyle \operatorname {boxcar} (x)=(b-a)A\,f(a,b;x)=A(H(x-a)-H(x-b)),}$

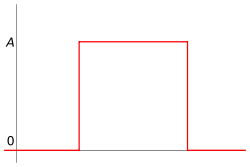
A graphical representation of a boxcar function

where f(a,b;x) is the uniform distribution of x for the interval [a, b] and ${\displaystyle H(x)}$ is the Heaviside step function. As with most such discontinuous functions, there is a question of the value at the transition points. These values are probably best chosen for each individual application.

When a boxcar function is selected as the impulse response of a filter, the result is a moving average filter.

The function is named after its resemblance to a boxcar, a type of railroad car.

See also

• Boxcar averager
• Rectangular function
• Step function
• Top-hat filter

References

1. Weisstein, Eric W. "Boxcar Function". MathWorld. Retrieved 13 September 2013.