Bankoff circle

In geometry, the Bankoff circle or Bankoff triplet circle is a certain Archimedean circle that can be constructed from an arbelos; an Archimedean circle is any circle with area equal to each of Archimedes' twin circles. The Bankoff circle was first constructed by Leon Bankoff in 1974.[1][2][3]

A Bankoff circle with the center C''6

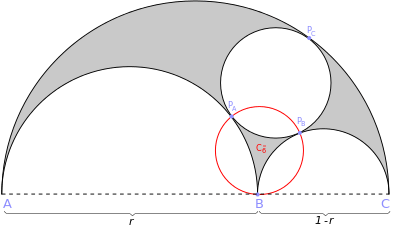
Construction

The Bankoff circle is formed from three semicircles that create an arbelos. A circle C1 is then formed tangent to each of the three semicircles, as an instance of the problem of Apollonius. Another circle C2 is then created, through three points: the two points of tangency of C1 with the smaller two semicircles, and the point where the two smaller semicircles are tangent to each other. C2 is the Bankoff circle.

Radius of the circle

If r = AB/AC, then the radius of the Bankoff circle is:

gollark: .
gollark: Oh, really? Huh
gollark: Just measure it yourself?!
gollark: I wonder if I could get away with NFTs which are just entirely blank transparent PNGs.
gollark: Oh, but Macron is good?

References
  1. Bankoff, L. (1974), "Are the twin circles of Archimedes really twins?", Mathematics Magazine, 47: 214–218, JSTOR 2689213.
  2. Dodge, Clayton W.; Schoch, Thomas; Woo, Peter Y.; Yiu, Paul (1999), "Those ubiquitous Archimedean circles", Mathematics Magazine, 72 (3): 202–213, JSTOR 2690883.
  3. Čerin, Zvonko (2006), "Configurations on centers of Bankoff circles" (PDF), Far East Journal of Mathematical Sciences, 22 (3): 305–320, archived from the original (PDF) on 2011-07-21.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.