Anomalous diffusion

Anomalous diffusion is a diffusion process with a non-linear relationship between the mean squared displacement (MSD), σ_r², and time, in contrast to a typical diffusion process, in which the MSD is a linear function of time. Physically, the MSD can be considered the amount of space the particle has "explored" in the system. An example of anomalous diffusion in nature is the subdiffusion that has been observed in the cell nucleus, plasma membrane and cytoplasm.[1]

Mean squared displacement ${\displaystyle \langle r^{2}(\tau )\rangle }$ for different types of anomalous diffusion

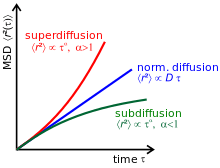
Unlike typical diffusion, anomalous diffusion is described by a power law,[2][3] σ_r² ~ Dt^α, where D is the diffusion coefficient and t is the elapsed time. In a typical diffusion process, α = 1. If α > 1, the phenomenon is called super-diffusion. Super-diffusion can be the result of active cellular transport processes. If α < 1, the particle undergoes sub-diffusion. [4]

The role of anomalous diffusion has received attention within the literature to describe many physical scenarios, most prominently within crowded systems, for example protein diffusion within cells, or diffusion through porous media. Sub-diffusion has been proposed as a measure of macromolecular crowding in the cytoplasm. It has been found that equations describing normal diffusion are not capable of characterizing some complex diffusion processes, for instance, diffusion process in inhomogeneous or heterogeneous medium, e.g. porous media. Fractional diffusion equations were introduced in order to characterize anomalous diffusion phenomena.

Recently, anomalous diffusion was found in several systems including ultra-cold atoms,[5] scalar mixing in the interstellar medium, [6] telomeres in the nucleus of cells,[7] ion channels in the plasma membrane,[8] colloidal particle in the cytoplasm,[9] and worm-like micellar solutions.[10] Anomalous diffusion was also found in other biological systems, including heartbeat intervals and in DNA sequences.[11]

The daily fluctuations of climate variables such as temperature can be regarded as steps of a random walker or diffusion and have been found to be anomalous.[12]

In 1926, using weather balloons, Lewis Richardson demonstrated that the atmosphere exhibits super-diffusion.[13] In a bounded system, the mixing length (which determines the scale of dominant mixing motions) is given by the Von Kármán constant according to the equation ${\displaystyle l_{m}={\kappa }z}$, where ${\displaystyle l_{m}}$ is the mixing length, ${\displaystyle {\kappa }}$ is the Von Kármán constant, and ${\displaystyle z}$ is the distance to the nearest boundary.[14] Because the scale of motions in the atmosphere is not limited, as in rivers or the subsurface, a plume continues to experience larger mixing motions as it increases in size, which also increases its diffusivity, resulting in super-diffusion.[15]

Types of Anomalous diffusion

Of interest within the scientific community, when an anomalous-type diffusion process is discovered, the challenge is to understand the underlying mechanism which causes it. There are a number of frameworks which give rise to anomalous diffusion that are currently in vogue within the statistical physics community. These are long range correlations between the signals [16] continuous-time random walks (CTRW [17]) and fractional Brownian motion (fBm), diffusion of colloidal particles in bacterial suspensions,[18] and diffusion in disordered media.[19][20] Anomalous subdiffusion in cellular cytosol can be an artifact resulting from using of polydisperse probes for measurements.[21]

Hyper-ballistic diffusion

One important class of anomalous diffusion refers to the case when the scaling exponent of the MSD increases with value greater than 2. Such case is called hyper-ballistic diffusion and it has been observed in optical systems[22].

See also

• Lévy flight – random walk with heavy-tailed step lengths
• Random walk
• Percolation
• Long term correlations
• long range dependencies
• Hurst exponent
• Detrended fluctuation analysis (DFA)
• Fractal – Self similar mathematical structures

References

1. Saxton, Michael J. (15 February 2007). "A Biological Interpretation of Transient Anomalous Subdiffusion. I. Qualitative Model". Biophysical Journal. 92 (4): 1178–1191. Bibcode:2007BpJ....92.1178S. doi:10.1529/biophysj.106.092619. PMC 1783867. PMID 17142285.
2. Ben-Avraham, Havlin (2000). Diffusion and Reactions in Fractals and Disordered Systems. Cambridge University Press.
3. S. Havlin, D. ben-Avraham (2002). "Diffusion in disordered media". Adv. Phys. 51 (1): 187–292. Bibcode:2002AdPhy..51..187H. doi:10.1080/00018730110116353.
4. Metzler, Ralf; Jeon, Jae-Hyung; Cherstvy, Andrey G.; Barkai, Eli (2014). "Anomalous diffusion models and their properties: non-stationarity, non-ergodicity, and ageing at the centenary of single particle tracking". Phys. Chem. Chem. Phys. 16 (44): 24128–24164. Bibcode:2014PCCP...1624128M. doi:10.1039/C4CP03465A. ISSN 1463-9076. PMID 25297814.
5. Sagi, Yoav; Brook, Miri; Almog, Ido; Davidson, Nir (2012). "Observation of Anomalous Diffusion and Fractional Self-Similarity in One Dimension". Physical Review Letters. 108 (9): 093002. arXiv:1109.1503. Bibcode:2012PhRvL.108i3002S. doi:10.1103/PhysRevLett.108.093002. ISSN 0031-9007. PMID 22463630.
6. Colbrook, Matthew J.; Ma, Xiangcheng; Hopkins, Philip F.; Squire, Jonathan (2017). "Scaling laws of passive-scalar diffusion in the interstellar medium". Monthly Notices of the Royal Astronomical Society. 467 (2): 2421–2429. arXiv:1610.06590. Bibcode:2017MNRAS.467.2421C. doi:10.1093/mnras/stx261.
7. Bronshtein, Irena; Israel, Yonatan; Kepten, Eldad; Mai, Sabina; Shav-Tal, Yaron; Barkai, Eli; Garini, Yuval (2009). "Transient anomalous diffusion of telomeres in the nucleus of mammalian cells". Physical Review Letters. 103 (1): 018102. Bibcode:2009PhRvL.103a8102B. doi:10.1103/PhysRevLett.103.018102. PMID 19659180.
8. Weigel, Aubrey V.; Simon, Blair; Tamkun, Michael M.; Krapf, Diego (2011-04-19). "Ergodic and nonergodic processes coexist in the plasma membrane as observed by single-molecule tracking". Proceedings of the National Academy of Sciences. 108 (16): 6438–6443. Bibcode:2011PNAS..108.6438W. doi:10.1073/pnas.1016325108. ISSN 0027-8424. PMC 3081000. PMID 21464280.
9. Regner, Benjamin M.; Vučinić, Dejan; Domnisoru, Cristina; Bartol, Thomas M.; Hetzer, Martin W.; Tartakovsky, Daniel M.; Sejnowski, Terrence J. (2013). "Anomalous Diffusion of Single Particles in Cytoplasm". Biophysical Journal. 104 (8): 1652–1660. Bibcode:2013BpJ...104.1652R. doi:10.1016/j.bpj.2013.01.049. ISSN 0006-3495. PMC 3627875. PMID 23601312.
10. Jeon, Jae-Hyung; Leijnse, Natascha; Oddershede, Lene B; Metzler, Ralf (2013). "Anomalous diffusion and power-law relaxation of the time averaged mean squared displacement in worm-like micellar solutions". New Journal of Physics. 15 (4): 045011. Bibcode:2013NJPh...15d5011J. doi:10.1088/1367-2630/15/4/045011. ISSN 1367-2630.
11. Buldyrev, S.V.; Goldberger, A.L.; Havlin, S.; Peng, C.K.; Stanley, H.E. (1994). "Fractals in Biology and Medicine: From DNA to the Heartbeat". In Bunde, Armin; Havlin, Shlomo (eds.). Fractals in Science. Springer. pp. 49–89. ISBN 978-3-540-56220-7.
12. Koscielny-Bunde, Eva; Bunde, Armin; Havlin, Shlomo; Roman, H. Eduardo; Goldreich, Yair; Schellnhuber, Hans-Joachim (1998). "Indication of a Universal Persistence Law Governing Atmospheric Variability". Physical Review Letters. 81 (3): 729–732. Bibcode:1998PhRvL..81..729K. doi:10.1103/PhysRevLett.81.729. ISSN 0031-9007. S2CID 55242320.
13. Richardson, L. F. (1 April 1926). "Atmospheric Diffusion Shown on a Distance-Neighbour Graph". Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences. 110 (756): 709–737. Bibcode:1926RSPSA.110..709R. doi:10.1098/rspa.1926.0043.
14. Cushman-Roisin, Benoit (March 2014). Environmental Fluid Mechanics. New Hampshire: John Wiley & Sons. pp. 145–150. Retrieved 28 April 2017.
15. Berkowicz, Ruwim (1984). "Spectral methods for atmospheric diffusion modeling". Boundary-Layer Meteorology. 30 (1): 201–219. Bibcode:1984BoLMe..30..201B. doi:10.1007/BF00121955.
16. Buldyrev, S.V.; Goldberger, A.L.; Havlin, S.; Peng, C.K.; Stanley, H.E. (1994). "Fractals in Biology and Medicine: From DNA to the Heartbeat". In Bunde, Armin; Havlin, Shlomo (eds.). Fractals in Science. Springer. pp. 49–89. ISBN 978-3-540-56220-7.
17. Masoliver, Jaume; Montero, Miquel; Weiss, George H. (2003). "Continuous-time random-walk model for financial distributions". Physical Review E. 67 (2): 021112. arXiv:cond-mat/0210513. Bibcode:2003PhRvE..67b1112M. doi:10.1103/PhysRevE.67.021112. ISSN 1063-651X. PMID 12636658.
18. Wu, Xiao-Lun (2000-01-01). "Particle Diffusion in a Quasi-Two-Dimensional Bacterial Bath". Physical Review Letters. 84 (13): 3017–3020. Bibcode:2000PhRvL..84.3017W. doi:10.1103/PhysRevLett.84.3017. PMID 11019000. S2CID 27387531.
19. S. Havlin, D. ben-Avraham (2002). "Diffusion in disordered media". Adv. Phys. 51 (1): 187–292. Bibcode:2002AdPhy..51..187H. doi:10.1080/00018730110116353.
20. Toivonen, Matti S.; Onelli, Olimpia D.; Jacucci, Gianni; Lovikka, Ville; Rojas, Orlando J.; Ikkala, Olli; Vignolini, Silvia (13 March 2018). "Anomalous-Diffusion-Assisted Brightness in White Cellulose Nanofibril Membranes". Advanced Materials. 30 (16): 1704050. doi:10.1002/adma.201704050. PMID 29532967.
21. Kalwarczyk, Tomasz; Kwapiszewska, Karina; Szczepanski, Krzysztof; Sozanski, Krzysztof; Szymanski, Jedrzej; Michalska, Bernadeta; Patalas-Krawczyk, Paulina; Duszynski, Jerzy; Holyst, Robert (2017-10-26). "Apparent Anomalous Diffusion in the Cytoplasm of Human Cells: The Effect of Probes' Polydispersity". The Journal of Physical Chemistry B. 121 (42): 9831–9837. doi:10.1021/acs.jpcb.7b07158. ISSN 1520-6106. PMID 28956920.
22. Peccianti, Marco; Morandotti, Roberto (2012). "Beyond ballistic". Nature Physics. 8 (12): 858–859. doi:10.1038/nphys2486.

• Weiss, Matthias; Elsner, Markus; Kartberg, Fredrik; Nilsson, Tommy (2004). "Anomalous Subdiffusion Is a Measure for Cytoplasmic Crowding in Living Cells". Biophysical Journal. 87 (5): 3518–3524. Bibcode:2004BpJ....87.3518W. doi:10.1529/biophysj.104.044263. PMC 1304817. PMID 15339818.
• Bouchaud, Jean-Philippe; Georges, Antoine (1990). "Anomalous diffusion in disordered media". Physics Reports. 195 (4–5): 127–293. Bibcode:1990PhR...195..127B. doi:10.1016/0370-1573(90)90099-N.
• von Kameke, A.; et al. (2010). "Propagation of a chemical wave front in a quasi-two-dimensional superdiffusive flow". Phys. Rev. E. 81 (6): 066211. Bibcode:2010PhRvE..81f6211V. doi:10.1103/physreve.81.066211. PMID 20866505. S2CID 23202701.
• Chen, Wen; Sun, HongGuang; Zhang, Xiaodi; Korosak, Dean (2010). "Anomalous diffusion modeling by fractal and fractional derivatives". Computers and Mathematics with Applications. 59 (5): 1754–1758. doi:10.1016/j.camwa.2009.08.020.
• Sun, HongGuang; Meerschaert, Mark M.; Zhang, Yong; Zhu, Jianting; Chen, Wen (2013). "A fractal Richards' equation to capture the non-Boltzmann scaling of water transport in unsaturated media". Advances in Water Resources. 52: 292–295. Bibcode:2013AdWR...52..292S. doi:10.1016/j.advwatres.2012.11.005. PMC 3686513. PMID 23794783.
• Metzler, Ralf; Jeon, Jae-Hyung; Cherstvy, Andrey G.; Barkai, Eli (2014). "Anomalous diffusion models and their properties: non-stationarity, non-ergodicity, and ageing at the centenary of single particle tracking". Phys. Chem. Chem. Phys. 16 (44): 24128–24164. Bibcode:2014PCCP...1624128M. doi:10.1039/c4cp03465a. ISSN 1463-9076. PMID 25297814.
• Krapf, Diego (2015), "Mechanisms Underlying Anomalous Diffusion in the Plasma Membrane", Lipid Domains, Current Topics in Membranes, 75, Elsevier, pp. 167–207, doi:10.1016/bs.ctm.2015.03.002, ISBN 9780128032954, PMID 26015283, retrieved 2018-08-13

External links

• Boltzmann's transformation, Parabolic law (animation)
• Anomalous interface shift kinetics (Computer simulations and Experiments)