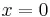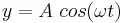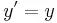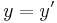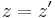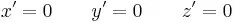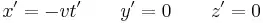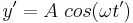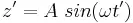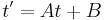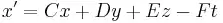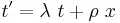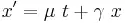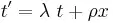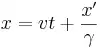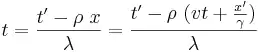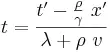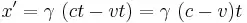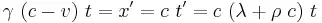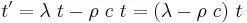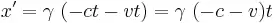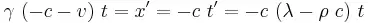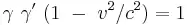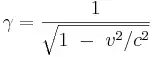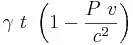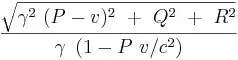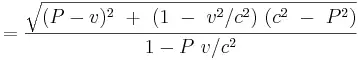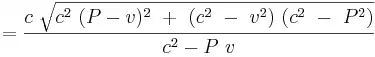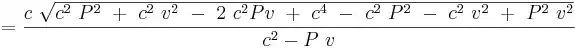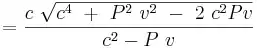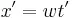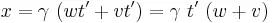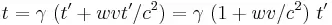Relativity

Relativity is the modern formulation of the meaning of space and time among different observers that are moving relative to each other. Its preliminary form ("Special Relativity") was formulated in 1905 by Albert Einstein, principally in two papers:

• On the Electrodynamics of Moving Bodies (Zur Elektrodynamik bewegter Körper)
• Does the Inertia of a Body Depend on its Energy Content? (Ist die Trägheit eines Körpers von seinem Energiegehalt abhängig?)

It's not rocket science, it's... Astronomy

The Final Frontier

Aristarchus of Samos Astronomy Betelgeuse Doppler effect Iapetus Mercury (planet) Planet SETI Solar System Space Ultimate fate of the Universe Vatican Observatory

The abyss stares back

Giordano Bruno Neil deGrasse Tyson Nicolaus Copernicus Patrick Moore Tycho Brahe v - t - e

“”Why did the road move beneath the chicken?

“”Lights all askew in the heavens, men of science more or less agog over results of eclipse observations.

—November 10, 1919 New York Times headline on the confirmation of General Relativity[1]

The second of these papers contains, though not in the modern form, what is possibly the most famous equation in physics: E=mc².

The later expansion of this, "General Relativity", was formulated by Einstein, in two papers published in 1911, and 1916:

• On the Influence of Gravitation on the Propagation of Light (Über den Einfluss der Schwerkraft auf die Ausbreitung des Lichtes)
• The Foundation of the General Theory of Relativity (Die Grundlage der allgemeinen Relativitätstheorie)

along with other papers by Einstein, Minkowski, Lemaître, Schwarzschild, Weyl, and others.

Relativity was such a radical, abstruse, nonintuitive, and complex phenomenon that, even today, it has a reputation for being difficult to comprehend. The name Einstein has become nearly synonymous with outstanding intellectual prowess. Once the predictions of General Relativity were experimentally confirmed by measuring starlight bending around the sun during a solar eclipse, beginning in 1919, Einstein's name became a household word. In the year 2000, Time Magazine, which had been naming a "Man or Woman of the Year" annually, named Einstein its first "Man of the Century".

In the early decades of the 20^th century, the Theory of Relativity as proposed was revolutionary, in that while it explained previously observed phenomena as well as the Newtonian mechanics it replaced, it also made some seemingly outlandish predictions. As those predictions were tested with newer measurement techniques which confirmed them, the phenomena thus observed became known as "relativistic effects", or just "relativity". These phenomena only come into observable play when dealing with very large masses or velocities — "relativistic velocities".

Galilean relativity

Relativity has its roots in common-sense experience about what time and space mean, and how they are perceived by different observers, including moving observers. Ultimately it derives from the commonplace observation that, when you are in uniform linear motion with respect to your "fixed surroundings" (i.e., not accelerating and therefore can't feel any force), you can't really tell whether you are in motion and the surroundings are fixed, or you are stationary and the surroundings are moving in the other direction. For example, if you are in a car traveling at a speed on a straight and smooth road, you can't "feel" the effects of the motion. You could imagine that the car is stationary, and, when you look out the window, you see telephone poles "moving" past you. Put another way, the question of what is stationary and what is moving is relative—there is no notion of absolute motion or absolute rest.

Of course, in the case of the car, we can make a case for believing that the car is in motion, but in the more general case we can't. For example, the distance between the Milky Way galaxy cluster and the Virgo cluster is increasing at a rate of 10⁶ m / sec. Which one is moving from the other? It doesn't matter.[2]

All of these considerations could be considered to be Galilean Relativity[3] because they are consequences of traditional Galilean/Newtonian mechanics. The way the world works, in terms of motion, velocity, acceleration, momentum, space, and time, comes from the discoveries of Galileo Galilei and Isaac Newton.

First, some ground rules and terminology. We use the term observer and frame of reference (frame for short) interchangeably. A frame of reference is a choice of x, y, and z coordinates and a calibration of time. An observer is simply someone who has chosen such coordinates.

We are only concerned with observers in uniform linear motion. Once the car speeds up, slows down, or goes around a curve, we are no longer oblivious to its motion. A frame of reference in uniform linear motion is called an inertial frame.

For our present purposes we can consider a frame of reference fixed to the Earth to be essentially inertial.[4] It happens that, under General Relativity, that isn't quite true, and the force of gravity arises from the failure to be inertial. But that won't concern us in a treatment of Special Relativity.

Now the fundamental Principle of Relativity, both Galilean and Einsteinian, is this:

All observers, in all states of uniform linear motion with respect to each other, observing the same phenomenon, will agree on whether that phenomenon obeys the laws of physics.

It is fairly straightforward to see this for the fundamental law of Newtonian physics:

F = m a (force equals mass times acceleration)

All observers "obviously" (in Newtonian physics; it will be less straightforward under Relativity) agree on the value of F, all observers "obviously" agree on the value of m (ditto), and all observers agree, but not quite obviously, on the value of a. To see why they agree on the acceleration, consider this: The uniform relative motion of the two observers has the effect of adding some constant velocity (the velocity of the observers' relative motion, in fact) to one observer's measurement of a particle's velocity compared with the other observer's measurement. But acceleration is the time derivative of the velocity, and under Galilean Relativity time is absolute, and adding a constant to something does not affect its derivative.

If one performs an experiment while on a uniformly moving spacecraft, the laws of physics that one observes will be the same as the laws that a "stationary" observer will see.

This is all straightforward and "obvious". So obvious, in fact, that it was not discovered to be wrong until about 1900.

The Galilean Transform

To put relativity on a concrete mathematical basis, consider two observers' measurements of the path of a particle. One observer describes the motion in terms of 4 coordinates—t, x, y, and z—time, and the usual three spatial coordinates. A particle moving around in circles in the y-z plane might be described by

where A is the radius of the circle and ω is the rotation rate.

Now suppose another observer is moving in the x direction at speed v relative to the first observer. Let her four space-time coordinates be t', x', y', and z'. That is, the coordinates have primes on them. We could call the second observer the "primed" one and the first observer the "unprimed" one.

Two frames of reference.

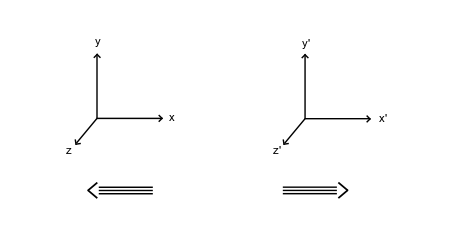
A little thought will show that the primed and unprimed coordinate systems are related by the Galilean Transform:

and the transform going the other way, obtained by simple algebraic equation solving:

The two transforms let each observer calculate her coordinates for the particle in terms of the other observer's coordinates.

To see that this is correct, note that a particle at rest at the origin of the primed frame will have

Using the Galilean Transform to solve this for the unprimed coordinates, we get

So the particle is moving forward in the x direction at speed v in the unprimed system. This is consistent with the idea that the primed system, and everything in it, is moving at speed v relative to the unprimed system.

If a particle is at rest at the origin of the unprimed frame, we will have

Solving this for the primed coordinates, we get

So the primed observer will see the particle moving backward at speed v, as expected. Each observer thinks that she is at rest and the other observer is moving.

We can use the Galilean Transform with the circular motion example given previously. Suppose a carousel-like amusement park ride exists somewhere in "stationary" space, so that a person on the ride (the unprimed frame) undergoes this motion:

Someone on that ride will observe all the usual things—the centrifugal force pushes him against the outer wall, and, if he throws a ball toward the person on the other side, it will be deflected by the Coriolis force.

Now suppose a primed observer is flying past, at speed v, in a spacecraft. Solving, we get

To this observer, the carousel is moving in the negative x direction, as well as turning around. The rider is moving in a helical path. All the positions and velocities will be different from this observer's perspective, but the centrifugal and Coriolis forces will still operate. All observers agree on the correctness of the laws of physics.

Before we leave the Galilean Transform and classical physics, a few more things. Transformations can actually be much more complicated than the simple one we have been using. The coordinate axes can point in random directions, and can have different origins. The time coordinate can be offset. The direction of relative motion could be anything. So the most general form of the Transform might be something like this:

etc.

That would needlessly complicate things, so we will only use transformations of the simple form that we have given. But notice that these equations are linear. We never have or 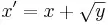. If we did, that would mean that the transformation would vary from place to place or from time to time. Clocks would not tick uniformly, and the laws of physics would vary from one place to another. That just doesn't happen.

Another question that can arise is: What about the observer on the carousel? Does any kind of relativity apply to him? The simple answer is no, since his frame is not inertial. But we will revisit this issue when we get to General Relativity, because there are some interesting things going on.

Modern physics

Galilean Relativity, and the Galilean Transform, are just common sense, so much so that the terms aren't even used except when drawing the comparison with Einsteinian Relativity. More precisely, they are pre-1900 common sense. Toward the end of the 19^th century, people noticed some problems with light, and the speed of light. By then, scientists knew about the connection between light and electrodynamics (Maxwell's equations in particular), and they could measure the speed of light with considerable accuracy. A question arose: What does the speed of light really mean?

If we treat light like everything else, it should travel at a speed determined either by the object that emitted it, or by the medium through which it travels.

The former case would make sense if light were like bullets fired from a gun with a muzzle velocity of 3 x 10⁸ m/s.[5] (By the way, this interpretation would be consistent with Isaac Newton's "corpuscular" theory of light, though it had fallen into disfavor by this time.) Using this model, under Galilean Relativity, if we shine a beam of light out from the front of a spacecraft traveling at 1 x 10⁸ m/s, stationary observers would see the light moving at 4 x 10⁸ m/s. If we shine it out the back, stationary observers would see it traveling at 2 x 10⁸ m/s backward. This would mean that light from distant stars would have speed that differed, either because of the stars' motions (which couldn't be measured) or because of the Earth's motion around the Sun (the effect of which could have been measured.) No such effect was observed. Furthermore, Maxwell's equations say that light travels at a certain speed, which can be deduced from measurable electrical constants, and turns out to be 3 x 10⁸ m/s. Relative to what? Maxwell's equations don't say. It just travels at 3 x 10⁸ m/s.

This brings us to the second possibility, that light always travels at 3 x 10⁸ m/s relative to the medium through which it travels. This was a more acceptable notion, because Maxwell's equations had overturned Newton's "corpuscular" theory and indicated that light was a wave motion.[6] The mechanism of sound waves was well understood. Sound travels through its medium at a speed determined by various physical attributes (density, elasticity) relative to that medium. Of course, this means that, when the emitter or absorber is traveling faster than the speed of sound, there could be strange effects, like modern sonic booms, but the general mechanism was well understood.

So Maxwell's equations made sense: electromagnetic waves travel at 3 x 10⁸ m/s through the medium. The motion of the star that emitted the light doesn't matter—an observer will always get the same speed. But what is the medium? Light can travel through a vacuum. The medium was hypothesized under the name "luminiferous aether", or "aether" for short.

But that didn't solve the problem. Maxwell's equations seem to say that light always travels at 3 x 10⁸ m/s relative to where I am standing now. This, of course, violates Galilean Relativity, that is, common sense. If two observers are in motion relative to each other, observing the same light beam, they can't both think that the aether is stationary relative to themselves. At least one of them (the one that is "really moving"), will sense an "aether breeze" going past him as he travels through the "truly stationary" aether, similar to the phenomenon that dogs enjoy when riding in a car. This means that light will be measured at different speeds by different observers.

This phenomenon was put to the test by the celebrated Michelson-Morley experiment in 1887. If there were a "breeze", the speed would be different in the "upwind" and "downwind" directions. No "breeze" was found. Just in case the universal "stationary" aether just happened to coincide with the Earth's motion around the Sun, the experiment was conducted again 6 months later. No effect was found, and the equipment was sensitive enough that it would have detected the breeze.

Perhaps the Earth locally drags the aether around with it. But there are effects from starlight that could have been observed if that were the case, and they were not observed. In any case, saying that the aether is dragged along is really the same as saying that there is no aether.

The inescapable conclusion is that there is no aether. The speed of light is always 3 x 10⁸ m/s relative to where I am standing now. Even for different observers. Galilean Relativity doesn't work.

Now the solution must involve meter sticks getting shorter and/or clocks going slower—we will work out the details of this presently. But as early as 1889, George FitzGerald proposed that this was what was happening. If a stationary observer watches a spacecraft go by at 90% of the speed of light, and a flashlight at the front of the spacecraft shines a beam of light forward, the observer will see the beam moving relative to the spacecraft at only 10% of the speed of light, while an astronaut on the spacecraft will think it is going at full speed. They can both be right if the stationary observer believes that the astronaut's meter stick got shorter and/or his clock got slower. These effects were known as the Lorentz-FitzGerald contraction. It was believed that some physical compression was actually taking place, and mechanisms were proposed whereby changes in the electric field inside the atoms of the meter stick would cause this.

“” There once was a young man named Fisk Whose fencing was exceedingly brisk So swift was his action The FitzGerald Contraction Reduced his rapier to a disk.

Einsteinian relativity

What Einstein did was to say that the reason the meter stick got shorter is that the meter itself got shorter, and the reason the clock ran slower was that the second got longer. No forces compressed anything. The Galilean transform simply isn't correct. It needs to be replaced by another transform that causes space and time to change.

One thing that this transform must do is provide for the following fundamental principle:

The speed of light, in meters per second, is the same for all observers.

So we need to figure out a transformation rule to replace the Galilean Transform, that obeys that principle. This is the Lorentz Transform. (Einstein himself did not express it quite this way—Hendrik Lorentz did.)

We should point out that this transform doesn't just "fix" light. Nor does it just "fix" things moving at the speed of light. It "fixes" everything, including Michelson and Morley's reference frame, which is, of course, why they got the result that they got. For things that move much slower than the speed of light, the fix is negligible, and the Lorentz Transform reduces to the Galilean Transform.

While the Michelson-Morley experiment is widely taken to be the observational breakthrough that led to Relativity, Einstein did not use it as the basis for his 1905 papers, and said that he had in fact been unaware of it. He based Relativity on Maxwell's equations, which alone are able to overthrow the Galilean Transform.

The Lorentz Transform

We will now derive the Lorentz Transform, which is at the heart of Special Relativity.

A few notes before we begin:

• The speed of light is denoted by c. This is a universally accepted bit of notation.

Also, we will make the same simplifying assumptions that we made earlier regarding the Galilean Transform:

• The two frames are oriented the same way, so that the respective coordinate axes are parallel.
• The motion is purely in the x direction, with velocity v.
• The origin t = x = y = z = 0 in the unprimed systems coincides with the origin t' = x' = y' = z' = 0 in the primed system.

We also make use of the observation that we made above, that the equations must be linear, to prevent the laws of physics from being different at different places or different times. Ignoring the y and z coordinates, the equations must be something like:

For some coefficients λ, ρ, μ, and γ.

Now the origin x' = 0 must be moving at speed v in the unprimed frame, that is, it has x = v t. But

so

so we can remove μ and reduce the equations to:

Next, we work out the reverse transform, by solving the above equations algebraically. We have:

so

Now suppose a light beam goes forward in the unprimed frame. It obeys x = c t. Plugging this into the first set of equations, it also obeys

But x' = c t', since the speed of light is the same in both systems, so

so

Now suppose a light beam goes backward. It obeys x = - c t. It also obeys

But x' = - c t', so

so

Adding equations (1) and (2), we get

Subtracting them, we get

This means we can remove λ and ρ from the equations, leaving only γ. The transform, and its inverse, are:

For some number γ. Assume that the unprimed observer has gone through the calculation above and calculated this number.

Now the primed observer can go through the same calculations, but with the sign of v reversed. She will get this transform, with its inverse:

Now her number γ' might not be the same, because γ may depend on v, and her value of v is the negative of the unprimed observer's value.

Now the two sets of transforms must be the same, so, looking at the two sets of equations, we must have

Are γ and γ' the same number? Yes, because they are the amounts by which each observer thinks the other one's clock has slowed down. By symmetry, each observer must think the other's clock has been affected by the same amount, so

So there's really just one number γ, and we get

where

How about the y and z coordinates? They aren't affected. This can be seen by considering that each observer holds out a meter stick sideways, in the y (or z) direction, as the frames pass each other at x = x' = t  t' = 0

The ends of the meter sticks will exactly pass each other at that instant. If either observer thought the other observer's meter stick were of a different length, by symmetry they would each have to come to the same conclusion about the other. They can't both think the other's meter stick is shorter when the ends are lined up exactly.

So the full Lorentz Transform (under the simplifying assumptions that we have made, specifically that the relative motion is in the x direction at speed v) is:

where

One thing to notice is that, for speeds much slower than the speed of light, γ approaches 1, and the transform approaches the Galilean Transform. (Just let c approach infinity in the above equations to see this.) The effects of relativity are not noticeable in ordinary life.[7]

Things that move at speeds that are a significant fraction of the speed of light are said to be relativistic. Electrons in a CRT display travel at about 0.3 c and are therefore relativistic. Modern particle accelerators can accelerate electrons to over 0.9999999999 c.

Check

Before declaring victory, we should verify that the Lorentz Transform preserves the speed of light in all directions, not just forward or backward.

Suppose a point moves in a straight line from the origin of the unprimed system, in any direction at all. Its unprimed motion is

for some values of P, Q, and R. (P, Q, and R are the components of its velocity vector.)

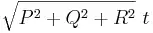The distance traveled at any instant t is , which is . Its speed is 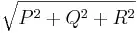. If it is moving at the speed of light in the unprimed system, .

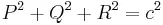Converting to the primed system, we have:

Now the distance traveled in the primed system is , which is

The time taken in the primed system is

So its speed is

Now 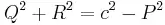, so the speed is

Now, since 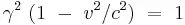, the speed is

as required.

Simultaneity

There is a common-sense notion that the question of simultaneity or non-simultaneity of two events has an absolute reality behind it. Under relativity this isn't true. Suppose, in the primed frame, we have an event at t' = x' = 0 and another event at t' = 0;x' = 1. They are clearly simultaneous to the primed observer. Under the Lorentz Transform, the unprimed observer will see the first event at t = 0, of course, but the second event will be at t = -γv/c², which is not zero unless v is zero.

The violation of simultaneity is perhaps the simplest relativistic effect to demonstrate—one doesn't need to derive the Lorentz Transform. Imagine a moving train, with someone turning on a light in the center of one of the cars. The light will reach the front and back of the car simultaneously, as seen by an observer on the train. But an outside observer, because of the constancy of the speed of light, will see the light hit the back wall first.

Time dilation

Suppose the primed observer has a stationary clock, ticking once per second, at the origin. The first tick is at t' = 0;x' = 0 and the next tick at t' = 1;x' = 0. But the unprimed observer will see the first tick at t = 0;x = 0 and the next one at t = γ;x = γv. The clock moved, obviously, but more importantly, the tick interval has increased from 1 second to γ seconds, that is, seconds.

Each observer thinks that the other one's clock has slowed down.

Length contraction

Suppose the primed observer holds out a meter stick in the direction of motion. The back end is at x' = 0;t' = anything and the front end at x' = 1;t' = anything.

The primed observer photographs it as it goes by, at her time t = 0. The back end is at x = 0;t = 0. The front end must be at x = 1/γ;t = 0. (Check this by transforming back to the primed system, getting x' = 1;t' = -v/c². The primed observer thinks that the photographs were taken at different times.)

So each observer thinks that the other's meter stick has shortened to 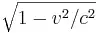meters.

The speed addition formula

If some object is moving forward at speed w in the primed frame, which is itself moving at speed v relative to the unprimed frame, how fast does the unprimed observer think the object is moving? The Galilean answer is w+v, of course. Under Relativity, we have

so

so

so its speed is

If w and v are less than the speed of light, the total will be also.

Moral relativism

For some strange reason, some people think that relativity is linked to moral relativism. No one knows why, but it is very amusing to see them go through verbal and intellectual contortions to justify such a connection. The folks over at Conservapedia even have a page about why it is not true, including instances in the Bible where Jesus performs miracles (bullshit keywords are in bold).

See also

Русскоязычным вариантом данной статьи является статья Теория относительности

• Anti-relativity
• Quantum mechanics
• Deutsche Physik

External links

• Welcome to Einstein Online - relativity and more!
• Einstein's geometric gravity
• Some Results of the Lorentz Transformation Equations
• Some Consequences of E=mc2

References

1. Lights all askew in the heavens November 10, 1919 New York Times
2. To cosmologists such questions do matter, because of such issues as inhomogeneity of the universe and motion through the primordial Cosmic Background Radiation. But these considerations don't concern us here.
3. The terms "Galilean Relativity" and "Galilean Transform" are modern terms, and Galileo certainly never heard them. Nor did he manipulate equations the way his transform does. He is simply being honored as the person whose physical insights gave rise to physics as we know it.
4. But not precisely inertial. A Foucault Pendulum can detect this.
5. In this article, we will use the figure 3 x 10⁸ m/s for the speed of light. Its actual speed is 299792458 m/s
6. But the "corpuscles" would come back, in the form of photons, with the advent of Quantum Mechanics.
7. Or are they? It could be argued that magnetism is just a relativistic consequence of the electric force.

ru:Теория относительности