Tutu - Haskell - (3460 2654 2476 2221 2060 1992 1900 + 50x10 = 2400)
Strategy:
- find A*
- bloat the path with it’s neighbors (distance 2)
- find again A*, but this time in the position+speed space, just like the pighead racer
Golf:
- left out most of the error checking, so the program assumes that there are always start and end points in the map and a path inbetween.
- short variable names
I’m not a Haskell golfer, so I don’t know how much can be further saved (Edit: turned out to be quite a bunch).
Performance:
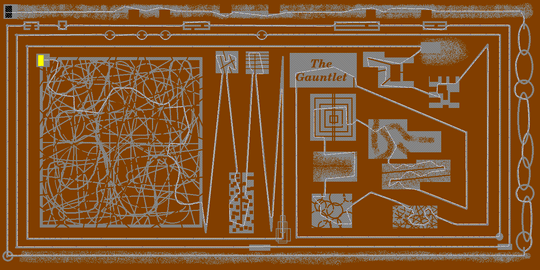
The Gauntlet runs in 9:21min on my 1,7GHz Core i5 from 2011. The City takes 7.2sec. (used -O1 with ghc, -O2 makes the program awfully slow)
Tweaking options are the thickness of the bloated path. For the smaller maps distance 3 saves one or two steps, but The Gauntlet runs too long, so I stay with distance 2. The race starts always at the first (i.e. top left) yellow pixel, optimizing by hand should save an additional step.
Rule conformity:
„You can't use path-finding libraries.“ - I do, but the source is included. The A* search functions are slightly heavily golfed versions of Cale Gibbard’s Data.Graph.AStar library (see http://hackage.haskell.org/package/astar).
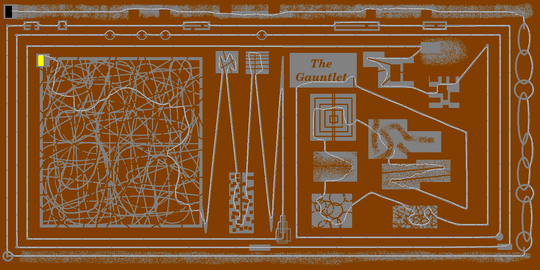
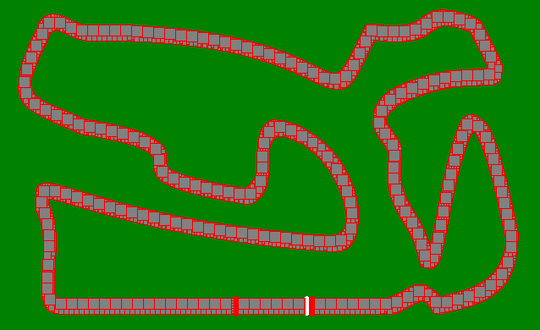
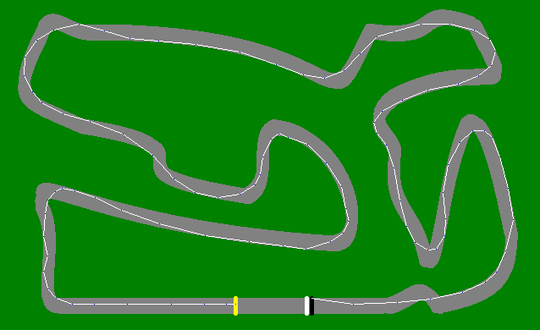
The City - 50 steps
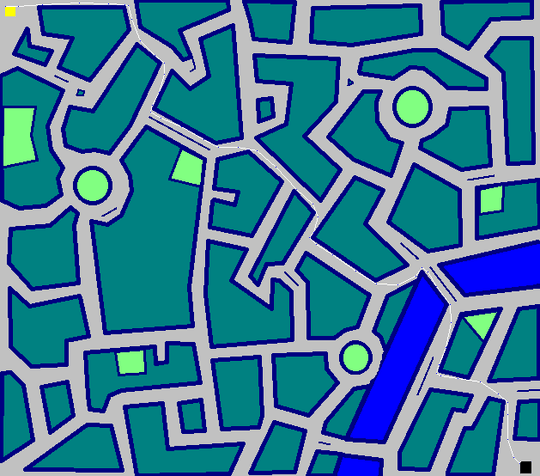
The Gauntlet - 722 steps
Ungolfed:
import System.Environment
import Data.Maybe (fromJust)
import Graphics.GD
import qualified Data.Matrix as M
import qualified Data.List as L
import qualified Data.Set as S
import qualified Data.Map as Map
import qualified Data.PSQueue as PSQ
main = do
trPNG <- loadPngFile =<< fmap head getArgs
(sX, sY) <- imageSize trPNG
px <- mapM (flip getPixel trPNG) [(x,y) | y <- [0..sY-1],x <- [0..sX-1]]
let tr = M.fromList sY sX (map (rgbaToTok . toRGBA) px)
let rt = findRt tr
let vrt = findVRt (head rt) (last rt) (bloat rt tr) tr
let wayPt = map ((\(a,b)->(b-1,a-1)) . fst) vrt
mapM (\(p1,p2) -> drawLine p1 p2 (rgb 255 255 255) trPNG
>> setPixel p1 (rgb 0 0 255) trPNG) (zip wayPt (tail wayPt))
savePngFile "out1.png" trPNG
print $ length vrt - 1
findVRt p1 p2 rt tr = (p1, (0,0)) : fromJust (aStar nghb (\_ _ -> 100)
(\(pos,_) -> fromJust $ Map.lookup pos rt)
((==) p2 . fst) (p1, (0,0)))
where
nghb ((y,x), (vy,vx)) =
S.fromList [(newp, (vy+dy,vx+dx)) |
dy <- [-15 .. 15],
let ady = abs dy,
dx <- [-15+ady .. 15-ady],
not $ dy==0 && dx == 0 && vy == 0 && vx == 0,
let newp = (y+vy+dy,x+vx+dx),
Map.member newp rt,
all ((/=) 1 . (M.!) tr) (bresenham (y,x) newp)]
bloat rt tr = foldr (\(p,h) -> Map.insert p h) Map.empty
(zip (reverse $ f $ f rt) [0..])
where
f = concatMap (n8 tr)
rgbaToTok (r, g, b, _)
| r+g+b == 0 = 3
| r==255 && g==255 && b==0 = 2
| r==g && r==b && 30 <= r && r <= 220 = 0
| otherwise = 1
findRt tr = s : fromJust (aStar nghb cost (const 1) ((==) 3 . (M.!) tr) s)
where
cost (y1,x1) (y2,x2) = if (x1==x2 || y1==y2) then 408 else 577
nghb = S.fromList . n8 tr
s = head [(y,x) | y <- [1..M.nrows tr], x <- [1..M.ncols tr],
M.getElem y x tr == 2]
n8 tr p@(y,x) = filter ((/=) 1 . (M.!) tr) (n8' y x)
where
n8' y x | y==1 || x==1 || y == M.nrows tr || x == M.ncols tr = [p]
| otherwise = [ (y-1,x-1), (y-1,x), (y-1,x+1), (y,x-1),
(y,x+1), (y+1,x-1), (y+1,x), (y+1,x+1) ]
bresenham start@(y0,x0) end@(y1,x1) = walk start (el `div` 2)
where
walk p@(y,x) err
| p == end = [p]
| err-es < 0 = p : walk (y+sdy,x+sdx) (err-es+el)
| otherwise = p : walk (y+pdy,x+pdx) (err-es)
dx = x1-x0; dy = y1-y0;
adx = abs dx; ady = abs dy
sdx = signum dx; sdy = signum dy
(pdx,pdy,es,el) = if adx > ady then (sdx,0,ady,adx) else (0,sdy,adx,ady)
data AStar a c = AStar {
vi :: !(S.Set a), wa :: !(PSQ.PSQ a c), sc :: !(Map.Map a c),
mH :: !(Map.Map a c), ca :: !(Map.Map a a), en :: !(Maybe a) }
deriving Show
aStarInit s = AStar S.empty (PSQ.singleton s 0) (Map.singleton s 0)
Map.empty Map.empty Nothing
aStar graph dist heur goal start =
let s = runAStar graph dist heur goal start
in case en s of
Nothing -> Nothing
Just e -> Just (reverse . takeWhile (not . (== start))
. iterate (ca s Map.!) $ e)
runAStar graph dist heur goal start = aStar' (aStarInit start)
where
aStar' s = case PSQ.minView (wa s) of
Nothing -> s
Just (x PSQ.:-> _, w') ->
if goal x
then s { en = Just x }
else aStar' $ L.foldl' (expand x) (s { wa = w',
vi = S.insert x (vi s)})
(S.toList (graph x S.\\ vi s))
expand x s y =
let vi = sc s Map.! x + dist x y
in case PSQ.lookup y (wa s) of
Nothing -> link x y vi (s { mH
= Map.insert y (heur y) (mH s) })
Just _ -> if vi<sc s Map.! y then link x y vi s else s
link x y v s = s {
ca = Map.insert y x (ca s),
sc = Map.insert y v (sc s),
wa = PSQ.insert y (v + mH s Map.! y) (wa s) }
Golfed:
import System.Environment;import Graphics.GD;import Data.Matrix;import qualified Data.Set as S;import qualified Data.Map as J;import qualified Data.PSQueue as Q
j(Just x)=x;e(y,x)=(x-1,y-1);u=signum;q=J.empty;m=reverse;n=Nothing;z=255;s=abs;t=1<2;f(a,b)(c,d)|b==d||a==c=2|t=3;rT(r,g,b,_)|r+g+b==0=3|r==z&&g==z&&b==0=2|r==g&&r==b&&30<=r&&r<=220=0|t=5
main=do
i:_<-getArgs;t<-loadPngFile i;(a,b)<-imageSize t;p<-mapM(flip getPixel t)[(x,y)|y<-[0..b-1],x<-[0..a-1]];let r=fromList b a$map(rT.toRGBA)p;s:_=[(y,x)|y<-[1..b],x<-[1..a],getElem y x r==2];c p@(y,x)=filter((<5).(!)r)$if y==1||x==1||y==b||x==a then[p]else[(a,b)|a<-[y-1..y+1],b<-[x-1..x+1],a/=y||b/=x];y=s:j(aS(S.fromList.c)f(\_->1)((==3).(!)r)s);l=concatMap c;w=map(e.fst)$fV(head y)(last y)(foldr(\(p,h)->J.insert p h)q$zip(m$l$l y)[0..])r
mapM(\(c,d)->drawLine c d(rgb z z z)t>>setPixel c(rgb 0 0 z)t)$zip w$tail w;savePngFile"o.png"t
fV c d r t=(c,(0,0)):j(aS l(\_ _->99)(\(q,_)->j$J.lookup q r)((==d).fst)(c,(0,0)))where l((y,x),(a,b))=S.fromList[(w,(a+c,b+d))|c<-[-15..15],d<-[s c-15..15-s c],any(/=0)[a,b,c,d],let w=(y+a+c,x+b+d),J.member w r,all((<5).(!)t)$br(y,x)w]
br q@(i,j)r@(k,l)=w q$f`div`2where w p@(y,x)e|p==r=[p]|e-o<0=p:w(y+g,x+h)(e-o+f)|t=p:w(y+m,x+n)(e-o);a=s$l-j;b=s$k-i;h=u$l-j;g=u$k-i;(n,m,o,f)|a>b=(h,0,b,a)|t=(0,g,a,b)
data A a c=A{v::S.Set a,w::Q.PSQ a c,k::J.Map a c,mH::J.Map a c,ca::J.Map a a,en::Maybe a}deriving Show
aS g d h o u=b$en s where b Nothing=n;b(Just e)=Just(m.takeWhile(/=u).iterate(ca s J.!)$e);s=l$A S.empty(Q.singleton u 0)(J.singleton u 0)q q n;i x y v s=s{ca=J.insert y x$ca s,k=J.insert y v$k s,w=Q.insert y(v+mH s J.!y)$w s};l s=b$Q.minView$w s where b Nothing=s;b(Just(x Q.:->_,w'))|o x=s{en=Just x}|t=l$foldl(r x)(s{w=w',v=S.insert x$v s})$S.toList$g x S.\\v s;r x s y=b$Q.lookup y$w s where v=k s J.!x+d x y;b Nothing=i x y v$s{mH=J.insert y(h y)$mH s};b(Just _)|v<k s J.!y=i x y v s|t=s
Tutu siblings -TS#1 - (1764+43 = 2194)
Edit: TS#1 now separate answer.
Edit II: The path for The City is
[(6,7),(21,7),(49,5),(92,3),(126,4),(145,5),(149,6),(153,22),(163,47),(180,64),
(191,73),(191,86),(185,107),(177,122),(175,130),(187,137),(211,147),(237,162),
(254,171),(277,171),(299,175),(321,194),(345,220),(364,237),(370,252),(365,270),
(360,276),(368,284),(387,296),(414,315),(438,330),(463,331),(484,321),(491,311),
(498,316),(508,333),(524,354),(525,375),(519,404),(511,424),(508,434),(513,437),
(533,440),(559,444),(580,458),(591,468),(591,482),(591,511),(598,532),(605,539),
(606,537)]
In The Gauntlet Tutu moves as follows
[(99,143),(114,143),(137,150),(150,161),(149,173),(145,180),(141,197),(138,223),
(135,234),(143,241),(166,248),(186,250),(192,251),(192,261),(192,279),(195,285),
(209,287),(232,284),(248,273),(257,261),(272,256),(279,255),(284,245),(294,243),
(309,231),(330,226),(354,233),(380,253),(400,265),(421,271),(436,268),(438,266),
(440,269),(441,277),(450,278),(470,276),(477,276),(478,285),(481,307),(490,330),
(486,352),(471,370),(449,384),(435,391),(433,401),(446,411),(462,430),(464,450),
(459,477),(454,493),(457,514),(462,522),(472,523),(479,529),(491,531),(493,538),
(496,547),(503,546),(516,545),(519,549),(524,566),(531,575),(531,581),(535,576),
(538,557),(541,523),(545,475),(551,414),(559,342),(565,282),(570,236),(574,204),
(575,184),(574,177),(572,179),(568,174),(568,158),(569,144),(572,143),(578,154),
(585,160),(588,155),(593,140),(598,140),(605,153),(610,156),(611,170),(611,182),
(608,182),(598,175),(594,171),(590,176),(587,195),(589,224),(593,266),(599,321),
(605,376),(609,418),(612,446),(610,465),(615,478),(608,494),(605,521),(611,542),
(618,549),(622,551),(621,563),(611,572),(614,581),(623,581),(630,581),(630,573),
(636,556),(639,551),(642,531),(647,520),(640,511),(637,491),(639,461),(641,416),
(643,356),(647,289),(650,235),(652,195),(647,163),(645,143),(645,136),(653,136),
(670,138),(673,139),(676,155),(679,175),(681,181),(669,188),(662,194),(662,208),
(665,234),(669,274),(674,328),(681,395),(687,457),(692,505),(696,540),(700,560),
(703,566),(706,557),(707,535),(708,498),(711,448),(716,385),(720,325),(723,278),
(726,246),(729,229),(732,227),(733,238),(733,263),(733,303),(733,358),(733,428),
(733,483),(733,523),(732,549),(731,560),(728,558),(726,565),(726,575),(721,575),
(720,586),(720,592),(716,594),(715,608),(715,619),(711,619),(692,619),(658,619),
(609,619),(545,619),(466,619),(372,619),(285,619),(213,619),(155,619),(112,619),
(84,619),(70,618),(70,616),(70,599),(70,567),(70,520),(70,458),(70,381),
(70,300),(70,234),(70,183),(70,147),(70,126),(71,119),(80,119),(104,119),
(143,119),(197,119),(266,119),(350,119),(449,119),(563,119),(681,120),(784,121),
(873,121),(947,121),(1006,121),(1050,121),(1079,121),(1093,121),(1093,122),
(1086,131),(1069,145),(1059,151),(1040,151),(1006,151),(973,150),(955,149),
(950,150),(956,155),(977,160),(994,175),(1003,183),(1003,197),(993,214),
(987,220),(993,223),(1011,223),(1044,223),(1079,229),(1104,240),(1124,242),
(1134,239),(1134,231),(1134,221),(1139,218),(1156,218),(1177,217),(1183,216),
(1191,202),(1208,182),(1231,154),(1249,135),(1259,123),(1264,121),(1264,129),
(1264,152),(1264,190),(1264,243),(1264,311),(1264,393),(1264,460),(1264,512),
(1264,550),(1264,573),(1263,582),(1256,582),(1234,582),(1197,582),(1160,575),
(1132,562),(1118,548),(1113,538),(1107,541),(1099,549),(1102,561),(1113,570),
(1110,578),(1095,583),(1073,581),(1066,579),(1060,566),(1063,559),(1075,554),
(1072,549),(1065,542),(1051,539),(1043,528),(1023,520),(990,511),(970,500),
(953,501),(935,516),(911,534),(899,551),(891,573),(883,580),(867,581),(859,575),
(858,571),(843,566),(830,553),(832,540),(828,527),(819,520),(825,513),(839,506),
(842,495),(843,474),(844,468),(854,468),(877,467),(891,460),(895,452),(901,452),
(906,447),(909,443),(909,441),(915,435),(912,430),(914,429),(908,423),(904,421),
(899,418),(893,417),(879,409),(854,400),(842,390),(842,377),(839,362),(836,362),
(820,360),(812,352),(812,337),(812,307),(814,288),(815,282),(827,280),(834,284),
(850,282),(873,277),(889,280),(891,284),(891,301),(897,320),(903,324),(916,320),
(925,310),(935,314),(953,325),(967,337),(976,345),(981,346),(986,362),(999,378),
(1006,385),(1007,387),(1008,387),(1015,382),(1017,382),(1018,381),(1022,386),
(1021,401),(1008,413),(1009,425),(1014,426),(1031,425),(1038,429),(1047,425),
(1053,429),(1067,426),(1076,425),(1090,427),(1099,424),(1113,426),(1134,427),
(1147,431),(1150,430),(1152,437),(1147,438),(1128,438),(1105,443),(1093,450),
(1089,453),(1085,449),(1075,452),(1064,460),(1055,458),(1052,462),(1049,460),
(1042,464),(1025,463),(1015,463),(1010,470),(1013,471),(1021,472),(1027,476),
(1033,477),(1042,484),(1052,480),(1059,486),(1076,487),(1099,497),(1134,510),
(1169,523),(1191,535),(1205,540),(1210,539),(1210,528),(1210,502),(1210,461),
(1209,409),(1208,372),(1207,349),(1206,341),(1192,335),(1165,327),(1132,310),
(1084,293),(1045,273),(997,256),(961,240),(934,229),(922,218),(919,201),
(917,197),(906,199),(892,212),(876,212),(845,212),(809,212),(781,219),(768,226),
(768,235),(768,259),(768,298),(768,352),(768,421),(769,489),(769,543),(769,582),
(769,606),(769,615),(775,615),(796,615),(832,615),(883,615),(949,615),
(1030,615),(1110,615),(1175,615),(1225,615),(1261,614),(1282,613),(1288,612),
(1296,598),(1296,577),(1296,541),(1296,490),(1296,424),(1296,343),(1296,264),
(1296,200),(1296,151),(1296,116),(1296,96),(1295,90),(1285,90),(1260,90),
(1220,90),(1165,90),(1095,90),(1010,90),(920,90),(844,90),(783,90),(737,90),
(706,90),(690,90),(688,89),(689,86),(681,78),(671,82),(663,90),(648,90),
(618,90),(573,90),(517,90),(476,90),(450,90),(438,89),(439,86),(431,78),
(421,82),(413,90),(398,90),(381,88),(369,78),(357,83),(353,90),(341,90),
(314,90),(297,88),(287,78),(277,82),(269,90),(254,90),(224,90),(179,90),
(123,90),(82,90),(56,90),(43,92),(43,96),(43,115),(43,149),(43,198),(43,262),
(43,341),(43,428),(43,500),(43,557),(43,599),(44,627),(45,640),(49,641),
(67,641),(100,641),(148,641),(211,641),(289,641),(382,641),(490,641),(613,641),
(750,641),(872,641),(979,641),(1071,641),(1148,641),(1212,640),(1261,639),
(1295,638),(1315,636),(1321,633),(1321,621),(1321,594),(1321,552),(1321,495),
(1321,423),(1321,336),(1321,254),(1321,187),(1321,135),(1321,98),(1321,75),
(1320,66),(1313,66),(1291,66),(1254,66),(1207,67),(1175,68),(1157,68),(1154,68),
(1154,75),(1146,75),(1123,75),(1102,74),(1096,73),(1096,69),(1091,66),(1074,66),
(1042,66),(1007,66),(986,65),(980,64),(980,60),(975,57),(958,57),(926,57),
(891,58),(871,59),(866,60),(865,66),(855,66),(830,66),(790,66),(735,66),
(667,66),(614,66),(575,66),(550,65),(540,64),(540,60),(535,57),(518,57),
(489,58),(474,60),(474,62),(472,66),(459,66),(431,66),(388,66),(330,66),
(269,66),(223,66),(191,66),(174,66),(171,65),(168,56),(158,55),(150,61),
(149,66),(138,66),(112,66),(98,63),(95,57),(83,57),(65,59),(61,62),(59,66),
(46,66),(25,67),(18,69),(18,79),(18,104),(18,144),(18,199),(18,269),(18,354),
(18,441),(18,513),(18,570),(18,612),(18,639),(19,652),(26,656),(38,663),
(58,663),(93,663),(143,663),(208,663),(288,663),(383,663),(493,663),(618,663),
(758,663),(884,663),(995,663),(1091,663),(1172,663),(1239,663),(1291,663),
(1328,663),(1350,663),(1358,662),(1361,651),(1376,637),(1378,621),(1374,597),
(1378,574),(1378,541),(1375,519),(1383,501),(1376,483),(1370,478),(1370,464),
(1373,438),(1379,400),(1379,366),(1369,337),(1369,303),(1369,272),(1368,255),
(1382,238),(1381,221),(1371,209),(1375,196),(1380,170),(1374,143),(1367,129),
(1372,112),(1373,85),(1365,64),(1358,57),(1356,41),(1353,39),(1350,41),
(1346,37),(1336,36),(1333,32),(1317,30),(1288,30),(1244,30),(1185,30),(1141,30),
(1102,22),(1057,22),(1026,21),(1005,23),(993,21),(988,25),(975,22),(972,24),
(959,21),(943,24),(937,29),(920,30),(889,30),(843,30),(788,30),(747,30),
(706,39),(664,36),(629,38),(591,34),(559,34),(538,30),(506,30),(465,30),
(431,22),(391,23),(356,22),(328,23),(308,30),(280,30),(237,30),(179,30),
(106,30),(30,28)]

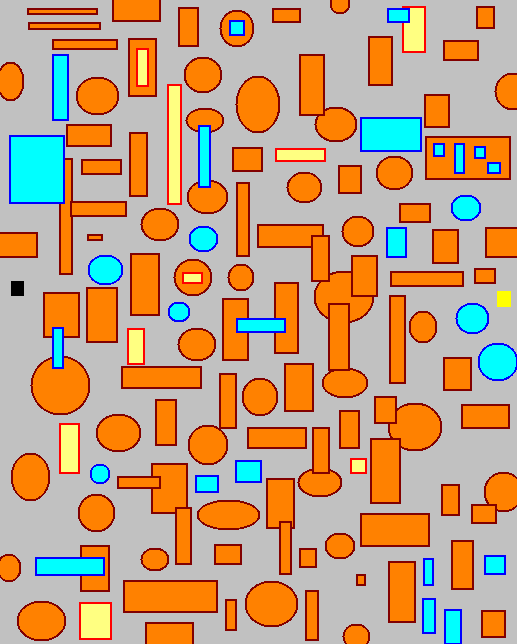
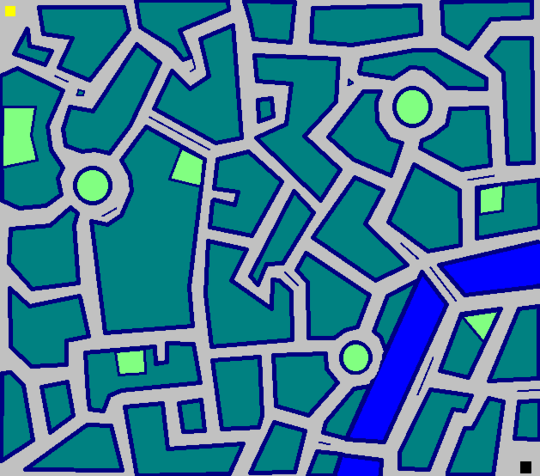
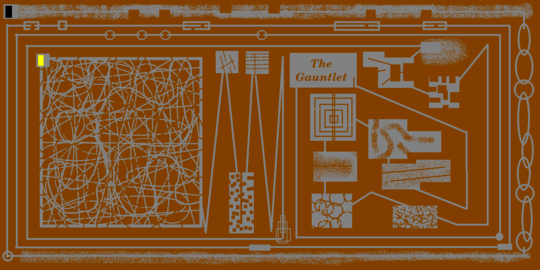
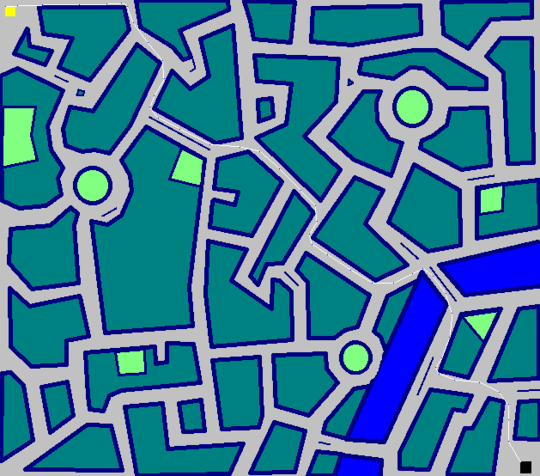
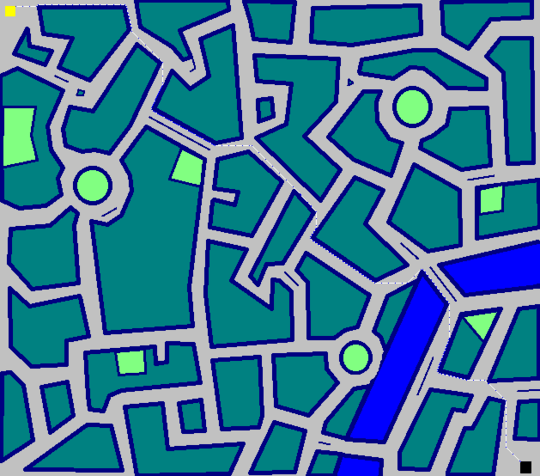


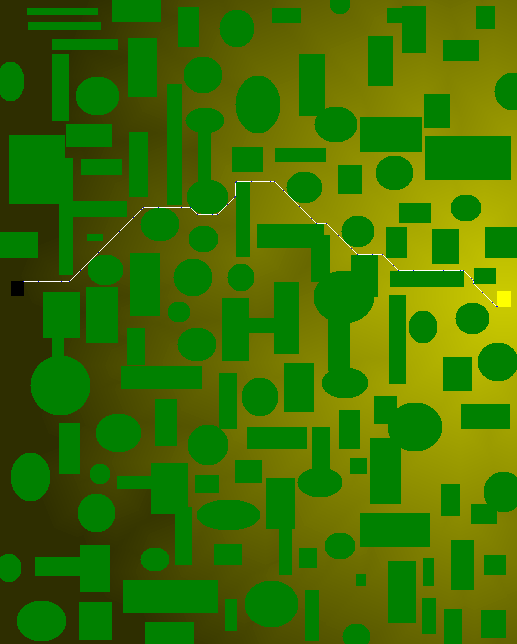
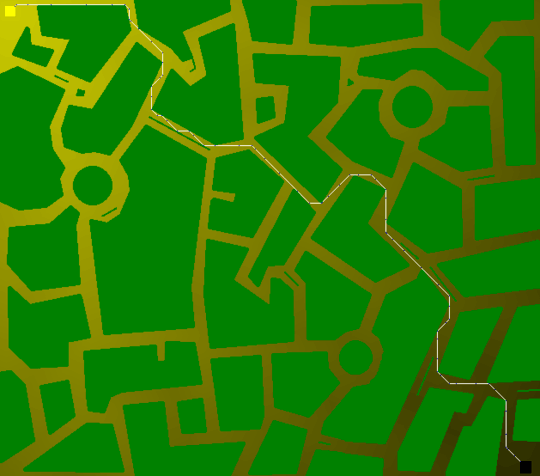
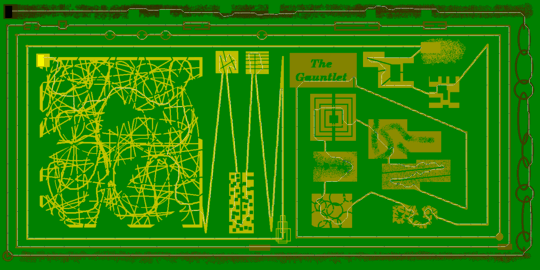


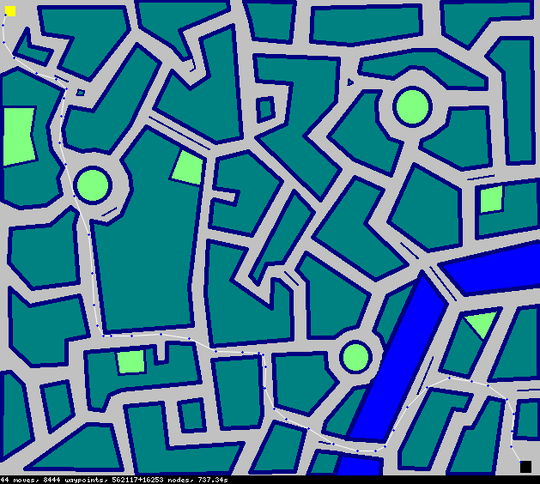
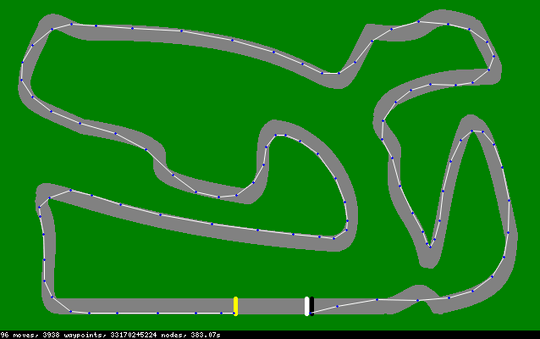
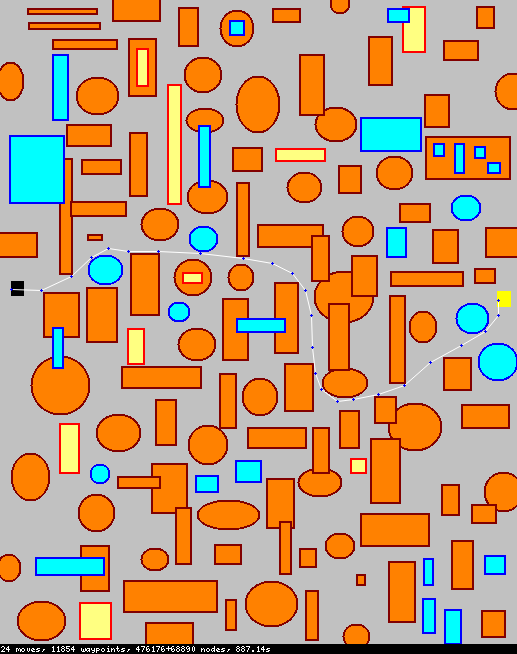
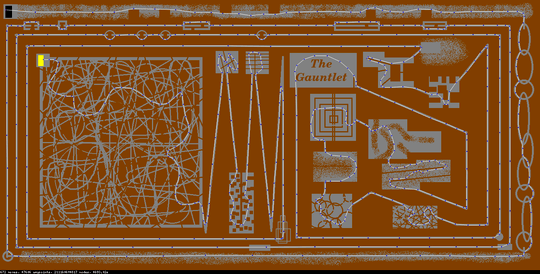 (672 moves if you don't want to zoom)
(672 moves if you don't want to zoom)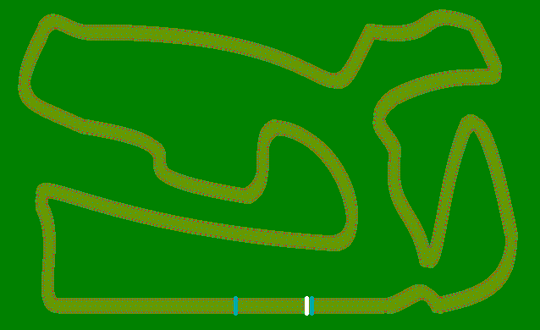
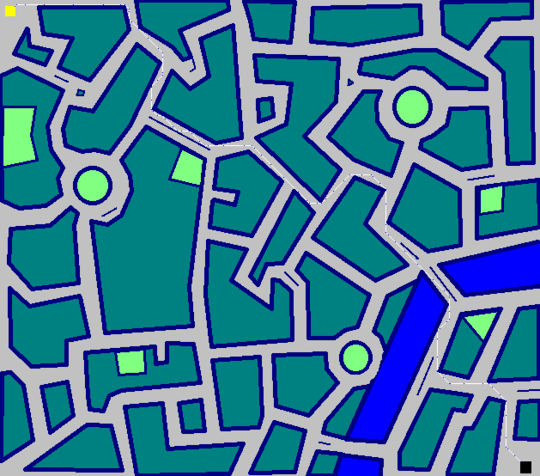
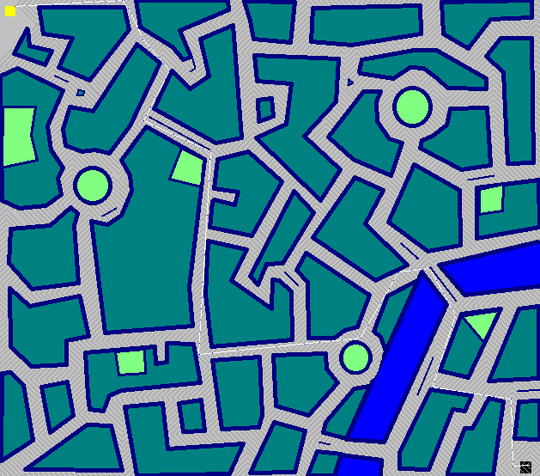
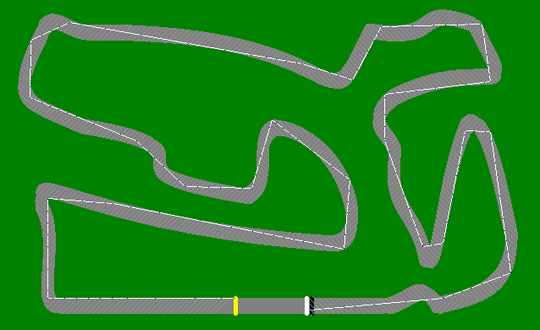
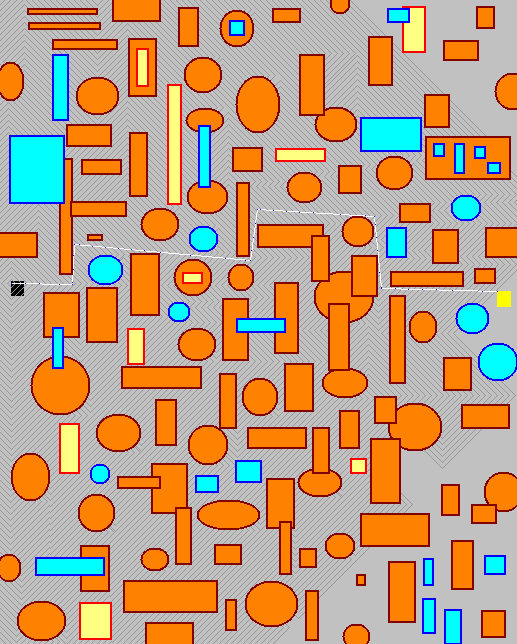
Just an idea: I fear a Gauntlet track solution, given just as a picture file is hard for you to test against your acceleration constraint. I think verifying any NEW-BOUNTY-solution would be easier for you, if you insist on a listing of the path polygon points like so: (x0, y0) (x1, y1) ... (xn, yn). Where n is the turn count found. – Bob Genom – 2015-01-14T20:37:58.457
@Bob. Good idea. It is too hard to judge from the code or the resulting image by eye. I will update the rules. It should not be to hard to update programs to output these points. I will write a small script to verify the points too. – Logic Knight – 2015-01-15T06:37:24.620
I tried a variant based on RRTs. Noncompeting - didn't even try to golf it. Works... interestingly? Works very well on many-branching courses, but doesn't work well at all on anything with a lot of doubling back on itself. (It has to wait until it randomly chooses a point nearer the current leaves than the previous part of the course, not including velocity, before it continues.). On the gauntlet it never even gets out of the starting area :( – TLW – 2016-09-01T22:44:01.690
@xnor duplicate enough but not perfect, I have to add: This question uses graphical input (leading to much larger boards) and allows more acceleration. The BFS implementation would probably time out here. There are also no opponents. – John Dvorak – 2014-12-06T08:02:32.100
This challenge also disallows tunneling through walls. Which brings me to my question: What line drawing algorithm do we have to use? If we have leeway here, how much? I feel like this might be abusible somewhat - especilally if "hand-drawn" "lines" are allowed.
– John Dvorak – 2014-12-06T08:06:14.587I like Martin's challenge but I was aiming for something different here. There is a code-golf aspect which rewards small clever algorithms. There is also graphical output which stimulates competition. I hope this question will be allowed - I am looking forward to some clever answers. – Logic Knight – 2014-12-06T08:07:54.453
@Jan A straight line is required with no anti-aliasing. Built-in line drawing functions are allowed, or you can plot a line using Bresenham's line algorithm. – Logic Knight – 2014-12-06T08:10:52.557
2If the duplicate thing turns out to not be a problem, I think you should add a lot more tracks to judge with. There's many degrees of hard-coding. This makes it hard to draw the line between a program that optimizes for a map with general featured line "roads" and one heavily tailored to this specific map. – xnor – 2014-12-06T08:36:26.550
@MartinBüttner the grey is well-specified. As for yellow, I'd assume #FF0 – John Dvorak – 2014-12-06T10:48:04.640
Good idea. Perhaps make the score equal to code size plus the average of several tracks times 10. I am a lousy pixel artist. Anybody like to come up with an interesting track? – Logic Knight – 2014-12-06T10:55:55.630
@Martin Yes the yellow, black, and white have the usual unique RGB values. Grey is is much more broad and specified in the question. – Logic Knight – 2014-12-06T11:06:29.687
"You must not hard code the optimum path or any of the parameters of the test tracks" Can we tell the program the coordinates of the finish? Otherwise it's a maze exploring problem, which makes the title rather misleading. – Level River St – 2014-12-06T11:12:08.790
@Steve Your program must find a finish location with internal logic after it has read in the track image file, then (I assume) plan a path from a start to the finish. I guess it is a cross between rally driving and orienteering. The path-finding aspect is certainly important, but so is the optimal braking and cornering. – Logic Knight – 2014-12-06T11:18:13.400
I think the global idea is fun, but the golf scoring discourages sophistication. Basically your test track will cost 3000 points to a bot moving at constant speed, and beating that will only reduce the cost to maybe 1500, which can probably not be achieved with 1500 or less extra characters in the source. – None – 2014-12-08T15:14:52.697
@kuroineko, This is a central part of this puzzle. The best scores will be from small clever algorithms. Complex programs (although with a perfect path and car control) will attract a size penalty. You might try (as Bob has done below) a simple but effective program, then improve it and shrink it to be competitive. Of course you could also build a larger clever program and display your great car control, which may attract upvotes. Good results will always get a vote from me. – Logic Knight – 2014-12-08T16:13:33.507
well the challenge is interresting anyway, but still the golf part practically rules out languages like C++ that cannot read png images natively. – None – 2014-12-09T13:38:56.037
1I should have made this obvious in the question, but you can use any third party libraries to read and write images, plot lines and dots etc. You may not use a path-finding library though. Like all golf-like questions, verbose languages (like Java) will suffer, but you can take satisfaction in being the best in your language group. – Logic Knight – 2014-12-09T14:13:51.077