24
11
You all know the Newton method to approximate the roots of a function, don't you? My goal in this task is to introduce you into an interesting aspect of this algorithm.
Newton's algorithm converges only for certain, but most of all complex input values. If you picture the convergence of the method for all input values over the complex plane, you usually get a beautiful fractal like this:
Specifications
The goal of this task is, to generate such fractals. This means, that you get a polynomial as the input and have to print out the corresponding fractal as an image in a format of your choice as the output.
Input
The input is a whitespace-separated list of complex numbers. They are written down in the style <Real part><iImaginary part>, like this number: 5.32i3.05. You may assume, that the input number has no more than 4 decimal places and is smaller than 1000. The first of them must not be zero. For example, this could be an input to your program:
1 -2i7.5 23.0004i-3.8 i12 0 5.1233i0.1
The numbers are interpreted as the coefficients of a polynomial, beginning with the highest power. Throughout the rest of this specification, the input polynomial is called P. The above input is equal to this polynomial:
f(x) = x5 + (-2 + 7.5i)x4 + (23.0004 - 3.8i)x3 + 12ix2 + 5.1233 + 0.1i
The input may come to you either from stdin, from an argument passed to the program or from a prompt displayed to your program. You may assume, that the input does not contain any leading or trailing whitespace characters.
Rendering
You have to render the fractal in the following way:
- Choose as many colors as roots of P plus an extra color for divergence
- For each number in the visible plane, determine whether the method converges and if yes to which root. Color the point according to the result.
- Do not print rulers or other fancy things
- Print a black point at the points, that are the polynomials roots for orientation. You may print up to four pixels around each root.
- Find a way to choose the visible plane in a way, that all roots are distinguishable and spread widely across it, if possible. Although a perfect placement of the output frame is not required, I reserve the right to refuse to accept an answer that chooses the frame in an unacceptable way, eg. always at the same coordinates, all roots are in one point, etc.
- The output image should have a size of 1024*1024 pixels.
- Rendering time is 10 minutes maximum
- Using single precision floating-point values is enough
Output
The output should be a raster graphics image in a file format of your choice, readable by standard software for a brand X operating system. If you want to use a rare format, consider adding a link to a website where one can download a viewer for it.
Output the file to stdout. If your language does not support putting something to stdout or if you find this option less convenient, find another way. In any way, it must be possible to save the generated image.
Restrictions
- No image processing libraries
- No fractal generating libraries
- The shortest code wins
Extensions
If you like this task, you could try to color the points according to the speed of convergence or some other criteria. I'd like to see some interesting results.
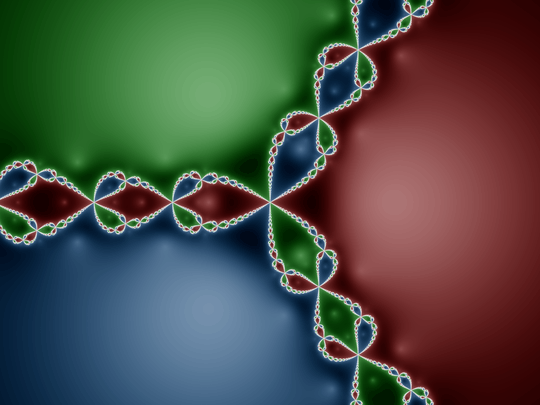 Image from Wikimedia commons
Image from Wikimedia commons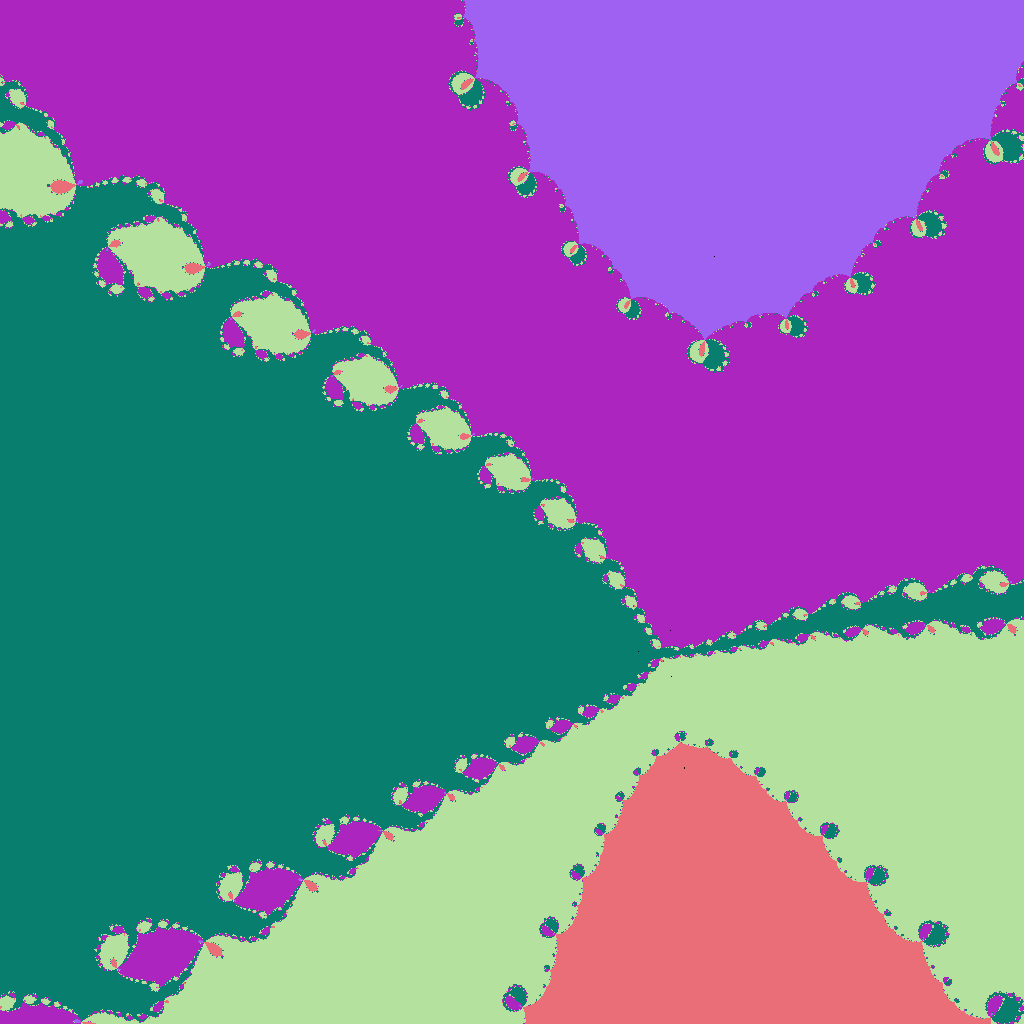
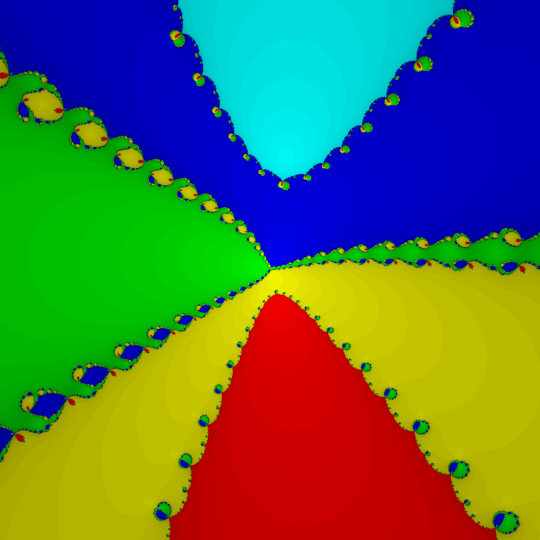
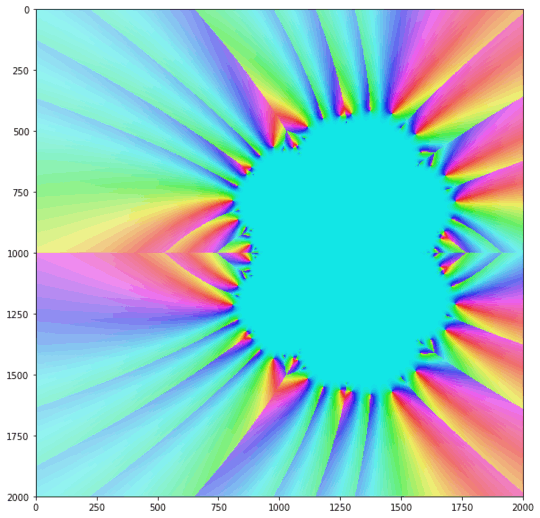
@MartinBüttner Please do not edit parts of the question without knowing why they are the way they are. For instance, I use
<pre>instead of four spaces so no syntax highlighting takes place. Also, the question says<Real part><iImaginary part>for a reason because the right format is1234instead of1234iwhen the imaginary part is missing. – FUZxxl – 2014-12-18T12:08:27.000@MartinBüttner Sorry, I missed one instance where you corrected to polynomial. Of course, polynomial is the correct word in this case. – FUZxxl – 2014-12-18T14:19:01.790
6I'm not entirely sure whether this is suitable as a code golf. In my eyes the task is way too complex. I may be proven wrong, though. – Joey – 2011-05-09T19:37:50.417
@Joey: It IS complex. But I think a solution takes at most 1500 chars. – FUZxxl – 2011-05-09T19:43:05.057
5@Joey: Indeed. I would like for this to be a [tag:code-challenge] myself. – Joey Adams – 2011-05-09T19:50:20.853
I think 1500chars is on the long side for code-golf. I don't like the time limits, as languages which don't use native floats/ints are severly disadvantaged. eg, Python can be almost 2 orders of magnitude slower than C for the same algorithm (especially with math) – gnibbler – 2011-05-09T21:30:08.150
The requirements are impossible to meet in their full generality. E.g. consider input
1 -1000 0.01 -10. It has roots -0.1, 0.1, 1000. If the x-axis is linear and the output image is 1024 pixels then two of the roots cannot be distinguished. – Peter Taylor – 2011-05-09T21:35:51.547graphic format is too much, consider 2D array, or is Processing (processing.org ) or HTML5 canvas allowed? – Ming-Tang – 2011-05-09T22:28:53.363
@SHiNKiROU, .bmp and .tga are pretty trivial file formats. – Peter Taylor – 2011-05-09T22:31:27.370
2... or PPM for that matter. – Joey – 2011-05-09T22:51:55.793
1@Joey: My intention was to create a rather difficult task, as many people dislike very easy tasks. – FUZxxl – 2011-05-10T14:29:41.703
Well, Ventero already told me that this strikes the other end of the scale. It also helps to have at least two different reference solutions (usually golfed) to a task to judge its complexity. But that's probably only my own opinion on that :-). The problem with such complex tasks is that you cannot just throw it away and try a different implementation if it takes you an hour or two to write even the first one. While longer solutions offer more places to golf it also makes iterating on an answer more complex and time-consuming which is why I at least don't like too complex tasks too much. – Joey – 2011-05-10T17:01:22.153
1It's easily broken down into separate tasks, and if your language supports complex floating point numbers natively then you can save a large chunk. I have a not-fully-golfed version running at 1600 chars, of which 340 are the complex number class. It doesn't yet identify the roots and use colours, but I'm trying to track down what I presume is a bug in the N-R code. (Finding a root of x^3-1 starting at -0.5+0.866i surely shouldn't diverge!) – Peter Taylor – 2011-05-10T22:06:01.953
"This task is doable in less than 450 characters in more than 3 languages" [Ev's conjecture (: ] – Eelvex – 2011-05-11T01:01:04.720
Can I cheat by using the svg or even html format? (putting full of divs) – Ming-Tang – 2011-05-11T01:53:51.253
@SHiNKiROU: It says "raster graphics", so no vectors. But you may use SVG and embed a raster image. If you plan something interesting, just show me. – FUZxxl – 2011-05-11T05:16:53.867
so I can't draw full of vector squares as pixels – Ming-Tang – 2011-05-11T05:21:42.687
Ah, that's what you mean. If you like just Do it. You could also submit an SVG with embedded javascript as a submission. – FUZxxl – 2011-05-11T07:56:51.260
"0" and "1" are not of the form
<Real part>i<Imaginary part>. Neither is "i12" unless the brackets mean "optional". – Jesse Millikan – 2011-05-12T01:08:01.3401clarified: EIther part may be dropped if zero, but not both. – FUZxxl – 2011-05-12T07:32:13.663