Generalized Cantor set segment lengths

Question

Problem

Let's define a generalized Cantor set by iteratively deleting some rational length segments from the middle of all intervals that haven't yet been deleted, starting from a single continuous interval.

Given the relative lengths of segments to delete or not, and the number of iterations to do, the problem is to write a program or function that outputs the relative lengths of the segments that have or have not been deleted after n iterations.

Example: Iteratively delete the 4th and 6th eighth

Input:

n – number of iterations, indexed starting from 0 or 1

l – list of segment lengths as positive integers with gcd(l)=1 and odd length, representing the relative lengths of the parts that either stay as they are or get deleted, starting from a segment that doesn't get deleted. Since the list length is odd, the first and last segments never get deleted. For example for the regular Cantor set this would be [1,1,1] for one third that stays, one third that gets deleted and again one third that doesn't.

Output:

Integer list o, gcd(o)=1, of relative segment lengths in the nth iteration when the segments that weren't deleted in the previous iteration are replaced by a scaled down copy of the list l. The first iteration is just [1]. You can use any unambiguous output method, even unary.

Examples

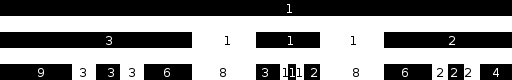
n=0, l=[3,1,1,1,2] →                 [1]
n=1, l=[3,1,1,1,2] →     [3,    1,    1,    1,    2]
n=2, l=[3,1,1,1,2] → [9,3,3,3,6,8,3,1,1,1,2,8,6,2,2,2,4]

n=3, l=[5,2,3]     → [125,50,75,100,75,30,45,200,75,30,45,60,45,18,27]
n=3, l=[1,1,1]     → [1,1,1,3,1,1,1,9,1,1,1,3,1,1,1]

You can assume the input is valid. This is code-golf, so the shortest program measured in bytes wins.

Answer 1

Jelly,  15 13  12 bytes

-2 thanks to Dennis (using a Link rather than a chain allows right to be used implicitly by ¡; No need to wrap the 1 in a list due to the fact that Jelly prints lists of one item the same as the item)
-1 thanks to Erik the Outgolfer (use Ɗ to save the newline from using Ç)

1×€³§JḤ$¦ẎƊ¡

A full program printing a list in Jelly format (so [1] is printed as 1)

Try it online!

How?

1×€³§JḤ$¦ẎƊ¡ - Main link: segmentLengths; iterations
1            - literal 1 (start with a single segment of length 1)
           ¡ - repeat...
             - ...times: implicitly use chain's right argument, iterations
          Ɗ  - ...do: last 3 links as a monad (with 1 then the previous output):
   ³         - (1) program's 3rd argument = segmentLengths
 ×€          -  1  multiply €ach (e.g. [1,2,3] ×€ [1,2,1] = [[1,4,3],[2,4,2],[3,6,3]])
        ¦    -  2  sparse application... 
       $     - (2) ...to: indices: last two links as a monad:
     J       - (2)          range of length = [1,2,3,...,numberOfLists]
      Ḥ      - (2)          double            [2,4,6,...] (note: out-of bounds are ignored by ¦)
    §        - (2) ...of: sum each (i.e. total the now split empty spaces)
         Ẏ   -  3  tighten (e.g. [[1,2,3],4,[5,6,7]] -> [1,2,3,4,5,6,7])
             - implicit print

Answer 2

Python 2, 120 107 104 103 100 99 89 bytes

f=lambda n,l:n and[x*y for i,x in enumerate(l)for y in[f(n-1,l),[sum(l)**~-n]][i%2]]or[1]

Try it online!

---

Saved

• -10 bytes, thanks to Neil

Answer 3

Haskell, 76 58 bytes

l%0=[1]
l%n=do(x,m)<-l%(n-1)`zip`cycle[l,[sum l]];map(*x)m

Try it online!

The function (%) takes the list of line lengths l as first argument and the number of iterations n as second input.

Thanks to Angs and Ørjan Johansen for -18 bytes!

Answer 4

R, 94 bytes

f=function(n,a)"if"(n,unlist(Map(function(g,i)g(i),c(c,sum),split(m<-a%o%f(n-1,a),row(m)))),1)

Try it online!

Answer 5

JavaScript (Firefox 42-57), 80 bytes

f=(n,l,i=0)=>n--?[for(x of l)for(y of(i^=1)?f(n,l):[eval(l.join`+`)**n])x*y]:[1]

Needs those specific versions because it uses both array comprehensions and exponentiation.

Answer 6

JavaScript (Node.js), 71 bytes

l=>f=(n,c=1,e)=>n--?''+l.map(_=>(e=!e)?f(n,c*_):eval(l.join`+`)**n*c):c

Try it online!

Answer 7

Java 10, 261 bytes

L->n->{if(n<1){L.clear();L.add(1);}else if(n>1){var C=new java.util.ArrayList<Integer>(L);for(int l=C.size(),i,x,t,s;n-->1;)for(i=x=0;i<L.size();){t=L.remove(i);if(i%2<1)for(;i%-~l<l;)L.add(i,C.get((i++-x)%l)*t);else{x++;s=0;for(int c:C)s+=c;L.add(i++,t*s);}}}}

Modifies the input-List instead of returning a new one to save bytes.

Try it online.

L->n->{                       // Method with List and integer parameters and no return-type
  if(n<1){                    //  If `n` is 0:
    L.clear();                //   Remove everything from the List
    L.add(1);}                //   And only add a single 1
                              //  Else-if `n` is 1: Leave the List as is
  else if(n>1){               //  Else-if `n` is 2 or larger:
    var C=new java.util.ArrayList<Integer>(L);
                              //   Create a copy of the input-List
    for(int l=C.size(),       //   Set `l` to the size of the input-List
        i,x,t,s;              //   Index and temp integers
        n-->1;)               //   Loop `n-1` times:
      for(i=x=0;              //    Reset `x` to 0
          i<L.size();){       //    Inner loop `i` over the input-List
        t=L.remove(i);        //     Remove the current item, saving its value in `t`
        if(i%2<1)             //     If the current iteration is even:
          for(;i%-~l<l;)      //      Loop over the copy-List
            L.add(i,C.get((i++-x)%l)*t);
                              //       And add the values multiplied by `t`
                              //       at index `i` to the List `L`
        else{                 //     Else (the current iteration is odd):
          x++;                //      Increase `x` by 1
          s=0;for(int c:C)s+=c;
                              //      Calculate the sum of the copy-List
          L.add(i++,t*s);}}}} //      Add this sum multiplied by `t`
                              //      at index `i` to the List `L`

Answer 8

Jelly, 13 bytes

Ø1××S¥ƭ€³Ẏ$¡Ṗ

Try it online!

Full program. Outputs 1 instead of [1]. Annoyingly, ḋ doesn't work like ×S¥ in this context, and ƭ doesn't work well with nilads. >_<

Answer 9

APL (Dyalog Classic), 20 bytes

{(∊⊢×⍵(+/⍵)⍴⍨≢)⍣⍺,1}

Try it online!

Answer 10

K (ngn/k), 27 bytes

{x{,/y*(#y)#x}[(y;+/y)]/,1}

Try it online!

{ } is a function with arguments x and y

(y;+/y) a pair of y and its sum

{ }[(y;+/y)] projection (aka currying or partial application) of a dyadic function with one argument. x will be (y;+/y) and y will be the argument when applied.

,1 singleton list containing 1

x{ }[ ]/ apply the projection x times

(#y)#x reshape to the length of the current result, i.e. alternate between the outer y and its sum

y* multiply each element of the above with the corresponding element of the current result

,/ concatenate

Answer 11

Ruby, 73 bytes

->a,l{r=[1];a.times{s=p;r=r.flat_map{|x|(s=!s)?l.map{|y|x*y}:x*l.sum}};r}

Try it online!

Answer 12

Pyth, 20 bytes

us.e?%k2*bsQ*LbQGE]1

Input is segment array l, then iterations n. Try it online here, or verify all the test cases at once here.

us.e?%k2*bsQ*LbQGE]1   Implicit, Q=1st arg (segment array), E=2nd arg (iterations)
u                E     Execute E times, with current value G...
                  ]1   ... and initial value [1]:
  .e            G        Map G, with element b and index k:
        *bsQ               Multiply b and the sum of Q {A}
            *LbQ           Multiply each value of Q by b {B}
    ?%k2                   If k is odd, yield {A}, otherwise yield {B}
 s                       Flatten the resulting nested array